The weak and strong ends of a theory

Joseph  Minahan

Viewpoint

The weak and strong ends of a theory

Department of Physics and Astronomy, Uppsala University, 751 08 Uppsala, Sweden

September 21, 2009• Physics 2, 79

A mathematical formulism makes a step forward in proving the AdS/CFT correspondence that connects quantum mechanics with gravity.

Figure caption — **Figure 1:** An important calculation in gauge theory can be mapped to one of the most fundamental toy models in the study of magnetism: the Heisenberg spin chain.

In 1997, Juan Maldacena made the startling conjecture that theories of gravity on curved spaces are equivalent to ordinary quantum field theories confined to the boundaries of those spaces. The most concrete example of this correspondence is for a string theory living on a ten-dimensional spacetime called ${AdS}_{5} \times S_{5}$ [1], which is conjectured to be equivalent to a special form of Yang-Mills gauge theory living on the boundary of the five-dimensional ${AdS}_{5}$ space (called anti-de Sitter space). (The boundary in the case of ${AdS}_{5}$ is a four-dimensional spacetime.) In the 1970s, Yang-Mills theories were developed as an extension of field theories like quantum electrodynamics (QED) to the case of strong interactions in quantum chromodyamics (QCD), while anti-de Sitter spaces are solutions to Einstein gravity equations with a negative cosmological constant that gives a constant curvature of space.

The gauge theory that appears in Maldacena’s conjecture is a highly symmetric version called $N$ = 4 super Yang-Mills (SYM). $N$ = 4 SYM has the maximal amount of supersymmetry—the symmetry that pairs up bosons and fermions. It is also an exact conformal field theory (CFT), meaning that the physics is the same under any rescaling of energies or length scales. (This is a property not shared by the strong interactions, which come with a natural energy scale of about 200 MeV, but $N$ = 4 SYM still provides a useful theory for understanding several aspects of the strong interaction.) If the correspondence, called the AdS/CFT correspondence, is correct then we will have successfully merged gravity with quantum mechanics, at least in this particular background spacetime, since the $N$ = 4 SYM on the boundary is believed to be a consistent theory.

The AdS/CFT correspondence is widely accepted as true but remains unproven, even in certain limits where calculations become vastly simplified. The difficulty in proving AdS/CFT is that it works as a strong/weak duality. That is, if particles in the gauge theory are strongly coupled, implying that the quantum corrections to the theory are large, then the curvature of ${AdS}_{5} \times S_{5}$ is small. This leads to a weak coupling between the different vibrational modes in the string theory, and vice versa. Thus one must be able to make computations in the strong coupling regime for at least one of the theories (either AdS gravity or quantum field theory) in order to directly compare results between them. In a paper appearing in Physical Review Letters [2], Nikolay Gromov, Vladimir Kazakov, and Pedro Vieira (GKV), at DESY in Hamburg, Germany, École Normale Supérieure, in Paris, France, and the Max-Planck-Institut in Potsdam, Germany, respectively, make important progress in this direction by formulating a set of functional equations, called a $Y$ -system (see Appendix for greater detail), for the gauge theory that are true for any value of the coupling—strong or weak. These equations open up the possibility of directly computing a whole class of physical quantities in the gauge theory and comparing them with string theory predictions.

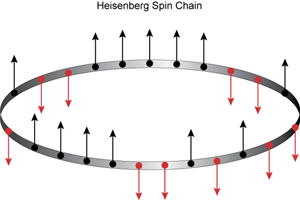
The key idea behind the GKV results is integrability. An integrable model is a many-body system that can effectively be reduced to a combination of two-body systems, rendering the theory solvable. This concept is perhaps best explained by a simple and familiar example: the Heisenberg ferromagnet in one dimension (Fig. 1). In this model, $L$ spin-1/2 magnetic dipoles lie on a circular chain. Each dipole is assumed to only interact with the dipole closest to it on either side. The Hamiltonian for this system is proportional to a coupling constant, $λ$ , times a sum over the magnetic interactions between nearest neighbor spins.

The ground state has all of the spins aligned (say, up). One constructs excited states—called “magnons”— by adding down-pointing spins on the chain. The individual magnons are like particles, with a momentum $p_{i}$ and a known dispersion relation for the energy, $ε (p_{i})$ . The scattering of the magnons off of one another is encoded in a momentum-dependent phase, called the $S$ matrix, that appears in the wave function for a two-magnon state. Since the wave function must satisfy the condition that $x \equiv x + L$ , the momenta of two scattering magnons are quantized. It turns out that for scattering processes that involve more than two magnons, the quantization conditions still only depend on the two-magnon $S$ matrices (and thus the system is integrable). The relationship between the magnon momenta and the $S$ matrices leads to what are known as the Bethe equations and we can solve them to find the allowed sets of $p_{i}$ . The energy of the states is the total of the magnon energies $E = Σ_{j} ε (p_{j})$ .

What does this all have to do with the gauge theory? It turns out that even though they describe very different physical systems, we can map useful computations in the gauge theory to an integrable problem analogous to that of the Heisenberg magnet. To see this, consider that the physical information of a conformal field theory is contained in correlation functions of its composite operators (or, observables). These operators are products of the elementary fields that constitute the gauge theory. A correlation function tells us how the quantum fluctuations of one operator are correlated with the fluctuations of one or more other operators as a function of their separation in space and time. Since the theory is conformal, the only scales in the problem are the separations between the operators. Specifically, under a rescaling of all lengths by a factor of $β$ , an operator with a well-defined scaling dimension $Δ$ will be multiplied by a factor of $β^{- Δ}$ .

There is a particular class of composite operators, called “single trace operators,” that are invariant under gauge transformations, a property all physical operators must satisfy. A single trace operator can be built up by taking the trace of a product of three complex scalar fields: $Z (x)$ , $W (x)$ , and $Y (x)$ , along with their conjugates. (All fields in $N$ = 4 SYM are like the gluons of the strong interaction: they come with a color and an anticolor at the same time. Although the strong interaction has only three colors, in $N$ = 4 SYM we can consider an arbitrary number $N$ of colors and the fields are expressed as $N \times N$ matrices, with the row index labeling the color and the column index labeling the anticolor.) Let us consider single trace operators containing only two types of scalar fields, say $Z$ and $W$ . A typical operator made up of $L$ such scalars would be given by $tr$ ( $Z Z W$ … $W Z W$ … $Z Z$ ) with the $Z$ and $W$ fields arranged in some particular order inside the trace (note that $L$ here is simply the number of operators, and not a physical length as in the Heisenberg spin chain). A free scalar field has scaling dimension one in four spacetime dimensions so the scaling dimension $Δ$ for our operator is $L$ when the coupling, that is, strength of the interactions between the particles of the theory, is turned off.

However, the interactions of the gauge theory shift the scaling dimension to $Δ = L + Γ$ , where $Γ$ is called the anomalous matrix and can be computed perturbatively in the coupling $g$ . For certain limits, it turns out that $Γ$ is equivalent to the Hamiltonian for the Heisenberg chain magnet, with $λ = g^{2} N$ and in place of the magnetic exchange, one considers the interaction between the fields at the $ℓ$ and $ℓ + 1$ positions in the trace. Therefore the first-order correction (one loop) to the anomalous dimension matrix for this class of operators is the Heisenberg Hamiltonian with the $Z$ and $W$ fields as the up and down spins!

The one-loop anomalous dimensions are then found by solving the Heisenberg Bethe equations. If we expand the class of operators to include all types of fields, then the anomalous dimension matrix is still equivalent to an integrable Hamiltonian and the Bethe equations are enlarged to include a more general set of magnons [3].

Higher loop contributions to the anomalous dimension can also be determined in the limit that $L$ is infinite, but the finite size corrections were a missing piece of the puzzle [4]. Although for weak coupling $(λ < < 1)$ , $Γ$ is well approximated by a local spin chain Hamiltonian, for $λ \sim 1$ the Hamiltonian becomes long range. In this limit, finite size effects become important [5,6]. The GKV paper, which successfully treats both the weak and strong coupling limits, incorporates previous work on finite size systems to the particular problem of finite $L$ operators in $N = 4$ SYM (see Appendix for details). What is remarkable is that they can calculate the anomalous dimension out to four orders of perturbation theory in a highly compact form. By comparison, in a typical field theory calculation, this same computation could only be done with hundreds of Feynman diagrams. String theory predicts that the anomalous dimension should scale as $Γ \sim 2 λ^{1 / 4}$ , when $λ > > 1$ [7]. Recent numerical results using the GKV $Y$ -system show excellent agreement with this prediction. The GKV $Y$ -system promises to have other applications as well, especially within the intermediate region, $λ \sim 1$ , where neither perturbation theory nor string theory are particularly useful.

Even after 35 years of research on QCD, there are still some features of gauge theories where we only have a limited understanding. Any new results that can help improve our intuition about these theories are clearly significant. In this respect, the substantial effort by GKV towards confirming Maldacena’s conjecture is a major step forward.

Appendix

Underlying the GKV construction is what is called the thermodynamic Bethe ansatz (TBA). Al. Zamolodchikov [8] observed that by switching the spatial and temporal directions, the Euclidean path integral of a relativistic 1+1 dimensional theory with the spatial direction compactified on a circle of circumference $L$ has the same path integral as the uncompactified theory at temperature $T = 1 / L$ . Hence the ground-state energy $E (L)$ of the former equals the free energy $F (1 / L)$ of the latter. $F (1 / L)$ is derived using the Bethe equations, which are valid because now the spatial dimension is infinite. The theory can have various species of particles where each particle type has a dispersion relation $ϵ_{a} (θ) = \sqrt{p_{a}^{2} + m_{a}^{2}}$ , or, in terms of a rapidity variable $θ$ , $ϵ_{a} (θ) = m_{a} cosh θ$ , $p_{a} (θ) = m_{a} sinh θ$ . Zamolodchikov showed that the ground-state energy $E (L)$ is equal to the integral over $θ$ of a simple expression involving $p_{a} (θ)$ and $Y_{a} (θ) \equiv e^{- ε_{a} (θ) L}$ , where the “pseudo-energies” $ε_{a} (θ)$ equal the usual energies $ϵ_{a} (θ)$ at infinite $L$ . Subsequently, it was shown how to extract the energy of the exited states from this integral [9]. At finite $L$ , the pseudo-energies satisfy a series of consistency conditions that depend on the particle S matrices. These conditions reduce to a set of functional equations for the $Y_{a} (θ)$ called the $Y$ -system, and their form depends on the symmetries of the theory.

The interchange of the spatial and temporal directions in the TBA replaces $(p, ϵ)$ by $(i ϵ, i p)$ . For a relativistic theory the dispersion relation is invariant under this transformation. But the magnon dispersion relation found in $N = 4$ SYM is nonrelativistic, so, instead, the TBA procedure sets the ground-state energy $E (L)$ equal to the free energy $F^{*} (1 / L)$ of a “mirror theory” [10,11], where the mirror has $(p, ϵ)$ given by $(i ϵ^{*}, i p^{*})$ . $ϵ^{*} (p^{*})$ is the dispersion relation of the original theory, where the $*$ indicates that the quantities are evaluated in the mirror’s physical region where $i ϵ^{*}$ and $i p^{*}$ are real. Gromov, Kazakov, and Vieira, following the important work in Ref. [12], find the integral for $E (L)$ over a rapidity variable $u$ , where now the integral involves a set $Y_{n, 0} (u)$ for the magnons in the mirror theory $(n = 1)$ , and their bound states $(n > 1)$ . GKV then postulate that the $Y_{n, 0} (u)$ are part of an integrable $Y$ -system, $Y_{a, s} (u)$ , where $a$ and $s$ are integers ( $a$ nonnegative). Definining a new quantity $T_{a, s}$ , where $Y_{a, s} = \frac{T_{a, s + 1} T_{a, s - 1}}{T_{a + 1, s} T_{a - 1, s}}$ , the GKV $Y$ -system can be written as a series of so-called Hirota equations: $T_{a, s}^{+} T_{a, s}^{-} = T_{a + 1, s} T_{a - 1, s} + T_{a, s + 1} T_{a, s - 1}$ , with $T_{a, s}^{\pm} = T_{a, s} (u \pm \frac{i}{2})$ .

To solve these equations one must impose some boundary conditions. First, the symmetry group structure of the magnons sets $Y_{a, s} (u) = 0$ if both $a > 2, | s | > 2$ . Second, there are transformations on the $T_{a, s}$ that leave $Y_{a, s}$ fixed, so one is free to set $T_{0, s} = 1$ . Third, one can take the large $L$ limit and use the Bethe equations to determine the asymptotic form of the $Y_{n, 0}$ . In this limit the $Y_{n, 0}$ are exponentially suppressed, decoupling the $T_{n, s}$ into two independent halves where the exact expressions are known [13]. This information is sufficient to generate the anomalous dimensions order by order in $λ$ using the Hirota equations.

Recently, GKV used the $Y$ -system to generate numerically the anomalous dimension for the shortest nontrivial operator, which has $L = 4$ , from small to very high values of $λ$ [14].

References

J. M. Maldacena, Adv. Theor. Math. Phys. 2, 231 (1998); Int. J. Theor. Phys. 38, 1113 (1999); arXiv:hep-th/9711200
N. Gromov, V. Kazakov, and P. Vieira, Phys. Rev. Lett. 103, 131601 (2009); arXiv:0901.3753
J. A. Minahan and K. Zarembo, JHEP 03, 013 (2003); arXiv:hep-th/0212208; N. Beisert and M. Staudacher, Nucl. Phys. B 670, 439 (2003); arXiv:hep-th/0307042
N. Beisert and M. Staudacher, Nucl. Phys. B 727, 1 (2005); arXiv:hep-th/0504190; N. Beisert, Adv. Theor. Math. Phys. 12, 945 (2008); arXiv:hep-th/0511082; R. A. Janik, Phys. Rev. D 73, 086006 (2006); arXiv:hep-th/0603038; N. Beisert, B. Eden, and M. Staudacher, J. Stat. Mech. 01, P021 (2007); arXiv:hep-th/0610251
D. Serban and M. Staudacher, JHEP 06, 001 (2004); arXiv:hep-th/0401057
J. Ambjorn, R. A. Janik, and C. Kristjansen, Nucl. Phys. B 736, 288 (2006); arXiv:hep-th/0510171
S. S. Gubser, I. R. Klebanov, and A. M. Polyakov, Phys. Lett. B 428, 105 (1998); arXiv:hep-th/9802109
Al. B. Zamolodchikov, Phys. Lett. B 253, 391 (1991)
P. Dorey and R. Tateo, Nucl. Phys. B 482, 639 (1996); arXiv:hep-th/9607167
J. Ambjorn, R. A. Janik, and C. Kristjansen, Nucl. Phys. B 736, 288 (2006); arXiv:hep-th/0510171
G. Arutyunov and S. Frolov, JHEP 12, 024 (2007); arXiv:0710.1568
Z. Bajnok and R. A. Janik, Nucl. Phys. B 807, 625 (2009); arXiv:0807.0399
V. Kazakov, A. S. Sorin, and A. Zabrodin, Nucl. Phys. B 790, 345 (2008); arXiv:hep-th/0703147
N. Gromov, V. Kazakov, and P. Vieira, arXiv:0906.4240

About the Author

Joseph Minahan received his Ph.D. from Princeton University in 1987. He is a professor at Uppsala University in Sweden where he has been since 2000. His research is centered on string theory and integrable systems.