Electrons Take Their Places on a Liquid Helium Grid

Kimitoshi Kono

Viewpoint

Electrons Take Their Places on a Liquid Helium Grid

Low Temperature Physics Laboratory, RIKEN, Hirosawa 2-1, Wako-shi, 351-0198, Japan

December 19, 2011• Physics 4, 110

A device that efficiently transfers single electrons from one grid point to another on the surface of liquid helium could provide a scalable way to make arrays of electron qubits.

Figure caption — **Figure 1:** This scanning electron micrograph shows an electrostatic “split” gate that controls the passage of a single electron on a liquid helium surface from one electron reservoir to another. Liquid helium is condensed on the flat bed and electrons flow along the top surface of the condensed helium. (Inset: A close-up of the split gate.) Bradbury *et al.* use an array of gates to detect and control the transport of single electrons on a liquid helium surface operated under the same principle.

Electrostatic potentials never have a minimum or maximum in vacuum, which is why trapping charged particles at a specific point in space requires an elaborate mechanism. (The 1989 Nobel prize was awarded for such efforts, in recognition of the development of ion trapping techniques [1].) Now, Forrest Bradbury, at Princeton University, and colleagues have used one of the few systems where charges can be localized—electrons trapped on the surface of liquid helium ( $He$ )—to both transport a large number of single electrons and detect them one by one. Their device, which they describe in Physical Review Letters [2], offers a potentially scalable way to make an array of quantum bits (qubits) from individual electrons.

Liquid helium is actually a very sparse medium. The radius of a helium atom is less than $30$ picometers (pm) and it has a large zero-point motion, so the mean distance between atoms in liquid helium- $4$ is $360 pm$ , and slightly larger in liquid helium- $3$ . This results in ample void space within which electrons could potentially move. However, since the two electrons that occupy the $1 s$ orbit of a helium atom already form a closed shell, the Pauli exclusion principle forces the wave function of an additional (free) electron to vanish at the position of each helium atom. The kinetic energy of an electron in the void space would therefore be about $1$ electron volt (eV), making it impossible for low-energy electrons to penetrate into liquid helium.

On the other hand, an electron above a liquid helium surface induces a polarization in the liquid and experiences a weak attractive force. The two forces—the attraction to the surface and the repulsive force within the liquid—act on the electron to form a potential well at the surface. The motion perpendicular to the surface of these so-called “surface state electrons” is quantized to form discrete energy levels, and the binding energy of the ground state amounts to $1 meV$ . Correspondingly, the electronic wave functions have their maximum amplitudes at around $10$ nanometers from the surface. In the direction parallel to the surface, electron motion is free, except that electrons can scatter from both helium vapor gas atoms (a weak effect at low temperatures) or surface capillary waves, called “ripplons.” Electrons in this two-dimensional system—the coldest pure electron system known to form—have a very high mobility, more than $10^{4} m^{2} /$ (volt second)—some $2$ orders of magnitude greater than the mobility of graphene. The system is very clean and simple, and lends itself to a transparent theoretical description.

Thermionic emission, photo emission, or glow discharge are all methods for populating the surface of bulk liquid helium with electrons (a positively biased plate electrode immersed underneath the liquid and parallel to the surface provides the necessary storing force). The electron density is limited to less than $2 \times 10^{9} / {cm}^{2}$ , above which a surface instability develops. At this density, the electronic surface states on liquid helium form a nondegenerate two-dimensional (2D) electron system. One of the most prominent properties observed in this system is the formation of the 2D Wigner solid, which is a pure electron crystal [3].

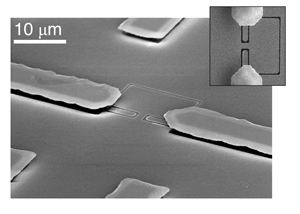
Though the Wigner crystal of electrons is interesting in its own right, a recent focus of this field is to explore ways to control single electrons on the surface of liquid helium. In particular, theorists proposed these surface electrons could function as qubits, but first, techniques to manipulate and control the electrons individually had to be developed [4]. In 2001, a group working at the University of London demonstrated they could manipulate a strip of electrons forming a Wigner crystal on liquid helium by condensing the liquid into a micron-sized capillary and using pre-patterned electronic gates to pull the charges along the capillary [5] (see 18 October 2001 Focus story). By employing such a capillary-condensed helium channel (see Fig. 1), experimentalists were able to measure the conductivity of a quasi-one-dimensional electron wire [6], count the number of electrons (down to the last one) in a pool on the channel [7], and observe single-lane electron transport through a narrow constriction [8] (see 21 January 2011 Focus story).

Building on this work, Bradbury et al. use electrostatic gates to move electrons around the surface of helium condensed within a set of $120$ parallel running capillaries, each $3$ micrometers ( $μ m$ ) wide. Gate electrodes, which are also $3 μ m$ wide, run under the capillaries in the perpendicular direction. The device acts as a charge-coupled device, in that the grid of capillaries and gates functions as an array of pixels. Some gates are dedicated to charge sensing, and are sensitive enough to register a single electronic charge per capillary. Bradbury et al. also devised a way to reliably move the electrons around the grid. They bundle the gates into groups of three, by applying the same potential to each group, to form one pixel. One of the gates in the group stores the charge, while the other two can move the charge to a group of gates to the left or to the right. To move the charge off one group, the team applies an appropriate pulse sequence to the group and its neighbors—a process called “clocking.” The performance of this device is so efficient that the loss of charge is $10, 000$ times smaller than in conventional semiconductor-based devices that perform the same operation—equivalent to an efficiency of $99.99999999 %$ (“ten nines”). Moreover, the clocking is able to transfer a single electron per pixel. It is a real breakthrough in single-electron manipulation on liquid helium, and will open a new possibility to efficiently transfer quantum information between qubits.

There are several ways qubits could be constructed from such a system. One way would be to use two discrete energy levels associated with the electronic surface states to define a two-level system. These levels could be influenced by the existence of a neighboring electron to produce a Conditional NOT (CNOT) operation [4]. An alternative is to take advantage of the lateral confinement of electrons on the surface of helium, which results in quantized orbital motion similar to what is observed in semiconductor quantum dots. Harnessing the spin of electrons on liquid helium to form a qubit is a compelling option. Since liquid helium provides an extremely pure nonmagnetic environment for electrons, such qubits would likely have a long spin decoherence time [9].

References

http://www.nobelprize.org/nobel_prizes/physics/laureates/1989/.
F. R. Bradbury, M. Takita, T. M. Gurrieri, K. J. Wilkel, K. Eng, M. S. Carroll, and S. A. Lyon, Phys. Rev. Lett. 107, 266803 (2011)
C. C. Grimes and G. Adams, Phys. Rev. Lett. 42, 795 (1979)
P. M. Platzman and M. I. Dykman, Science 284, 1967 (1999)
P. Glasson, V. Dotsenko, P. Fozooni, M. J. Lea, W. Bailey, G. Papageorgiou, S. E. Andresen, and A. Kristensen, Phys. Rev. Lett. 87, 176802 (2001)
H. Ikegami, H. Akimoto, and K. Kono, Phys. Rev. Lett. 102, 046807 (2009)
E. Rousseau, D. Ponarin, L. Hristakos, O. Avenel, E. Varoquaux, and Y. Mukharsky, Phys. Rev. B 79, 045406 (2009)
D. G. Rees, I. Kuroda1, C. A. Marrache-Kikuchi, M. Höfer, P. Leiderer, and K. Kono, Phys. Rev. Lett. 106, 026803 (2011)
S. A. Lyon, Phys. Rev. A 74, 052338 (2006)

About the Author

Kimitoshi Kono is the Chief Scientist of the Low Temperature Physics Laboratory, RIKEN. He received his Doctor of Science degree from the Department of Physics, University of Tokyo in 1982. He was an associate professor at the Hyogo University of Teacher Education, Tsukuba University, and the ISSP at the University of Tokyo before moving to RIKEN in 2000. He was a Humboldt Reseach Fellow at the University of Mainz (1988–1989) and at the University of Konstanz (1991). An experimentalist, his research interests include the properties of superfluid helium- $3$ , strong correlation effects in single-electron transport on the surface of liquid helium, electron qubits on liquid He, the quantum transport associated with spins and supersolidity of helium- $4$ .