Microwaving and stirring the quantum Hall spaghetti

Michael M. Fogler

Viewpoint

Microwaving and stirring the quantum Hall spaghetti

Michael M. Fogler

Department of Physics, University of California, San Diego, 9500 Gilman Drive, La Jolla, CA 92093, USA

May 16, 2011• Physics 4, 39

An ingenious experiment has probed the collective response of the quantum Hall stripe phase.

Figure caption — **Figure 1:** Quantum Hall stripes (dark wavy lines) are perturbed by surface acoustic waves propagating with momentum $q$ (bottom arrow). A microwave field of frequency $ω$ (red arrows) excites magnetophonons. Photoluminescence (vertical wavy lines) signals how much energy is absorbed as a function of $ω$ and $q$ .

Two-dimensional (2D) electron materials such as semiconductor quantum wells and graphene form exotic phases when placed in a strong magnetic field and cooled down to low temperatures. The energy spectrum of such systems consists of macroscopically degenerate energy levels (Landau levels) that lead to the rich physics of integral and fractional quantum Hall effects (IQHE and FQHE). Different states of 2D electron matter appear when the degeneracy of the topmost partially filled Landau level is lifted by interactions among electrons.

In an article in Physical Review Letters, Igor Kukushkin at the Institute for Solid State Physics in Chernogolovka, Russia, and coauthors in Stuttgart and Israel present the results of an experiment [1] that probes a particularly intriguing quantum Hall state—the stripe phase—that is believed to form when the topmost Landau level is nearly half filled. The stripe phase can be thought of as a periodic one-dimensional array of linear domains in which the topmost Landau level is either completely empty or completely full. The electron density is periodically modulated as well; hence the stripe phase is a type of charge-density wave.

The stripe phase was predicted $15$ years ago [2], and its existence was soon confirmed by transport measurements [3,4]. It has attracted much interest [5] because it is different from other quantum Hall phases and also because similar structures have been discovered more or less simultaneously in other strongly correlated systems such as high- $T_{c}$ superconducting cuprates and manganites. The stripe phase and its close relative the bubble phase—a triangular crystal of circular domains—are also ubiquitous in other fields of physics, chemistry, and biology. Examples include magnetic films, pattern-formation systems, “conventional” charge-density waves, nuclear matter, and liquid crystals. However, this author’s favorite analogy to the stripe phase is the quantum Hall spaghetti. The image of spaghetti emerges if one realizes that the stripes are quite flexible and so are not expected to be perfectly straight. Disorder and thermal and quantum fluctuations should introduce distortions in the ideal stripe pattern, making them somewhat twisted and randomized, like spaghetti on a plate.

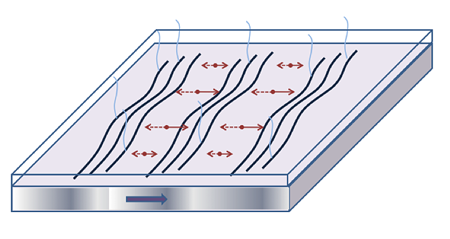
In their experiment, Kukushkin et al. investigated the stripe phase in a state-of-the-art $GaAs$ quantum well structure with a technique combining surface acoustic waves (SAW), microwaves, and photoluminescence. The goal of the experiment was to probe the energy-momentum dispersion of the collective modes—magnetophonons—of the system. One can think of the magnetophonons as undulations of the stripes under the influence of a weak in-plane electric field and a strong transverse magnetic field (Fig. 1). According to the interpretation given in the article, the SAW impart momentum to the system (“stir” it), while the electromagnetic radiation imparts energy (“microwaves” it). When this combination of energies and momenta match its dispersion relation, the system starts to resonantly absorb the energy. This causes heating of 2D electron gas, which is detected from the enhancement of photoluminescence signal.

Although both SAW and microwaves have been used to study quantum Hall systems, the combination of the two has been applied only once: Two years ago this very team used it to reveal the spectrum of collective modes of FQHE states at very high magnetic fields where all electrons reside in the lowest Landau level [6]. This task calls for considerable experimental skills to maintain the temperature in the tens of millikelvin range—with a dilution refrigerator—in the presence of radiation and strong magnetic fields. Though we do not yet understand how this sophisticated technique works, the ingenious method of exciting a system by simultaneous action of light and sound has proven to be powerful and uniquely suited to investigation of 2D electrons in quantum wells.

At this point, a brief survey of previous experimental results pertaining to the stripe phase is in order. The stripe phase is present near the half-integral filling of the third and higher Landau levels, which correspond to the filling factors from $9 / 2$ and above. In contrast, the first two Landau levels are the realm of the “usual” FQHE states [5]. The distinguishing property of the stripe phase is the anisotropy of its electronic properties, first seen in transport measurements [3,4]. This was a surprise because, as mentioned above, in a macroscopic system one would naively expect domains of random orientations pinned by unavoidable disorder. It is thought that a small anisotropy of electron-lattice interaction in $GaAs$ is responsible for orienting the stripes along the $[110]$ crystallographic direction. Although disorder can pin the stripes and destroy their long-range positional order, their orientation order survives. In such a system, electrons carry current along the stripes more easily than across them [7]. In agreement with this picture, resistance measured along the $[110]$ direction was found to be much lower than that in the perpendicular $[1$ $\bar{1} 0]$ direction.

Anisotropy was also detected in the microwave response [8,9], where a broad resonance was seen at frequency $\sim 0.1 GHz$ , but only for the electric field in the “hard” $[1$ $\bar{1} 0]$ direction. This resonance was attributed to the pinning mode—a characteristic frequency of magnetophonon oscillations localized by disorder. Such pinning modes are found in conventional charge-density waves. Because of pinning, the intrinsic dynamic response of the stripes can be probed only at wavelengths that are much shorter the localization length. This is a regime that the Stuttgart group [1] was apparently able to probe for the first time by utilizing SAW with frequencies up to $50 GHz$ and wavelengths in the $60$ – $300 nm$ range. The capability to access such short wavelengths is the principal novelty of their experimental technique, which sets it apart from all previous investigations.

The two main experimental findings of the Letter areas follows: (i) The magnetophonon spectrum has a strong variation when the SAW is launched along the “easy” transport direction (along the stripes) and a much weaker variation for the “hard” transport direction (across the stripes). (ii) The intensity of the response is higher in the latter case and it shows a considerable enhancement whenever the SAW wavelength is a multiple of $3.6 R_{c}$ , where $R_{c}$ is the classical cyclotron radius ( $35 nm$ in this experiment).

Some of these findings are in line with existing theory but others are unexpected. For example, the dispersion along the easy direction possesses a characteristic minimum (precursor of the bubble phase), in agreement with theory [10]. On the other hand, due to the absence of the restoring force for shear deformations, the collective mode frequency along the hard direction is supposed to vanish [5]. The nonvanishing but weak dispersion observed in the experiment is puzzling, indicating that a finite shear modulus exists, possibly due to pinning. The experimentalists further suggest that the period $3.6 R_{c}$ of the intensity oscillations represents the spatial period of the stripes. However, theoretical calculations—both mean-field [11] and exact diagonalization [12] ones—give a smaller number: $\sim 2.7 R_{c}$ . Appearance of the same characteristic length scale in theory and experiment is encouraging, but the numerical discrepancy warrants additional investigation.

Developing a theoretical model capable of analyzing the results of the Stuttgart experiments will be the necessary first step in this direction. A possibly useful analogy is the theory of acousto-optic effect. On the experimental front, it will be interesting to see if one can measure the collective-mode spectrum of the bubble phases, which replace the stripe phase when the top Landau level becomes either less than $40 %$ or greater than $60 %$ filled. Since the bubble phases are known to have a strong microwave signature, it would help to further validate the new technique of Kukushkin et al. A more challenging project is to try and utilize an in-plane magnetic field, which can induce stripe phases at lower filling fractions $7 / 2$ and $5 / 2$ . Earlier studies [9,13,14] tell us that application of the field along the easy resistance direction can also cause the hard and the easy anisotropy axes to interchange. Measuring collective mode spectra as a function of the in-plane field should therefore shed light on the nature of these phase transitions.

This novel spectroscopic technique may be adapted to measure collective modes in a wide variety of 2D electron systems such as monolayer and multilayer graphene, thin films, etc. If successful, this direction of research would have implications reaching far beyond quantum Hall spaghetti phases.

References

I. V. Kukushkin, V. Umansky, K. von Klitzing, and J. H. Smet, Phys. Rev. Lett. 106, 206804 (2011)
A. A. Koulakov, M. M. Fogler, and B. I. Shklovskii, Phys. Rev. Lett. 76, 499 (1996)
M. P. Lilly, K. B. Cooper, J. P. Eisenstein, L. N. Pfeiffer, and K. W. West, Phys. Rev. Lett. 82, 394 (1999)
R. R. Du, D. C. Tsui, H. L. Stormer, L. N. Pfeiffer, K. W. Baldwin, and K. W. West, Solid State Commun. 109, 389 (1999)
M. M. Fogler in High magnetic fields: Applications in condensed matter physics spectroscopy, Lecture Notes in Physics Vol. 595, edited by C. Berthier, L. P. Levy, and G. Martinez (Springer, Berlin, 2001), pp. 98-138[Amazon][WorldCat]
I. V. Kukushkin, J. H. Smet, V. W. Scarola, V. Umansky, and K. von Klitzing, Science 324, 1044 (2009)
A. H. MacDonald and M. P. A. Fisher, Phys. Rev. B 61, 5724 (2000)
G. Sambandamurthy, R. M. Lewis, H. Zhu, Y. P. Chen, L. W. Engel, D. C. Tsui, L. N. Pfeiffer, and K. W. West, Phys. Rev. Lett. 100, 256801 (2008)
H. Zhu, G. Sambandamurthy, L. W. Engel, D. C. Tsui, L. N. Pfeiffer, and K. W. West, Phys. Rev. Lett. 102, 136804 (2009)
R. Côté and H. A. Fertig, Phys. Rev. B 62, 1993 (2000)
M. M. Fogler, A. A. Koulakov, and B. I. Shklovskii, Phys. Rev. B 54, 1853 (1996)
F. D. M. Haldane, E. H. Rezayi, and K. Yang, Phys. Rev. Lett. 85, 5396 (2000)
W. Pan, R. R. Du, H. L. Stormer, D. C. Tsui, L. N. Pfeiffer, K. W. Baldwin, and K. W. West, Phys. Rev. Lett. 83, 820 (1999)
M. P. Lilly, K. B. Cooper, J. P. Eisenstein, L. N. Pfeiffer, and K. W. West, Phys. Rev. Lett. 83, 824 (1999)

About the Author

Michael Fogler is an Associate Professor of Physics at the University of California, San Diego. His research is in condensed-matter theory. His focus is low-dimensional electron systems such as nanowires, semiconductor quantum wells, and most recently, graphene.