Closing in on the Kagome Magnet
The purview of condensed-matter physics is connecting models of the microscopic world of atoms and electrons to emergent macroscopic phenomena. But it isn’t always easy to make this connection. For decades, physicists have been trying to nail down the macroscopic phase of the ground state of the so-called antiferromagnetic Heisenberg model for a lattice of corner-sharing triangles dubbed the kagome lattice, after a type of Japanese basket weaving. Hai-Jun Liao, from the Chinese Academy of Sciences in Beijing, and colleagues [1] have now opened up a new flank in this hunt by giving numerical evidence that the ground state of this model is an exotic phase known as a gapless spin liquid. The result gives additional evidence that the kagome-lattice material Herbertsmithite, which has been widely studied experimentally [2], is a gapless spin liquid in the ground state.
At low temperature, electrons in a solid can localize, leaving only their spin degrees of freedom relevant. These spins then typically order to form various phases: a ferromagnet, an antiferromagnet, a valence-bond solid, and so on. Given the panoply of such possibilities, it is surprising when, instead, the spins choose not to order. Such a disordered state is called a spin liquid in analogy to the disordered state of an actual liquid. Spin liquids go beyond the standard paradigm used by theorists to classify phases by the symmetries they break.
If one were looking for a spin liquid, as condensed-matter physicists do, the place to search would be a frustrated magnet. To understand what this is, consider the antiferromagnetic Heisenberg model, in which neighboring spins prefer to antialign. Such an interaction is ripe for frustration if the neighboring spins form a triangle, as there would be no way to simultaneously maximally satisfy all three bonds. Forty years ago, Phil Anderson began the search for spin liquids suggesting that triangles connected in a triangular lattice might support a spin liquid [3]. This was eventually shown not to be true, but lattices of connected triangles have been the subject of searches for spin liquids ever since, hence the interest in the kagome lattice. Recent work has begun to converge on the likely possibility that the antiferromagnetic Heisenberg model on the kagome lattice, or kagome Heisenberg antiferromagnet (KHAF), is a spin liquid in the ground state [4–10].
While it might be natural to think that there is but one way to not be ordered—that is, a single spin-liquid phase—there are actually a menagerie of possible spin liquids. When doing spin-liquid taxonomy, one of the first divisions you might make is between a spin liquid that is gapped and one that is gapless. It is into this critical taxonomic debate, between the gapped and the gapless spin liquid, that Liao and colleagues have waded. In a gapped spin liquid, the next closest state in energy is always a finite amount of energy away. In a gapless spin liquid, that next closest state comes down to almost, but not quite, the same energy in the infinite limit.
Computational techniques are the method “du jour” for tackling disordered spin systems. To find the exact ground state of these systems, you ideally want to consider an infinite two-dimensional system; it is only in this limit where phases are well defined, and real materials have so many electrons they might as well be considered infinite. But no methods can give this solution. Instead, one often takes the largest system one can afford to simulate on today’s computers, solves it exactly, and tries to extrapolate to infinite size. Unfortunately, in simulating frustrated quantum systems, this route makes for a slow march toward infinity. The computational time to find a solution using the most advanced methods scales exponentially with the width of the system; assuming Moore’s law for computer processing speed continues, one can simulate approximately an extra lattice row a year. It is being stuck at small systems that has left the problem of finding the ground state of the KHAF unresolved for so long.
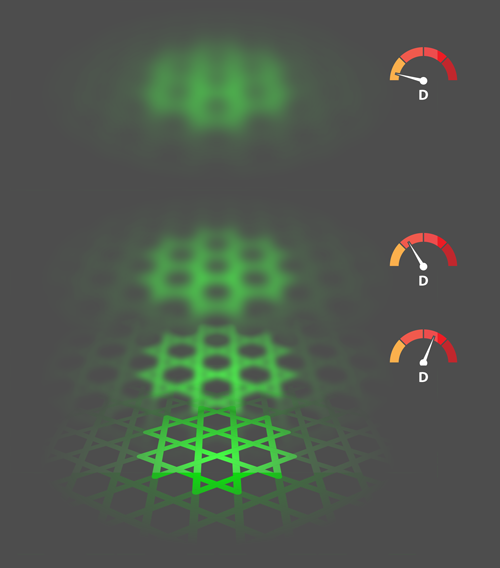
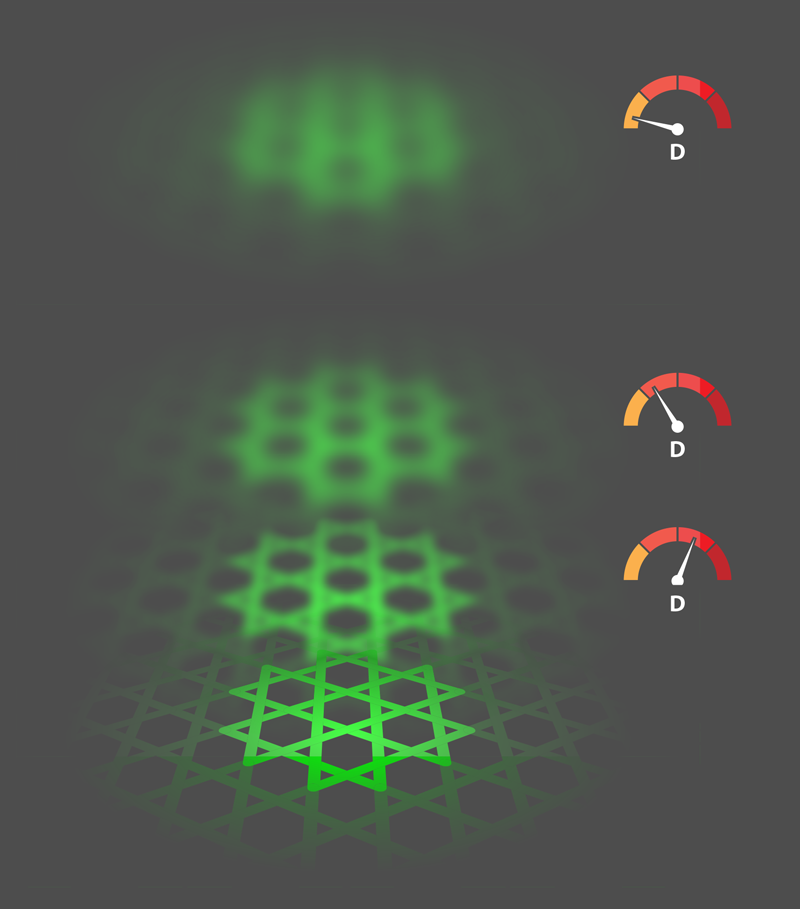
Liao and colleagues have done an end run around this approach. Instead of creeping up from small systems, they work directly in the infinite limit. This is done by optimizing a wave function known as a projected entangled simplex state (PESS), which gives a representation of the infinite system. Unfortunately there is no free lunch: the PESS approach is not exact but gets better as you turn up a parameter (known technically as the tensor bond dimension) in the wave function (Fig. 1). To reach the exact result, you would extrapolate to infinite . This is what Liao and co-workers did to rule out an ordered phase for the ground state of the KHAF. To establish its gapless nature, though, the researchers considered how fast the energy of the wave function approached the exact KHAF ground-state energy as a function of . If the ground state of the KHAF was gapped, we would expect the convergence to the exact result to be exponential with . Instead, the authors find that the energy approaches the exact energy algebraically with . Therefore, they excluded a gapped state.
It’s possible that the label “gapless spin liquid” may stick for the KHAF. Liao and co-workers make a compelling case, and their study is at the leading edge of a number of studies that also make cases for gapless behavior [6–8]. If they’re right, while we can hope to further classify what type of gapless spin liquid the KHAF is, the community should rejoice in finally having nailed the basic nature of one of the most complicated of frustrated magnets. But caveats abound in numerical algorithms. There are many researchers in the “extrapolate-from-small-systems” crowd [4, 5, 9, 10] whose results compare favorably with the result obtained by Liao and colleagues, and yet they argue for the opposing conclusion of a gapped state. KHAF has a long history of surprising us. It may still turn out to be a gapped phase, an ordered phase, a hybrid between them, or even a quantum critical point between two phases [11].
After a decades-long search to understand the KHAF, we can’t say whether this is the last word. But what is clear is the algorithms, experience, and knowledge accumulated in tackling the KHAF have built the edifice that allows us to continue making good on the goal of condensed-matter physics to connect the microscopic to macroscopic.
This research is published in Physical Review Letters.
References
- H. J. Liao, Z. Y. Xie, J. Chen, Z. Y. Liu, H. D. Xie, R. Z. Huang, B. Normand, and T. Xiang, “Gapless Spin-Liquid Ground State in the Kagome Antiferromagnet,” Phys. Rev. Lett. 118, 137202 (2017).
- M. R. Norman, “Colloquium : Herbertsmithite and the Search for the Quantum Spin Liquid,” Rev. Mod. Phys. 88, 041002 (2016).
- P. W. Anderson, “Resonating Valence Bonds: A New Kind of Insulator?,” Mater. Res. Bull. 8, 153 (1973).
- S. Yan, D. A. Huse, and S. R. White, “Spin-Liquid Ground State of the Kagome Heisenberg Antiferromagnet,” Science 332, 1173 (2011).
- S. Depenbrock, I. P. McCulloch, and U. Schollwöck, “Nature of the Spin-Liquid Ground State of the Heisenberg Model on the Kagome Lattice,” Phys. Rev. Lett. 109, 067201 (2012).
- S.Jiang et al., “Competing Spin Liquid Phases in the Heisenberg Model on the Kagome Lattice,” arXiv:1610.02024.
- Y. C. He, M. P. Zaletel, M. Oshikawa, and F. Pollmann, “Signatures of Dirac Cones in a DMRG study of the Kagome Heisenberg model,” arXiv:1611.06238.
- Y. Iqbal, F. Becca, S. Sorella, and D. Poilblanc, “Gapless Spin-Liquid Phase in the Kagome Spin-1/2 Heisenberg Antiferromagnet,” Phys. Rev. B 87, 060405 (2013).
- H.-C. Jiang, Z. Wang, and L. Balents, “Identifying Topological Order by Entanglement Entropy,” Nat. Phys. 8, 902 (2012).
- J. W. Mei, J. Y. Chen, H. He, and X. G. Wen, “Gapped Spin Liquid with Topological Order for Kagome Heisenberg Model,” arXiv:1606.09639.
- H. J. Changlani, D. Kochkov, K. Kumar, B. K. Clark, and E. Fradkin, “The Mother of All States of the Kagome Quantum Antiferromagnet,” arXiv:1703.04659.