Meetings: Fluid Instabilities that Grow Like Toes
Sid Nagel began his talk with a demonstration. Whipping out a tube of yellow paint, Nagel squashed a blob of the paint between two glass slides. The blob spread out into a flat disk. Gripping the two slides in different hands, he then pried them apart. The blob vanished. In its place appeared a maze of wispy, feather-like tendrils emanating from a central point. The physicist from the University of Chicago had everyone’s attention at the Tony and Pat Houghton Conference on Non-Equilibrium Statistical Mechanics that took place in Providence, Rhode Island, earlier this month.
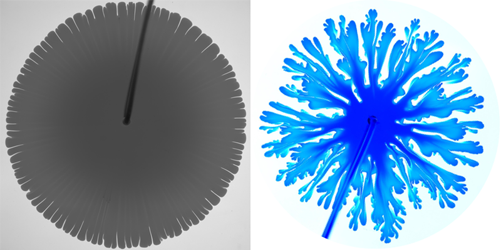
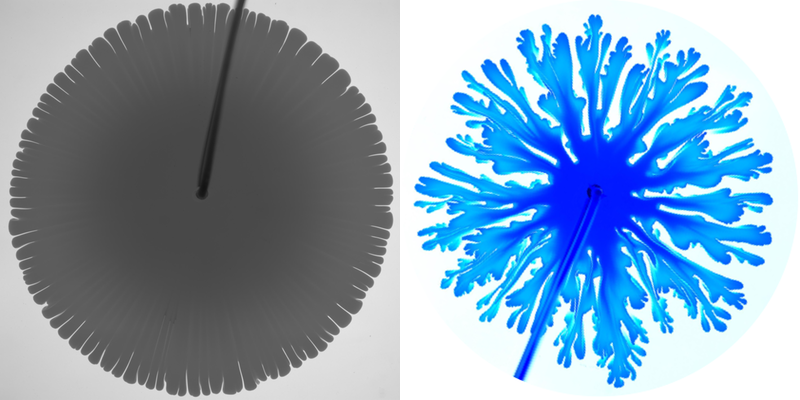
Known as a fingering instability, the pattern Nagel demoed is commonplace, developing when one fluid infiltrates another of higher viscosity—in this case, air invading paint. But Nagel and his colleague Irmgard Bischofberger, now at the Massachusetts Institute of Technology, Cambridge, recently uncovered a new pattern in these much-studied two-fluid systems: short, blunt tubes that grow out of the blob. These tubes bear little resemblance to the long feathery structures of a fingering instability. “I don’t call these fingers, they’re stubby little things. I call them toes,” said Nagel at the conference.
In their experiments, the duo confined a water-glycerol fluid mixture between two glass plates. They then injected a second different mixture of the same two fluids, with a lower viscosity and dyed blue to make it visible, into the system through a hole in the center of one of the plates.
When the viscosity ratio between the two mixtures was small, that is, the viscosity of the invading mixture was tiny compared to the invaded mixture, a fingering-instability pattern appeared. The fingers in the pattern elongated and split repeatedly as more and more of the lower-viscosity fluid was pushed into the system. By contrast, when the viscosity ratio was large (close to 1), no pattern appeared. In this case, the invading mixture took on a circular shape that grew in radius but did nothing else. Nothing unexpected there; both of these regimes have been seen before. But in between these two behaviors, the team found a viscosity sweet spot where something different happened.
“This is a problem that we thought was well understood,” said Nagel. “But if you look at it carefully you see a whole new behavior.”
In this intermediate regime, the interface between the two mixtures starts out disk shaped, akin to Nagel’s squashed blob of paint. After a short time delay, which depends on the exact viscosity ratio, blunt structures—the toes—pop out. The toe pattern grows as more fluid is injected, but unlike the fingers, the toes are stable; they don’t split up and their number stays constant.
Nagel noted another big difference between the fingers and toes: In the toe regime, all parts of the pattern grow at the same rate. If you enlarge snapshots of the pattern at different time steps so that they all have the same diameter, the images are indistinguishable. Such proportional growth has previously only been seen in biological systems. Mammals, for example, grow proportionally, maintaining their overall shape from birth to adulthood. Fluid instabilities tend not to.
Having a nonbiological example of proportional growth could help in understanding how and when this behavior occurs, Nagel said. “It could tell us what are the necessary conditions for having a pattern that shows proportional growth.”
–Katherine Wright