Moiré Effect Could Enhance Neutron Interferometry
Since the early days of quantum theory, its peculiarities have fascinated, worried, and even annoyed physicists. To investigate some of the most puzzling aspects of quantum mechanics, such as wave-particle duality and Schrödinger-cat states, experiments have often utilized the technique of perfect-crystal neutron interferometry, which involves interfering matter waves scattering off crystal planes [1]. But the demands of future experiments require that neutron interferometers advance in performance. A new neutron interferometer—developed by Dusan Sarenac, from the University of Waterloo in Canada, and colleagues—could outperform its predecessor in some important aspects [2]. Their approach utilizes the long-known moiré effect, in which overlaid stripe patterns create a wider periodic pattern. Moiré-based neutron interferometry could be easier to set up, less prone to environmental disturbance, and less wavelength-dependent than perfect-crystal interferometry. As the technique is developed further, it could measure the gravitational constant to higher precision than previous techniques and open new possibilities in materials science.
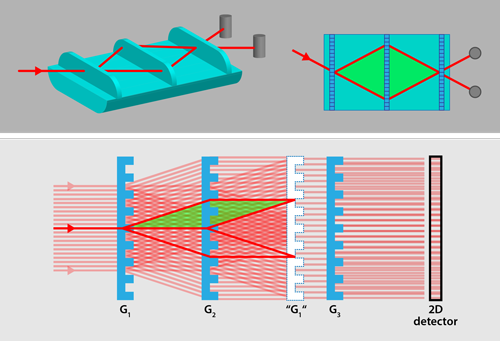
Perfect-crystal neutron interferometry requires aligning several silicon crystal slabs—each approximately the size of a business card—at separations of a couple of centimeters with a position tolerance of a few angstroms. The slabs split the incoming neutron beam by Bragg diffraction such that the resulting paths form an interference loop (Fig. 1). Researchers have cleverly accomplished the very difficult task of slab alignment by taking a large silicon crystal ingot and machining it down so that the different slabs poke out of a common crystal base. Since 1974, numerous pioneering experiments have exploited macroscopic interferometer path separations of up to several centimeters to investigate, for example, nuclear physics, gravitational and inertial effects, topological phases, and entanglement of various degrees of freedom [3].
Despite its success, the perfect-crystal approach has a few limitations. First, the operation of such a device requires maintaining stable environmental conditions with respect to vibrations and temperature fluctuations. Second, perfect crystals only diffract neutrons arriving in a narrow window of wavelengths and angles, meaning that many of the incoming neutrons fly straight through the interferometer. This poses a problem, as the incoming neutron flux is often rather weak, and one would prefer to use as much of it as possible. Third, the precision of some neutron interferometer experiments—such as measurements of gravity—depends on the product of interferometer path lengths and their separation, that is, the area enclosed by the two paths. For perfect-crystal setups, the maximum enclosed area is currently around 100cm2 [4], but extending to larger areas will be challenging, as interferometer fabrication and stable operation becomes more difficult with increasing crystal size.
The interferometer from Sarenac et al. [2] offers some flexibility that perfect-crystal systems lack. Their technique utilizes the moiré effect to create an enlarged pattern from two nearly identical periodic patterns. By analyzing the enlarged pattern, one can infer small differences between the initial patterns. As was done in recent x-ray imaging experiments [5], the researchers utilize the phase moiré effect, in which the patterns are created by periodic structures in transparent materials. In their experiment, the team starts with a neutron beam having a Maxwell-Boltzmann-shaped spectrum with a maximum wavelength of 0.5 nm. Along this beam, they place three transparent silicon gratings with grooves spaced at intervals of 2.4 𝜇m. These grooves induce a phase shift in neutrons that pass through them. By design, the first grating diffracts a neutron ray into three rays: one transmitted and two deflected left and right of the transmitted ray by a small angle of 0.01°. These rays propagate towards the second grating, where they are diffracted again in a way that reunites them with other rays from the first grating, forming the desired interference loops (Fig. 1).
In the plane of reunion, behind the second grating, a wave pattern is formed that is essentially an image of the first grating. The third grating, which is identical to the first one, is not mounted at this image position but close to it. As a result, the neutron wave front enters the third grating with a pattern whose period is slightly larger than that of the third grating, and thus a moiré pattern forms at the detector. The advantage of using the moiré effect is that it creates wide fringes (millimeter scale), which simplifies the detection of shifts in the fringe pattern. The new technique works for a wide range of neutron-beam wavelengths, thus allowing a larger percentage of the incoming flux to be cast into a useful signal, as compared with perfect-crystal interferometry. Moiré neutron interferometry should also be less sensitive to misalignment and environmental disturbances.
The continuous array of interleaved interference loops is sensitive to phase gradients generated by gravity or by small samples inserted in the interferometer. The latter case offers a great opportunity for materials science applications. Placing a sample between the second and third gratings changes the phase of the neutrons, which will modify the moiré pattern, as compared with the empty interferometer. By detecting these changes, researchers can learn about the microstructure within the material.
Neutron interferometers are also important tools in gravity studies. In such experiments, researchers orient the interferometer with one path closer to the Earth than the other [6]. The phase difference between the two paths is then proportional to the enclosed area multiplied by the gravitational acceleration g. Recent experiments have used enclosed areas of around 10cm to measure gravitationally induced phase shifts [7–9]. In the moiré neutron interferometer, the maximum enclosed area was 8cm2, but Sarenac et al. hope to increase this value to greater than 100cm2 by implementing smaller grating periods (which will induce larger diffraction angles) and building longer instruments.
Forthcoming tests will demostrate whether a longer setup can meet expectations with respect to relaxed adjustment and stability requirements. The team’s results show that the fringe contrast (which is the relative difference between bright and dark fringes) decreases as the gratings are moved apart. Moreover, while the maximum contrast observed in this proof-of-principle experiment was 3%, even the theoretical upper limit is only 33% [2, 5], which, in some cases, is bad news since the phase uncertainty is inversely proportional to the contrast.
If the team can manage to extend the length of their setup while maintaining sufficient contrast, they should be able to measure the gravitational acceleration g to a precision of 1 part in 105. The authors also point out that an improved measurement of Newton’s gravitational constant “big G” could be feasible by simply placing a large mass next to the interferometer. Thus a pile of lead bricks on a pallet hanging from a crane, say, could be used to look for possible modifications to the gravitational theory.
This research is published in Physical Review Letters.
References
- H. Rauch, W. Treimer, and U. Bonse, “Test of a Single Crystal Neutron Interferometer,” Phys. Lett. A 47, 369 (1974).
- D. Sarenac et al., “Three Phase-Grating Moire Neutron Interferometer for Large Interferometer Area Applications,” Phys. Rev. Lett. 120, 113201 (2018).
- H. Rauch and S. A. Werner, Neutron Interferometry: Lessons in Experimental Quantum Mechanics, Wave-Particle Duality, and Entanglement (Oxford University Press, Oxford, 2015)[Amazon][WorldCat].
- J. Springer, M. Zawisky, R. Farthofer, H. Lemmel, M. Suda, and U. Kuetgens, “A Large-Area Neutron-Interferometer Optimized for Coherent Beam Deflection: Applications,” Nucl. Instrum. Methods Phys. Res., Sect. A 615, 307 (2010).
- H. Miao, A. Panna, A. A. Gomella, E. E. Bennett, S. Znati, L. Chen, and H. Wen, “A Universal Moiré Effect and Application in X-Ray Phase-Contrast Imaging,” Nat. Phys. 12, 830 (2016).
- R. Colella, A. W. Overhauser, and S. A. Werner, “Observation of Gravitationally Induced Quantum Interference,” Phys. Rev. Lett. 34, 1472 (1975).
- K. C. Littrell, B. E. Allman, and S. A. Werner, “Two-Wavelength-Difference Measurement of Gravitationally Induced Quantum Interference Phases,” Phys. Rev. A 56, 1767 (1997).
- G. van der Zouw, M. Weber, J. Felber, R. Gähler, P. Geltenbort, and A. Zeilinger, “Aharonov–Bohm and Gravity Experiments with the Very-Cold-Neutron Interferometer,” Nucl. Instrum. Methods Phys. Res., Sect. A 440, 568 (2000).
- V.-O. de Haan, J. Plomp, A. A. van Well, M. Th. Rekveldt, and Y. H. Hasegawa, “Measurement Of Gravitation-Induced Quantum Interference for Neutrons in a Spin-Echo Spectrometer,” Phys. Rev. A 89, 063611 (2014).