Quark Picture Put to the Test

Thomas Elias Cocolios

Viewpoint

Quark Picture Put to the Test

Institute for Nuclear and Radiation Physics, Department of Physics and Astronomy, KU Leuven, Leuven, Belgium

November 27, 2023• Physics 16, 199

A measurement of the charge radius of an aluminum nucleus probes the assumption that there are only three families of quarks.

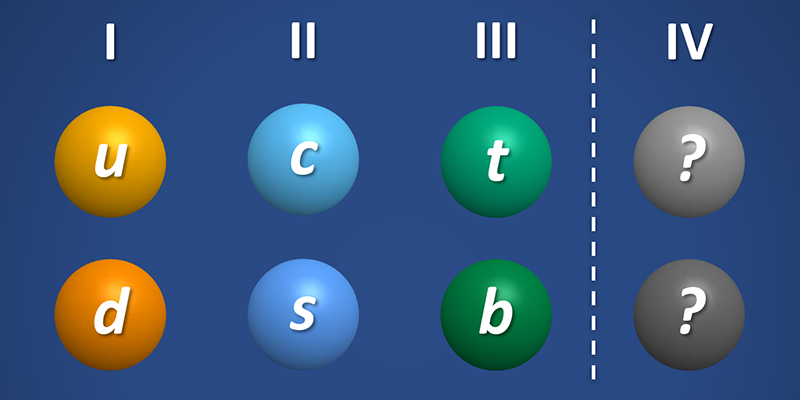
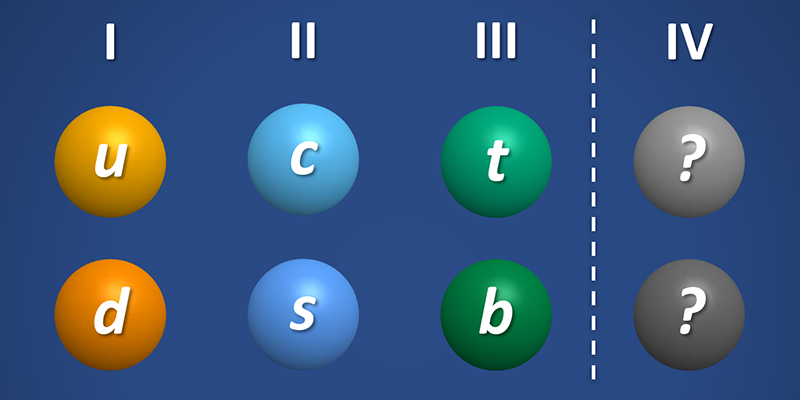
Figure caption — **Figure 1:** The standard model of particle physics considers three families of quarks: up (u) and down (d); charm (c) and strange (s); and top (t) and bottom (b). However, our current understanding does not completely exclude the possible existence of a fourth family.

In the standard model of particle physics, matter is made of elementary particles called quarks and leptons. Quarks are the heavy constituents that form, for example, protons and neutrons, whereas leptons are the light constituents, such as the electron. The six known quarks—up, down, charm, strange, top, and bottom—are split into three families. But could there be a fourth family? Answering that question would require hundreds of different measurements in particle and nuclear physics. However, not all these measurements are yet available or precise enough, and many parameter values are only inferred or extrapolated. Now Peter Plattner at CERN in Switzerland and his colleagues show how a single one of these measurements can shift our understanding of this fundamental question [1].

In the quantum-mechanical framework of the standard model, quarks can oscillate among their different flavors. The best-known example occurs in the beta decay of radioactive nuclei: a proton is transformed into a neutron (or vice versa) when one of its quarks oscillates from up to down (or down to up). The rate of beta decay depends on many factors involving both nuclear and atomic physics, but the rate at which the quarks oscillate is described by a single quantity: V_ud, the so-called matrix element of the transformation of an up quark into a down quark.

When the matrix elements for all the possible combinations of quarks are brought together, one obtains a 3 × 3 matrix called the Cabibbo-Kobayashi-Maskawa (CKM) matrix [2, 3]. If the standard model is complete, then the CKM matrix must be unitary—that is, the sum of the squares of the matrix elements along any row or column must be 1—representing a complete description of all possible quark oscillations. However, should that unitarity test fall short of 1, then another family of quarks is needed to fill the gap (Fig. 1). The search for physics beyond the standard model closely explores all the matrix elements to look for any possible departure from CKM-matrix unitarity. But how precisely can these quantities be measured?

Of all the quarks, the up quark is the most experimentally accessible and provides the most stringent test of CKM-matrix unitarity. And of the three matrix elements involving this quark, the largest and most precisely known is V_ud. However, in the unitarity test, the square of that matrix element is needed, and the uncertainty in V_ud remains the main contribution to the final uncertainty in the sum. V_ud cannot be determined directly but must be extracted from measurements of beta-decay rates—once those have been corrected for nuclear and atomic factors, such as spin and nuclear charge distribution. Of the more than 3000 different radioactive nuclei ever observed in the laboratory, there are a handful in which beta decay is simpler than in the others and in which those corrections are minimal. The long-lived excited state (isomer) of aluminum ^26mAl is one of these—and, thanks to recent efforts, it has one of the most precisely measured beta-decay rates that constrains V_ud [4].

Plattner and colleagues explored how the nuclear charge radius of ^26mAl directly affects the determination of V_ud and thus the testing of CKM-matrix unitarity. Although the charge radius of the ground-state ²⁶Al was already reported [5], that of the isomer had been more elusive, and the value used to evaluate V_ud had been extrapolated. The challenge came from the half-life—6.35 seconds for the isomer versus 717,000 years for the ground state—and from the low production of the isomer. To overcome these issues, Plattner and colleagues studied ^26mAl using two different experiments: COLLAPS at the radioactive-ion-beam facility ISOLDE at CERN [6] and IGISOL CLS at the Accelerator Laboratory of the University of Jyväskylä, Finland [7]. These facilities use different nuclear reactions to generate and extract ²⁶Al and ^26mAl, resulting in different ratios for the production yields of the two nuclear states. To distinguish these states, the team used the difference in half-life at COLLAPS and multiple atomic transitions in aluminum at IGISOL CLS.

Altogether, the two campaigns allowed Plattner and colleagues to extract a value for the ^26mAl charge radius of 3.130 $\pm$ 0.015 fm—significantly higher than the previously reported figure of 3.040 $\pm$ 0.020 fm [5]. The researchers then studied how this new value affects CKM-matrix unitarity. They found a shift closer to unitarity for the top row of the CKM matrix: from 0.99848 $\pm$ 0.00070 to 0.99856 $\pm$ 0.00070.

At first sight, this value differs from 1 by at least 2 standard deviations. However, having seen the impact of a single remeasurement of one input out of hundreds, one might wonder whether some underlying systematic uncertainties have been underestimated—as was the case with ^26mAl. For this reason, experimental nuclear physicists should further explore the different observables involved in the determination of V_ud. In the case of ^26mAl, the accuracy of the evaluated charge radius could be improved with a direct measurement of the charge radius of the ground-state ²⁶Al using so-called muonic x-ray spectroscopy [8]. Moreover, further investigation is needed to accurately determine the charge distribution of a wide range of isotopes [9]. Plattner and colleagues’ findings bring us a step closer to determining whether there is a fourth family of quarks, but many more experimental results are needed before we have a conclusive answer.

References

P. Plattner et al., “Nuclear charge radius of^26mAl and its implication for V_ud in the quark mixing matrix,” Phys. Rev. Lett. 131, 222502 (2023).
N. Cabibbo, “Unitary symmetry and leptonic decays,” Phys. Rev. Lett. 10, 531 (1963).
M. Kobayashi and T. Maskawa, “CP-violation in the renormalizable theory of weak interaction,” Prog. Theor. Phys. 49, 652 (1973).
J. C. Hardy and I. S. Towner, “Superallowed $0^{+} \to 0^{+}$ nuclear $𝛽$ decays: 2020 critical survey, with implications for V_ud and CKM unitarity,” Phys. Rev. C 102, 045501 (2020).
I. S. Towner and J. C. Hardy, “Calculated corrections to superallowed Fermi $𝛽$ decay: New evaluation of the nuclear-structure-dependent terms,” Phys. Rev. C 66, 035501 (2002).
R. Neugart et al., “Collinear laser spectroscopy at ISOLDE: new methods and highlights,” J. Phys. G: Nucl. Part. Phys. 44, 064002 (2017).
L. J. Vormawah et al., “Isotope shifts from collinear laser spectroscopy of doubly charged yttrium isotopes,” Phys. Rev. A 97, 042504 (2018).
A. Adamczak et al., “Muonic atom spectroscopy with microgram target material,” Eur. Phys. J. A 59, 15 (2023).
C.-Y. Seng, “Model-independent determination of nuclear weak form factors and implications for standard model precision tests,” Phys. Rev. Lett. 130, 152501 (2023).

About the Author

Thomas Elias Cocolios is an associate professor at the KU Leuven Institute for Nuclear and Radiation Physics in the Department of Physics and Astronomy, Belgium, where he obtained his PhD in 2010. In his research, he explores the shape of atomic nuclei using advanced laser-spectroscopy techniques and muonic x-ray spectroscopy of stable and radioactive samples. He performs his research mostly at the radioactive-ion-beam facility ISOLDE at CERN, Switzerland, and using muon beams from the Paul Scherrer Institute, Switzerland. He also translates his findings into the production of novel medical radionuclides at the MEDICIS facility at CERN and into the development of the ISOL@MYRRHA facility at the Belgian Nuclear Research Center (SCK CEN).