Quantum Repeater Goes the Distance
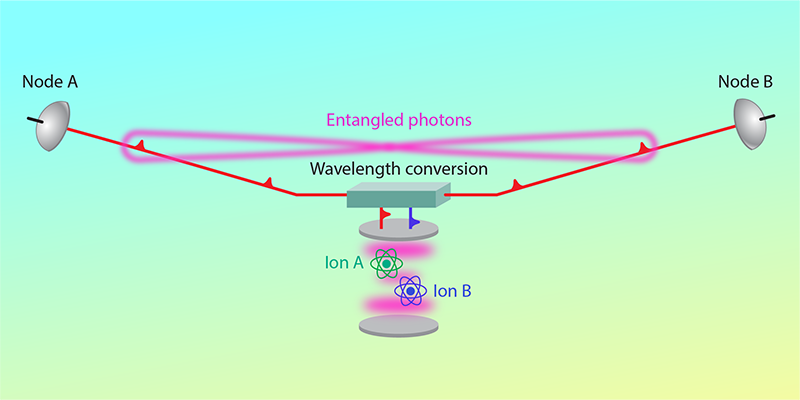
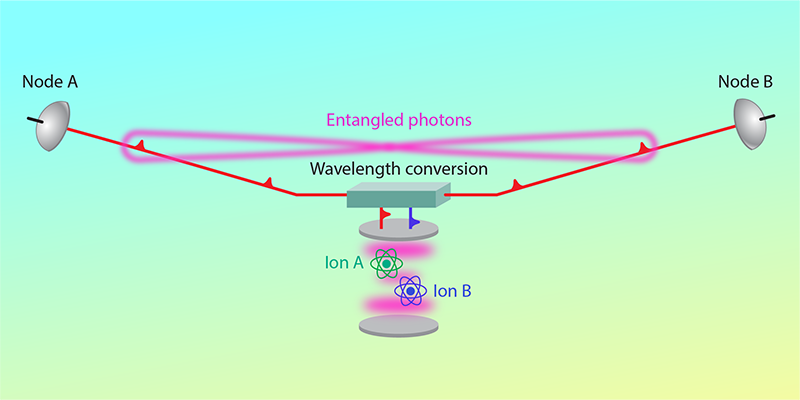
Communication networks have transformed our society over the past half century, and we can scarcely imagine our daily lives without them. Recent advances in the emergent field of quantum technologies have exhilarated scientists about the possibility of linking quantum devices in networks. Long-distance quantum communication portends functionality that is beyond the reach of classical networks [1]. To make full use of entanglement and other quantum effects, quantum networks exchange signals at the level of single photons. As a result, attenuation in fiber is the dominant source of error in these systems. Photon loss, however, can be remedied using a set of intermediate network nodes, called quantum repeaters, which create a direct entangled connection between distant network nodes [2]. A quantum repeater based on nitrogen-vacancy centers in diamond recently achieved the entanglement of two network nodes separated by a distance of 32 m [3]. Now Victor Krutyanskiy of the University of Innsbruck in Austria and colleagues implemented a quantum repeater using trapped ions, which they employed to splice two independent 25-km-long entangled links into a single 50-km-long connection [4] (Fig. 1). This distance is of the order required by practical quantum networks in the real world.
The significance of Krutyanskiy and coworkers’ feat can be appreciated by considering three desirable features that working quantum repeaters should have. The first is the ability to be equipped with quantum memories [5]. Because of photon loss and other inefficiencies in the hardware, the generation of remote entanglement is a probabilistic process. Requiring that an end-to-end connection is established only if all short-distance links simultaneously succeed would lead to an exponentially small overall success rate. By storing short-distance entanglement, quantum memories allow failed links to repeat their attempts at establishing entanglement.
The second desirable feature of quantum repeaters pertains to the photons themselves. Attenuation in optical fibers varies depending on the wavelength of light used to encode the signal. Modern fibers typically carry optical signals at telecom wavelengths—around 1550 nm—at which signal attenuation is at a minimum. It is highly desirable for the quantum repeater to be able to interface with light at these telecom wavelengths [6].
The third desirable feature is related to the “splicing” of entanglement. The repeater generates an entangled state between a stationary quantum memory and a “flying” photon that travels through the fiber. It then repeats the process with a different memory to produce a second flying photon. The two photons are routed to distant network nodes, thereby establishing two independent entangled links. The repeater then splices these links together via a procedure known as entanglement swapping. The splicing should be deterministic, not probabilistic, in order to avoid a reduction in the precious total success rate of end-to-end entanglement.
Krutyanskiy and collaborators integrated all three features into a single system. What’s more, they also successfully distributed entanglement between two network nodes, A and B, separated by 50 km, a distance that could be sufficient for practical applications of quantum networks. The team achieved this feat by trapping two ions of calcium 40Ca+ and using them as two quantum memories. The repeater protocol begins by initializing the two ions to their ground states and by sequentially illuminating them with laser pulses. The laser imparts enough energy to the ions to promote them to a higher-energy level. Subsequent decay of the ions results in each ion emitting a photon, which leaves the ion–photon pair entangled. The photons are collected into a wavelength converter, a device that changes the natural wavelength of the emitted photons to a telecom wavelength appropriate for their journey ahead. The two photons are then guided via 25-km-long spools of optical fiber to node A and node B. Finally, the repeater executes deterministic entanglement swapping on the two ions in its possession, transforming the ion–photon entanglement into a photon–photon entanglement spanning 50 km.
The final photon–photon state is characterized by state tomography, where the entanglement distribution is repeated many times and the photons are measured at nodes A and B in order to build a statistical measure, known as fidelity, of how close the shared photon–photon state is to the ideal one. Unit fidelity signifies a perfect ideal state. The obtained fidelity was 0.72, with nodes A and B obtaining entanglement with a success rate of 9.2 Hz and a success probability of 9.2 × 10−4 per attempt. This fidelity is far above the threshold of 0.5 necessary for the photons to be entangled. Furthermore, the team carried out an experiment in which photon–photon entanglement was distributed over 50 km directly without a repeater. The decreased success rate of 6.7 Hz demonstrated the advantage of using repeater-assisted schemes. This advantage may seem modest at the distances used in the experiment. However, without a repeater the success rate becomes vanishingly small for distances beyond 100 km.
In its study the Innsbruck team asked a thought-provoking question: How much better would the experimental parameters need to be to span an end-to-end distance of 800 km with multiple concatenated repeaters? Surprisingly, several of the parameters require only a minor improvement. The largest advance is needed in the nondeterministic entanglement swapper for photons, which would be required to link multiple repeaters. The researchers present convincing arguments that the improvements are within reach in the near future.
Recent years have seen thrilling experimental demonstrations in quantum communication [7, 8]. Combined with the long-distance capabilities demonstrated in this work, it is clear that quantum networks are rapidly transitioning from theoretical proposals to real-world implementations. Two important lessons learned from a classical network, the internet, must be kept in mind. First, good hardware by itself is not a sufficient path to scalable global communication. It must be accompanied by good software architecture. And second, good software takes a long time to mature. Physicists and engineers are working together to design specialized link layer protocols [9] as well as entire architectures of a future quantum internet [10] to make sure that hardware and software develop hand in hand.
References
- S. Wehner et al., “Quantum internet: A vision for the road ahead,” Science 362 (2018).
- H.-J. Briegel et al., “Quantum repeaters: The role of imperfect local operations in quantum communication,” Phys. Rev. Lett. 81, 5932 (1998).
- M. Pompili et al., “Realization of a multinode quantum network of remote solid-state qubits,” Science 372, 259 (2021).
- V. Krutyanskiy et al., “Telecom-wavelength quantum repeater node based on a trapped-ion processor,” Phys. Rev. Lett. 130, 213601 (2023).
- P. C. Humphreys et al., “Deterministic delivery of remote entanglement on a quantum network,” Nature 558, 268 (2018).
- D. Lago-Rivera et al., “Telecom-heralded entanglement between multimode solid-state quantum memories,” Nature 594, 37 (2021).
- V. Krutyanskiy et al., “Entanglement of trapped-ion qubits separated by 230 meters,” Phys. Rev. Lett. 130, 050803 (2023).
- S. L. N. Hermans et al., “Qubit teleportation between non-neighbouring nodes in a quantum network,” Nature 605, 663 (2022).
- A. Dahlberg et al., “A link layer protocol for quantum networks,” Proc. ACM Special Interest Group on Data Communication 159 (2019).
- R. Van Meter et al., “A quantum internet architecture,” 2022 IEEE Intl. Conf. Quantum Computing and Engineering (QCE) 341 (2022).