Setting Temporal Boundaries for Sound Waves
Waves modify their behavior when they move from one medium to another, as happens when light bends at the boundary between air and water. Similar wave behavior occurs when the properties of a single medium are suddenly switched at a specific moment in time. Experimentalists have studied such “temporal boundaries” for electromagnetic waves and water waves, but creating rapid, uniform changes for acoustic waves has been difficult. Now researchers have designed a mass-and-spring system with tunable stiffness that demonstrates a temporal boundary in acoustics [1]. Their experiment reveals an acoustic-wave behavior that’s analogous to optical refraction, and the demonstrated control of the waves suggests the possibility of trapping and releasing wave signals.
Boundaries are all around us. “When you walk from a dark room into a brightly lit room, you cross a spatial boundary. When you walk through a dark room, and someone suddenly turns the lights on, you cross a temporal boundary,” explains Brian Kim, who recently completed his PhD at the California Institute of Technology (Caltech) and is now a mechanical engineer at the USDA Forest Service. In most cases, a boundary marks a change in the wave speed (or index of refraction). This situation is familiar in optics: a light wave crosses from air into a glass lens, where the speed of light is slower. The wave’s frequency is preserved, but its wavelength decreases to accommodate the speed change. The modified wavelength can result in the bending, or refraction, of the light, as described by Snell’s law. A similar kind of refraction occurs when a wave crosses a temporal boundary: its wavelength is preserved—but its frequency changes to accommodate the differing speed of the wave. “It’s the converse of crossing a spatial boundary,” says Kim.
For studying temporal refraction, “the single greatest challenge has been its experimental realization,” says Chiara Daraio, a mechanical engineering professor at Caltech and the senior researcher on the study. The difficulty is that implementation typically requires a near-instantaneous change in the material properties of the medium through which the waves are propagating. Depending on the physical domain transporting the waves, that change could be related to permittivity, permeability, mass density, elastic modulus, or other properties. Instead of building on previous attempts to uniformly change the properties of a bulk medium, Daraio, Kim, and Christopher Chong of Bowdoin College in Maine constructed a lattice system that they could rapidly change by controlling the properties of its discrete elements. “This approach should be generally applicable for developing analogous systems across physical domains,” Daraio says.
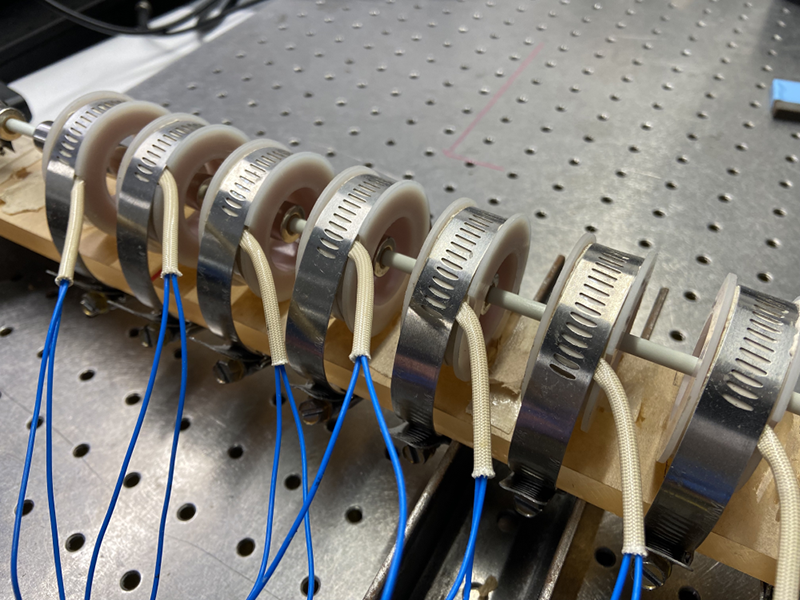
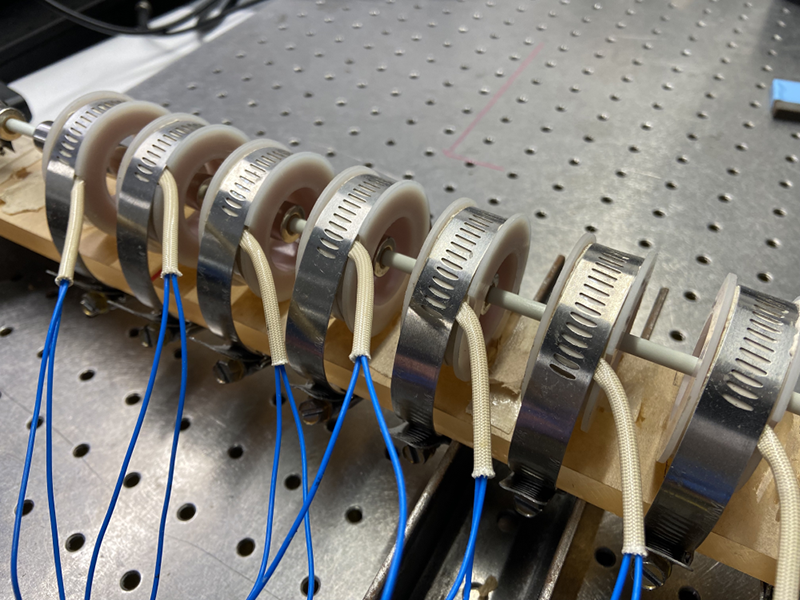
To create their temporal boundary, the researchers designed a 1D lattice made of 12 magnets arranged with alternating polarity so that they all repelled each other, as if they were a chain of ball bearings connected by springs. An electromagnetic coil was installed around each magnet. By applying a voltage to any given coil, the magnetic field generated by the current through the coil acted like a new spring pulling on the magnet. “Turning on” the coils caused the lattice to change, nearly instantaneously, from a loose ball-and-spring chain to a stiff ball-and-spring chain.
The researchers investigated how their temporal boundary affected an incoming traveling wave by using another coil to transmit sinusoidal pulses, between 10 and 30 Hz, across the lattice. They measured the positions of the magnets over time, recovering the frequency and wavelength of the input signal. As a pulse passed through the chain, they turned on the coils and observed that the wave’s spatial wavelength stayed unchanged, but the frequency increased. A similar behavior occurs when a musician tightens a violin string and makes its pitch rise (while the wavelength is constant). The researchers showed that the frequency change matched the predictions based on the temporal analogue of Snell’s law.
The researchers also detected a reflected wave that “bounced” off the temporal boundary and traveled back toward the source. The amplitude of this reflected wave—and that of the corresponding transmitted wave—matched expectations coming from a temporal equivalent of Fresnel’s relations, which traditionally describe the reflectance and transmission at a spatial boundary.
“The experimental setup is original and noteworthy, and it paves the way for observing new phenomena based on time-variable media in the acoustic domain,” says Emmanuel Fort, an experimentalist who specializes in wave–matter interactions at ESPCI Paris. While it would be more interesting to directly change the properties of a continuous medium, discretization offers a simple way to achieve spatiotemporal control, thereby allowing other phenomena to be explored, he adds.
Bumki Min, who designs wave structures for quantum systems at the Korea Advanced Institute of Science and Technology, calls the experiment a “significant milestone.” Notably, it demonstrates that the sound waves (or phonons) in an acoustic system can be controlled more easily—and possibly more precisely—than the light waves in optical systems. “This advantage indicates that the phononic regime may be poised for significant breakthroughs in wave manipulation using time-varying platforms,” he says.
The ability to change the frequency of sound waves and other traveling waves offers numerous possibilities for applications including signal processing and filtering, vibration mitigation, and energy harvesting. “One particularly exciting phenomenon is the ability to dynamically ‘trap’ and ‘release’ traveling waves,” says Kim. Applying a first temporal boundary could dramatically reduce the wave’s propagation speed, essentially trapping it, while applying a second temporal boundary could return the wave to its original velocity, essentially releasing it. Such short-term storage could be useful in processing wave signals in a compact device (see Focus: Stop Light on a Chip).
–Rachel Berkowitz
Rachel Berkowitz is a Corresponding Editor for Physics Magazine based in Vancouver, Canada.
References
- B. L. Kim et al., “Temporal refraction in an acoustic phononic lattice,” Phys. Rev. Lett. 133, 077201 (2024).