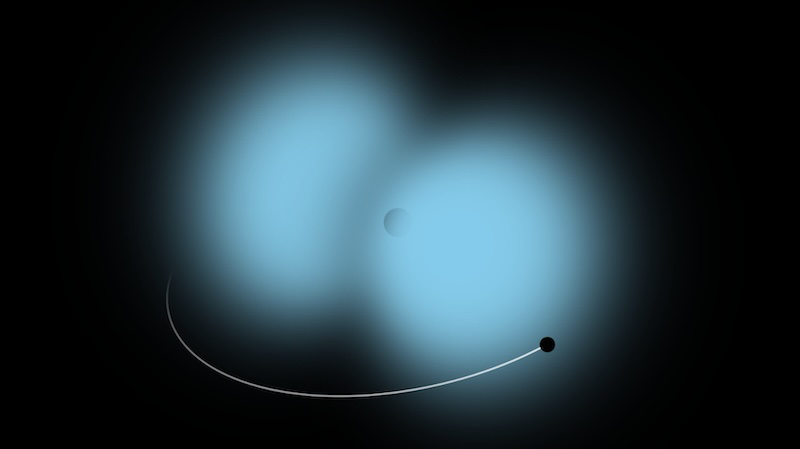
Signatures of Gravitational Atoms from Black Hole Mergers
Subrahmanyan Chandrasekhar famously stated that black holes are “the most perfect macroscopic objects there are in the Universe: The only elements in their construction are our concepts of space and time.” His observation relates to the fact that astrophysical black holes, as described by the Kerr spacetime, can be characterized by just two parameters: mass and spin. However, things might get more complex. Theorists have predicted that if a bosonic field interacts with a Kerr black hole, perturbations in the field can grow to form a cloud around the black hole, creating a “gravitational atom,” in which the bosons surrounding the black hole behave somewhat like the electrons surrounding an atomic nucleus [1] (Fig. 1). What’s more, if such a gravitational atom is part of a binary involving a second black hole, excitations and ionization processes akin to those occurring in hydrogen atoms may affect how the black hole binary evolves. Now Giovanni Tomaselli of the University of Amsterdam and two collaborators have explored the various evolutionary paths such binaries can follow [2, 3]. Considering a general set of black hole orbital motions—including those with eccentricity and inclination—the trio has pinpointed potentially detectable features of gravitational atoms in binaries.
How the lives of black hole binaries in a vacuum play out is a tale that has been told in many different scenarios since the seminal work of Philip Peters in the 1960s [4]. As the binary evolves, the loss of energy and angular momentum carried away by gravitational waves slowly shrinks the binary’s orbit. If the binary is initially in a highly eccentric state, gravitational waves will also efficiently circularize the orbital motion. Therefore, we expect to find vacuum binaries at the end of their lives in a quasicircular motion. The main assumption behind this expectation is that binaries evolve adiabatically through a sequence of quasistationary states. This process unfolds through a balance of conserved quantities. That’s because the sum of the energy and angular momentum possessed by the binary and radiated by the gravitational waves should remain constant throughout the binary’s evolution.
But a rotating black hole need not be in a vacuum. It could conceivably be surrounded by a cloud of bosons. This cloud will grow via the so-called superradiant instability, which amplifies small field perturbations by stealing rotational energy from the black hole and using such energy to power the expansion of the cloud. In their investigation, Tomaselli and his collaborators focused on black holes embedded within a cloud of bosons whose masses lie in the range 10–20–10–10eV. These so-called ultralight bosons arise from several extensions of the standard model of particle physics and include axions that are being considered as dark matter candidates.
If a black hole in a binary has such a cloud—that is, if the black hole is a gravitational atom—the binary’s orbital motion could excite cloud states, causing transitions between energy levels or even “ionizing” the atom [5–7]. The term “gravitational atom” is not a stretch. The cloud’s evolution is described by the Schrödinger equation and the energy levels are hydrogen-like in the nonrelativistic limit. Fully grasping the impact of gravitational atoms on binary evolution is an intricate problem even in the simplest possible scenario. Tomaselli and his collaborators dissected the life histories of gravitational atoms for orbits that have arbitrary eccentricities and arbitrary inclinations of the black hole spin with respect to the orbital plane. They also elucidated many complicated features, such as the occurrence of resonant atom-like transitions and the sudden cessation of transitions between states due to the breakdown of orbital resonances. They characterized some of these features analytically, providing ready-to-use formulas for others.
Two other features the researchers considered are floating and sinking orbits. As a binary evolves, it can enter a resonant state in which the orbital evolution is slowed down because of the transfer of angular momentum between the cloud and the binary. This so-called floating enables the binary to remain longer in a resonant state, which can end the cloud’s existence by depleting it of particles. Sinking orbits, on the other hand, are those that evolve faster such that the orbital motion gives the cloud a chance to survive, as the short time between transitions might not be enough to deplete the cloud. Floating and sinking orbits—together with ionization and the many possible types of resonances (hyperfine, fine, and Bohr)—should be accounted for in order to properly describe the evolution of such atomic binaries.
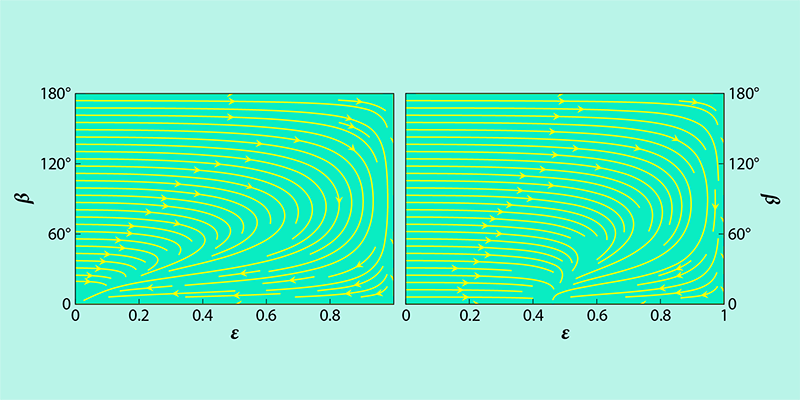
The results derived by Tomaselli and his collaborators enrich the usual picture of vacuum binary evolution in various ways. They show that, even though in most scenarios the cloud easily fades away through a floating resonant process, it leaves a legacy: The binary remains eccentric, and both eccentricity and inclination angle change continuously in a way that depends on the initial state of the system (Fig. 2). This outcome is in clear contrast with the simpler picture of a binary in vacuum, where circularization should be more efficient. This type of evolution leaves an interesting prospect: The presence of the bosons—and possibly the identification of their nature—could be inferred from the orbital parameters of binary populations. Another possibility, also explored by Tomaselli and his collaborators, has the cloud surviving for certain inclination angles of the binary, corresponding to nearly counter-rotating black holes. In such cases, the gravitational atom evolves to a state with sinking orbits. According to Tomaselli and his collaborators, these sinking-orbit binaries can retain their cloud in the inspiral phase, when the gravitational signals produced by the black holes enter the detection window of the Laser Interferometer Space Antenna (LISA) and other future gravitational-wave detectors. These conclusions are corroborated by the findings independently obtained by a team at the German Electron Synchrotron DESY and Hamburg University, Germany [8].
Further work should scrutinize the assumptions of the picture presented by Tomaselli and his collaborators. For instance, the simplifying assumption of adiabatic evolution through a progression of orbits amounts to a bold hypothesis that should be taken with care, as different parts of the orbits might experience different physical effects. Additionally, relativistic corrections might be important to forecast the lives of binaries with gravitational atoms, which was only recently explored in the quasicircular case [9, 10]. Nonetheless, the new work provides powerful guidance in the search for smoking-gun features of binary systems with gravitational atoms.
References
- S. Detweiler, “Klein-Gordon equation and rotating black holes,” Phys. Rev. D 22, 2323 (1980).
- G. M. Tomaselli et al., “Legacy of boson clouds on black hole binaries,” Phys. Rev. Lett. 133, 121402 (2024).
- G. M. Tomaselli et al., “Resonant history of gravitational atoms in black hole binaries,” Phys. Rev. D 110, 064048 (2024).
- P. C. Peters, “Gravitational radiation and the motion of two point masses,” Phys. Rev. 136, B1224 (1964).
- D. Baumann et al., “Probing ultralight bosons with binary black holes,” Phys. Rev. D 99, 044001 (2019).
- D. Baumann et al., “Gravitational collider physics,” Phys. Rev. D 101, 083019 (2020).
- D. Baumann et al., “Sharp signals of boson clouds in black hole binary inspirals,” Phys. Rev. Lett. 128, 221102 (2022).
- M. Bošković et al., “Signatures of ultralight bosons in the orbital eccentricity of binary black holes,” Phys. Rev. Lett. 133, 121401 (2024).
- F. Duque et al., “Axion weak leaks: Extreme mass-ratio inspirals in ultra-light dark matter,” Phys. Rev. Lett. (to be published) arXiv:2312.06767.
- R. Brito and S. Shah, “Extreme mass-ratio inspirals into black holes surrounded by scalar clouds,” Phys. Rev. D 108, 084019 (2023).