Alignment of Cells Affects Secondary Tumor Growth
In a cancer patient, tumor cells that circulate throughout the body in clusters pose a greater threat of metastasis than those that circulate individually. Those clusters are thought to come together while the cells are still within the primary tumor, but researchers still don’t understand the formation mechanism. Quirine Braat at Eindhoven University of Technology in the Netherlands and her colleagues have now used computer simulations to identify some of the factors at play [1].
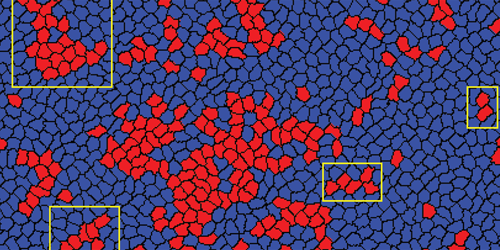
The team used a computational lattice model of cells and tissues (the cellular Potts model) to examine a 2D layer of two types of cells—one motile (able to move) and one nonmotile. The tendency of the motile cells to migrate was represented in the model by an external force applied to each one. For a given cell, this force could align strongly or weakly with the forces acting on its neighboring cells.
By varying the alignment strengths and by tuning the environment through which the cells migrated, the researchers found that the formation of coherent clusters depended on a nuanced interplay between these two factors. When nonmotile cells were sparse and motile cells could move freely, stronger alignment gave larger clusters. However, in a cellular environment dense with nonmotile cells, the largest motile clusters were formed for intermediate alignment strengths.
Real tumors exhibit both densely packed cells and strong cell–cell alignment, suggesting that the formation of large clusters is inhibited. The researchers say that the mean cluster size predicted by the model for such conditions agrees with experimental observations of circulating tumor cells and could have implications for understanding migrating cluster sizes after detachment from the primary tumor.
–Rachel Berkowitz
Rachel Berkowitz is a Corresponding Editor for Physics Magazine based in Vancouver, Canada.
References
- Q. J. S. Braat et al., “Formation of motile cell clusters in heterogeneous model tumors: The role of cell-cell alignment,” Phys. Rev. E 110, 064401 (2024).