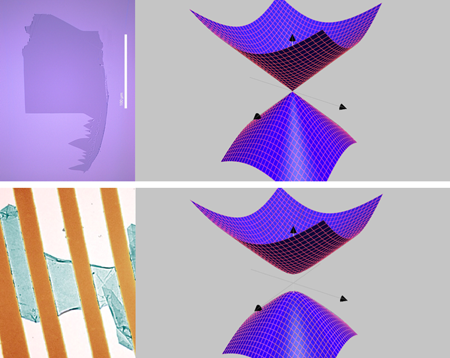
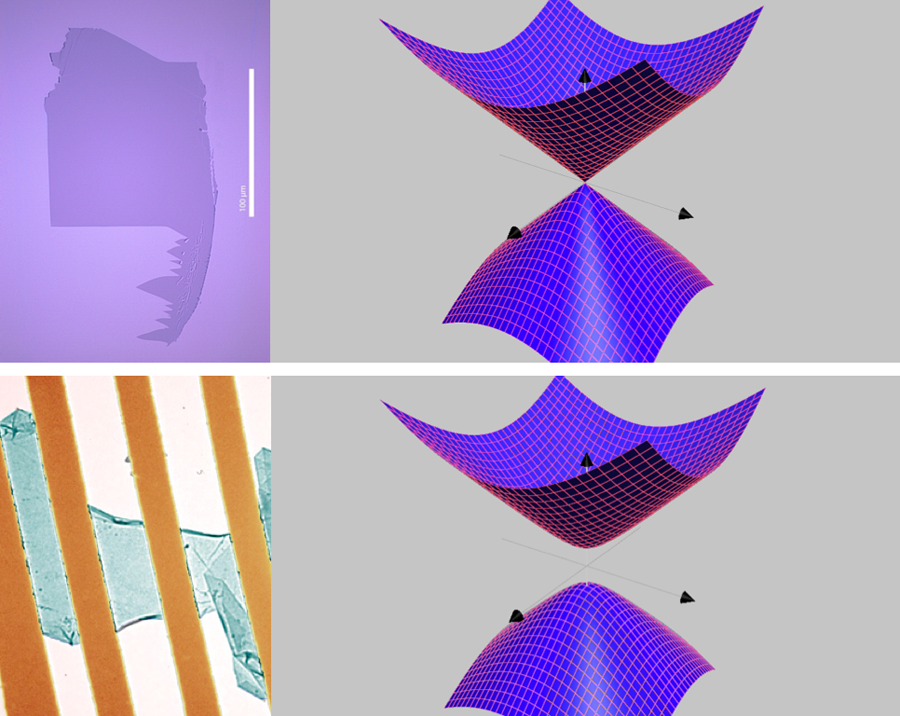
Pauling’s dreams for graphene

Antonio H. Castro Neto

Viewpoint

Pauling’s dreams for graphene

Antonio H. Castro Neto

Department of Physics, Boston University, 590 Commonwealth Ave., Boston, MA 02215

April 20, 2009• Physics 2, 30

Graphene, believed to be a semimetal so far, might actually be an insulator when suspended freely.

Figure caption — **Figure 1:** According to Drut and Lähde’s calculation, graphene on top of ${SiO}_{2}$ (top left, as seen in an optical microscope) is a semimetal with a conical electronic dispersion (top right). Suspended graphene on the other hand (bottom left, bright-field TEM image of a suspended graphene membrane in vacuum) is a semiconductor with a finite energy gap (bottom right).

Graphene research is probably one of the fastest growing fields in condensed matter physics. The material is one atom thick, albeit it can be seen with an ordinary optical microscope [1] (see Fig. 1). It has the properties of a good metal, although its electronic properties do not fit the standard theory of metals because its electrons propagate as massless Dirac particles [2]. Graphene is also resistant against extrinsic impurities because its chemical bonding is very specific and consequently graphene conducts electricity better, with less energy loss, than silicon [3] (the platform of all modern electronics). Moreover, graphene is one of the strongest materials ever measured in terms of Young’s modulus and elastic stiffness [4] (the only other material that is comparable in strength is diamond), nevertheless it is one of softest (the only example of a metallic membrane). It can be used as an ultrasensitive nano-mechanical resonator besides being highly impermeable [5]. Hence it is not surprising that so many high-tech industries are interested in developing graphene-based devices for a plethora of applications, from high-frequency transistors [6] to reversible hydrogen storage [7,8].

However, all the currently proposed applications of graphene are based on the idea that graphene is a semimetal, that is, a system without an electronic gap. In fact, there are many technological advantages for graphene to be a semiconductor instead of a semimetal. The most important one is that the presence of a gap would increase tremendously the on-off ratio for current flow that is needed for many electronic applications. In the last few years, researchers have been trying different ways to produce electronic gaps in graphene but they all come with serious problems. Gaps can be produced by geometrically confining graphene into nanoribbons [9] and quantum dots [10], but those systems are very sensitive to disorder introduced by the cutting process of the graphene sheet. Another possibility is to grow graphene on substrates that induce lattice potentials that can open a gap [11] but the disorder due to the growth process, and the charge transfer between graphene and the substrate, can change the nice electronic properties such as ambipolarity (equal conduction of electrons and holes) that one wants to preserve.

Although graphene has proven to be almost unbeatable in terms of electronic conduction and structural stability, it seems that electron-electron interactions have little effect on graphene’s properties. The lack of strongly interacting states in graphene is rather puzzling given that more than 50 years ago Linus Pauling [12] proposed that graphene should be an insulator due to strong electron-electron interactions, what is today called a Mott insulator [13]. Mott insulators should be contrasted with the more ordinary band insulators, where insulating behavior is generated by electron-ion interaction and can be understood within the independent-electron picture. Pauling based his arguments on the fact that graphene can be thought of as an infinite collection of benzene rings, from which the hydrogens were extracted, and its ground state, just like in benzene, should be a resonant valence bond (RVB) liquid with an electronic gap. Interestingly enough, more or less at the same time as Pauling, Philip Russell Wallace proposed, based on a theory that did not consider any electron-electron interactions, that graphene should be a semimetal [14]. So far Wallace has been “winning the race” but a paper by Joaquin Drut of Ohio State University and Timo Lähde of the University of Washington, published in Physical Review B [15], indicates that Pauling’s dreams for graphene may not be far from reach. Preliminary results were presented first by the same authors in Physical Review Letters [16].

Proposals that electron-electron interactions in graphene could generate an electronic gap were investigated in the context of the properties of graphite (from which graphene can be extracted), and actually preceded the discovery of graphene [17,18]. Because the elementary particles in graphene are Dirac fermions, gap opening is an analogue of the “chiral symmetry” breaking process that occurs in quantum electrodynamics (QED) in two dimensions [19]. Unlike two-dimensional QED, where the fermions propagate at speed of light $c$ , in graphene the Dirac fermions propagate at much smaller velocity $v \sim c / 300$ . The parameter that controls the gap opening is the so-called graphene fine-structure constant, $α_{g} = e^{2} / (ε ħ v)$ , which is the analogue of QED fine-structure constant, $α_{QED} \approx e^{2} / (ħ c) \approx 1 / 137$ ( $e$ is the electron charge, $ε$ is the dielectric constant of the environment, $ħ$ is Planck’s constant).

Since Dirac fermions in two dimensions have a vanishing density of states, the semimetal to semiconductor transition requires a large value of $α_{g}$ , the so-called critical coupling, $α_{c}$ . Thus the gap opening only occurs if $α_{g} > α_{c}$ . The two fundamental questions are as follows: (1) On the theoretical side, what is the value of $α_{c}$ ? (2) On the experimental side, can one find an environment with a sufficiently low $ε$ so that this condition is fulfilled? Notice that $α_{g}$ depends inversely on $ε$ , whose smallest value is $ε = 1$ (the value in vacuum). Hence $α_{g} < α_{g, vac} \approx 300 α_{QED} \approx 2.16$ . For ${SiO}_{2}$ , which is a common substrate for graphene [1], one has $α_{g, SiO} \approx 0.79$ . Because the problem in graphene, unlike QED, is inherently of a strong coupling nature, perturbative approaches [20] are not able to capture this transition and approximate solutions [17,18] can miss important quantum fluctuations. So one has to rely on nonperturbative methods.

Using Monte Carlo simulations that are analogous to the ones used in lattice gauge theory, Drut and Lähde start from the low-energy theory of graphene (the linearization of the spectrum around the Dirac points [2]) and discretize it on a hypercubic lattice [15]. In doing that they lose information of graphene’s honeycomb lattice. Nevertheless, because the system is particle-hole symmetric, the Monte Carlo simulation does not suffer from the infamous “sign problem” that plagues simulations of interacting fermionic systems. After careful analysis of the Monte Carlo data they found that $α_{c} \approx 1.1$ , that is, $α_{g, SiO} < α_{c} < α_{g, vac}$ , and hence the gap opening should be observed for graphene in vacuum but not for graphene on top of ${SiO}_{2}$ (see Fig. 1). With the advent of ultrahigh mobility suspended graphene samples [21–23] it will be possible to reach the value predicted by Drut and Lähde and check Pauling’s 50-year-old prediction.

However, many questions remain: the linearization procedure that is used by Drut and Lähde does not allow for an exact determination of the size of the gap since that depends on the lattice details. If the gap is too small the result will be interesting but purely academic. Moreover, suspended samples are known to have ripples [21] that are the result of the softness of the material and it is not exactly known how those ripples would affect the value of $α_{c}$ . Nevertheless, graphene continues to amaze and present us with new challenges. It seems that this is just the beginning of a new adventure in the world of graphene physics.

Acknowledgments

This research is partially supported by the U.S. Department of Energy under the grant DE-FG02-08ER46512.

References

K. S. Novoselov, A. K. Geim, S. V. Morozov, D. Jiang, Y. Zhang, S. V. Dubonos, I. V. Gregorieva, and A. A. Firsov, Science 306, 666 (2004)
A. H. Castro Neto, F. Guinea, N. M. R. Peres, K. S. Novoselov, and A. K. Geim, Rev. Mod. Phys. 81, 109 (2009)
A. K. Geim and K. S. Novoselov, Nature Materials 6, 183 (2007)
C. Lee, X. Wei, and J. Hone, Science 321, 385 (2008)
J. S. Bunch, S. S. Verbridge, J. S. Alden, A. M. van der Zande, J. M. Parpia, H. G. Craighead, and P. L. McEuen, Nano Letters 8, 2458 (2008)
Y.-M. Lin, K. A. Jenkins, A. Valdes-Garcia, J. P. Small, D. B. Farmer, and P. Avouris, Nano Letters 9, 422 (2009)
S. Patchkovskii, J. S. Tse, S. N. Yurchenko, L. Zhechkov, T. Heine, and G. Seifert, PNAS 102, 10439 (2005)
D. C. Elias, R. R. Nair, T. M. G. Mohiuddin, S. V. Morozov, P. Blake, M. P.. Halsall, A. C. Ferrari, D. W. Boukhvalov, M. I. Katsnelson, A. K. Geim, and K. S. Novoselov, Science 323, 610 (2009)
M. Y. Han, B. Özyilmaz, Y. Zhang, and P. Kim, Phys. Rev. Lett. 98, 206805 (2007)
L. A. Ponomarenko, F. Schedin, M. I. Katsnelson, R. Yang, E. H. Hill, K. S. Novoselov, and A. K. Geim, Science 320, 356 (2008)
S. Y. Zhou, G.-H. Gweon, A. V. Fedorov, P. N. First, W. A. de Heer, D.-H. Lee, F. Guinea, A. H. Castro Neto, and A. Lanzara, Nature Materials 6, 770 (2007)
L. Pauling, The Nature of the Chemical Bond and the Structure of Molecules and Crystals: An Introduction to Modern Structural Chemistry (Cornell University Press, Ithaca, NY, 1960)[Amazon][WorldCat]
P. Phillips, Ann. Phys. NY 321, 1634 (2006)
P. R. Wallace, Phys. Rev. 71, 622 (1947)
J. E. Drut and T. A. Lähde, Phys. Rev. B 79, 165425 (2009)
J. E. Drut, and T. A. Lähde, Phys. Rev. Lett. 102, 026802 (2009)
D. V. Khveshchenko, Phys. Rev. Lett. 87, 246802 (2001)
E. V. Gorbar, V. P. Gusynin, V. A. Miransky, and I. A. Shovkovy, Phys. Rev. B 66, 045108 (2002)
T. Appelquist, D. Nash, and L. Wijewardhana, Phys. Rev. Lett. 60, 2575 (1988)
J. Gonzalez, F. Guinea, and M. A. H. Vozmediano, Nucl. Phys. B 424, 595 (1994)
J. C. Meyer, A. K. Geim, M. I. Katsnelson, K. S. Novoselov, T. J. Booth, and S. Roth, Nature 446, 60 (2007)
K. I. Bolotin, K. J. Sikes, Z. Jiang, M. Klima, G. Fudenberg, J. Hone, P. Kim, and H. L. Stormer, Solid State Commun. 146, 351 (2008)
X. Du, I. Skachko, A. Barker, and E. Y. Andrei, Nature Nanotechnology 3, 491 (2008)

About the Author

Antonio H. Castro Neto is a Professor of Physics and Director of the Condensed Matter Theory Visitor’s Program at Boston University. He received his Ph.D. in 1994 from the University of Illinois at Urbana-Champaign and was a postdoctoral fellow at the Institute for Theoretical Physics in Santa Barbara (1994–1995). Castro Neto has worked on strongly correlated electron systems such as quantum magnets and superconductors. In the last four years, Castro Neto’s work has focused on graphene, a two-dimensional allotrope of carbon. He is a Fellow of the American Physical Society, and currently Divisional Associate Editor for Physical Review Letters and Colloquium Editor for Reviews of Modern Physics.