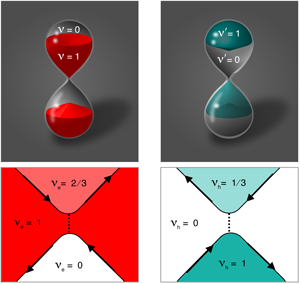
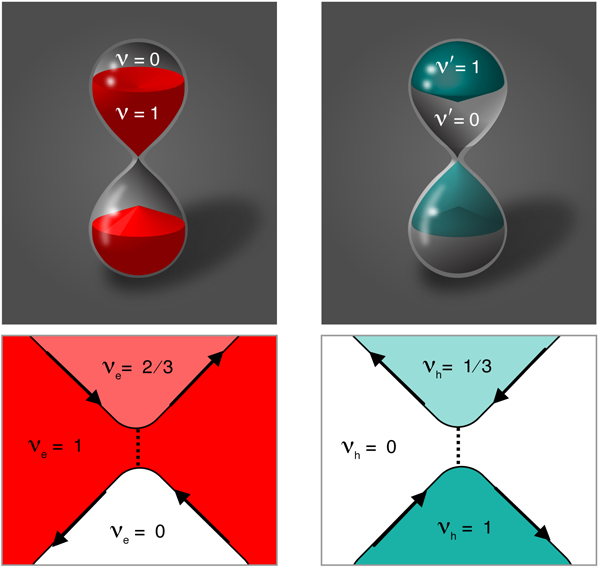
Quasiparticle doppelgängers

Matthew Grayson

Viewpoint

Quasiparticle doppelgängers

Robert R. McCormick School of Engineering & Applied Science, Northwestern University, 2145 Sheridan Road, Evanston, IL 60208-3118, USA

June 29, 2009• Physics 2, 56

By exploiting the concept of particle-hole duality, one can realize a point junction between integer and fractional quantum Hall phases, which constitutes a crucial building block towards possible applications of the quantum Hall effect.

Figure caption — **Figure 1:** Particle-hole duality transforms: holes as doppelgängers for particles. (Top left) Sand particles in the top of an hourglass empty downwards from a filled region, $ν = 1$ , and leave behind an empty region, $ν = 0$ . (Top right) Its dual $ν' = 1 - ν$ can describe the same process, except now the regions “filled” with air in the bottom half “empty” upwards. (Bottom left) Top view of a real sheet of electrons with different electrostatically gated filling factors $ν_{e}$ , bounded by chiral electron currents flowing as shown. (Bottom right) Its dual system is measured in terms of hole filling $ν_{h} = 1 - ν_{e}$ , bounded by opposite flowing chiral hole currents. The dotted line indicates the weak-coupling point where holes tunnel from $ν_{h} = 1$ to probe the $ν_{h} = 1 / 3$ density of states. The desired quantum point contact on the right is extremely challenging to fabricate directly, yet its dual on the left was readily realized by Roddaro and co-workers and reveals the same physics.

The fractional quantum Hall effect earns its “fractional” character from charge carriers having less than the electron charge $e$ [1]. Exploiting the properties of such fractional particles for quantum circuits is a foremost challenge in this field, because the fractional quantum Hall effect hides its most enduring mysteries at its edge—exactly where charges must enter and leave the system if quantum circuits are to manipulate these particles. Recent work by Stefano Roddaro, Nicola Paradiso, Vittorio Pellegrini, and Fabio Beltrami at Scuola Normale Superiore and CNR-INFM in Pisa, Italy, and Giorgio Biasiol and Lucia Sorba at Laboratorio Nazionale in Trieste, Italy, published in Physical Review Letters [2], has successfully realized a long-anticipated device to help unravel the fractional quantum Hall edge puzzle, namely a quantum point contact between different filling factors. The fabrication challenge of such a device is overcome with a clever twist: though the device is made using gated electrons, it functions like its ghostly twin—its doppelgänger—the dual device made up of holes. To understand the importance of such a device, we first review the longstanding questions about fractional quantum Hall effect edges, and then describe the utility of the particle-hole duality transform in the fractional quantum Hall effect for operating this device.

The fractional quantum Hall effect arises when a uniform density $n_{e}$ of two-dimensional electrons $q_{e} = - e$ is placed in a uniform magnetic flux density $n_{ϕ}$ with a constant filling factor ratio $ν = n_{e} / n_{ϕ}$ of electrons per flux quantum [3]. Each flux quantum can be treated as a mobile particle that expels a charge proportional to its area $A = 1 / n_{ϕ}$ and exposes the neutralizing positive background charge, $q_{ϕ} = + A n_{e} e = + ν e$ , thereby satisfying charge neutrality ( $n_{e} q_{e} + n_{ϕ} q_{ϕ} = 0$ ). For certain rational filling fractions $ν$ , Coulomb forces bind a discrete number of flux quanta to each electron, resulting in a gapped ground state with fractionally charged quasiparticle excitations $q^{*}$ [4]. For example, at $ν = 1 / 3$ , two flux quanta are bound to each electron, so $q^{*} = q_{e} + 2 q_{ϕ} = - e / 3$ . Each flux quantum binding to the $N^{th}$ electron induces a node in the wave function of all other $N - 1$ electrons, thus the resulting wave function represents a strongly correlated state.

The fractional quantum Hall effect edge, however, remains enigmatic because as the electron density tapers off at the boundary, it is not obvious that the same uniform-density transformation for quasiparticles would be valid. Wen proposed a powerful theory [5] predicting that the fractional quantum Hall effect edge was a chiral Luttinger liquid whose fundamental properties could be deduced from knowledge of the bulk ground state. As a consequence, he predicted it would be difficult for an electron to tunnel into the correlated fractional quantum Hall edge where the charge would have to be transferred to three $- e / 3$ quasiparticles. The correlations would therefore reduce the tunneling density of states at $ν = 1 / 3$ to $g (E) \sim E^{(1 / ν - 1)} \sim E^{2}$ , with a power-law suppression set by the filling factor. Experiments performed at an extended sharp tunnel barrier along a $ν = 1 / 3$ edge confirmed this power law [6], however, the exponent was slightly below the anticipated value, and values of this exponent at other filling besides $ν = 1 / 3$ deviated sharply from the chiral Luttinger liquid theory [7].

To shed new light on this edge puzzle, Roddaro et al. sought to measure the conceptually simpler case of electron tunneling at a point-contact junction between a $ν = 1$ edge and a $ν = 1 / 3$ edge. This presents a fabrication challenge: because the magnetic field is constant everywhere, regions of different filling factor require different electron density, which is achievable only with electrostatic gates in such close proximity that they would likely short each other. Roddaro’s trick, then, is to borrow from particle-hole symmetry and create a particle system whose complementary hole dual has the desired point-contact structure. Particle-hole duality arises whenever a maximum filling condition, for example $ν = 1$ , is defined for a system of particles, and particle density $ν$ relative to the vacuum can be thought of instead as a hole density $ν' = 1 - ν$ relative to the filled state. This is illustrated in the Fig. 1 (top) cartoon of an hourglass, which empties sand particles from the top, or equivalently “empties” air “holes” from the bottom.

In the fractional quantum Hall device in Fig. 1 (bottom), the spin-polarized filling factor $ν = 1$ can be defined as the filled state, because there is a sufficiently large energetic gap to higher levels. On the left, the real electron system consists of a sheet of electrons at filling factor $ν_{e} = 1$ , which have been locally gated to depletion in the lower region $ν_{e} = 0$ , and to a reduced density $ν_{e} = 2 / 3$ in the upper region. Although the analysis of quasiparticle tunneling might seem complicated in the electron basis, one need only consider its phantom dual system in the hole basis $ν_{h} = 1 - ν_{e}$ to recover its simplicity. On the right of Fig. 1 (bottom), the depleted region can now be thought of as completely filled with holes $ν_{h} = 1$ , coupled at a point-contact to a partially filled $ν_{h} = 1 / 3$ region, and separated by an intervening hole-vacuum $ν_{h} = 0$ . The authors observe the expected suppression in the tunneling density of states when holes tunnel from the $ν_{h} = 1$ into the region with $ν_{h} = 1 / 3$ , verifying the validity of this duality transformation. The authors can also tune the tunnel-coupling at the junction from the weak to the strong limit, and present results which highlight the importance of knowing the exact local filling factor at the point contact for modeling the behavior.

The results are notable for what they do not observe as well. It was predicted that at a strongly coupled $ν = 1$ to $ν = 1 / 3$ point contact, the conductance could reach as high as $G = 1 / 2 e^{2} / h$ and would indicate Andreev-like reflection of quasiparticles and quasiholes [8,9]. In the strong coupling limit, this behavior was not observed, helping to put certain limits on microscopic models of such point contact junctions. Understanding the dual behavior of the phantom holes in these point-contact junctions might eventually lead to real electron devices for non-Abelian quantum computation based on fractionally charged quasiparticles [10]. Glimpsing these quasiparticle doppelgängers might therefore be a good omen of things to come!

References

R. B. Laughlin, Phys. Rev. Lett. 50, 1395 (1983)
S. Roddaro, N. Paradiso, V. Pellegrini, G. Biasiol, L. Sorba, and F. Beltram, Phys. Rev. Lett. 103, 016802 (2009)
D. C. Tsui, H. L. Stormer, and A. C. Gossard, Phys. Rev. Lett. 48, 1559 (1982)
J. Jain, Phys. Rev. Lett. 63, 199 (1989)
X.-G. Wen, Phys. Rev. Lett. 64, 2206 (1990); Phys. Rev. B 41, 12838 (1990)
A. M. Chang, L. N. Pfeiffer, and K. W. West, Phys. Rev. Lett. 77, 2538 (1996)
M. Grayson, D. C. Tsui, L. N. Pfeiffer, K. W. West, and A. M. Chang, Phys. Rev. Lett. 80, 1062 (1998)
N. P. Sandler, C. C. Chamon, and E. Fradkin, Phys. Rev. B 57, 12324 (1998)
D. B. Chklovskii and B. I. Halperin, Phys. Rev. B 57, 3781 (1998)
S. Das Sarma, M. Freedman, and C. Nayak, Phys. Rev. Lett. 94, 166802 (2005)

About the Author

Matthew Grayson is an assistant professor at Northwestern University, Illinois, in the department of Electrical Engineering and Computer Science. His research includes magnetotransport of low-dimensional electron systems such as quantum Hall edges and quantum wires, engineering novel quantum devices to exploit the pseudospin degree of freedom, and investigating thermoelectric properties of unconventional semiconductor superlattices.