Lattice heat destroys coherence
Recent experimental advances in ultracold atomic gases have opened up new avenues to explore a large realm of quantum many-body phenomena. For example, several groups have now reported the realization of Mott insulators using bosonic and fermionic gases [1–3] confined to optical lattices formed by the interference patterns of laser beams. These gases are highly controllable, as various factors such as the strength of interatomic interactions, the lattice geometry and the spin composition can be tuned with great precision, allowing long-standing questions of quantum many-body physics to be addressed from a new angle. These interests are not restricted to atomic and molecular physics, indeed, ultracold atom systems could help to shed light on the origins of high-temperature superconductivity, or provide an opportunity to realize spin liquids.
At present, these high hopes are on hold, pending the ability to cool atom gases to sufficiently low temperatures [4,5], which will require the development of new experimental techniques [6]. Various approaches have been proposed: some rely on the controlled transfer of atoms to a certain quantum state, while other works suggest procedures to decrease the gas entropy. Most efforts have thus been devoted, so far, to optimizing the preparation of cold atom systems. Very few studies, however, have addressed another fundamental question: does the intrinsic heating from the optical lattice itself limit the attainable temperatures, and can this heating be controlled?
Preliminary forays towards understanding this complex problem were only made recently. Gerbier and Castin considered the effect of intrinsic heating on single (noninteracting) atoms confined to an optical lattice [7]. Now, another big step is reported in Physical Review A by Hannes Pichler, Andrew Daley, and Peter Zoller of the University of Innsbruck in Austria [8], where they present their results on how heating affects quantum phases of strongly correlated bosonic atoms.
In Gerbier and Castin’s study [7], the effect of the laser light of the optical lattice on single atoms has been investigated in detail for realistic atomic level structures. From this study, it appears that for red detuned optical lattices, where the frequency of the laser beams making up the lattice is smaller than the resonance frequency of the atom, the dominating scattering process is the absorption of a lattice photon followed by a spontaneous decay to the lowest band. For blue detuned optical lattices, where the laser frequency is larger than the atomic resonance frequency, however, this process is strongly suppressed and only interband transitions are relevant. Therefore, although the total heating rates are identical in both cases, decoherence in the relevant lowest band is strongly enhanced for red detuning. This result is of the utmost importance for experimentalists as it provides quantitative guidelines to help them choose the appropriate lasers to set up their optical lattices.
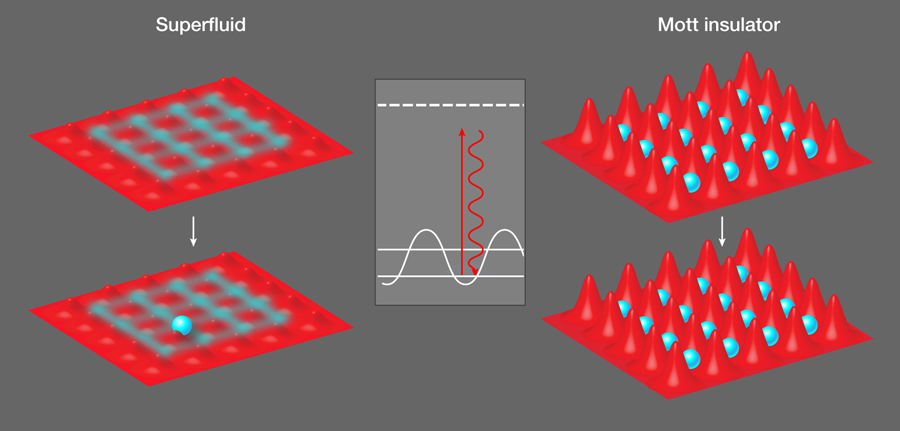
In their new work, Pichler et al. pushed the investigation further by evaluating the effects of intrinsic heating on interacting atoms (many-body states). They first considered the problem of intraband transitions in a red-detuned, one-dimensional, single-band lattice model using master equation formalism. Their careful description of the heating processes combined with a powerful numerical approach—quantum trajectories solved by time-dependent density matrix renormalization group techniques [9,10]—allowed them to characterize the evolution of experimentally measurable observables such as single particle correlation functions. They show that the intraband scattering process leads to localization. Therefore, as presented in Fig. 1, left, quantum states having long coherence lengths, such as a superfluid state, are very much affected by scattering. By contrast, localized states, such as a Mott insulator (Fig. 1, right), prove to be very robust against the intraband scattering process. Pichler et al. also propose that in the considered setups, interband scattering events have less influence on the quantum states, as the atoms promoted to a higher band by scattering events might not fall back into the lowest band within an experimentally accessible timescale.
The theoretical team has convincingly shown that a quantitative characterization of atom-lattice interactions is, in principle, possible, at least for one-dimensional systems. Even though at this stage, many experimentally relevant effects had to be neglected, their result highlights the importance of intrinsic heating and paves the way to more sophisticated theoretical studies. Comparing such model calculations with experiments will help identify the processes that contribute most to the destruction of quantum many-body states. Once these processes have been identified, experimentalists could develop new setups to minimize their unwanted effects. However, for now, it is not clear whether the atom-lattice interaction, the technical noise, or the nonadiabatic lattice ramp [4] constitutes the main source of heating in optimized setups. Despite current difficulties, there is good reason to be optimistic that, in the coming years, the control over ultracold atom systems will continue to improve and allow experimentalists to reach the highly sought after quantum phases that can only be dreamed of today.
References
- M. Greiner, O. Mandel, T. Esslinger, T. W. Hänsch, and I. Bloch, Nature 415, 39 (2002)
- U. Schneider, L. Hackermüller, S. Will, T. Best, I. Bloch, T. A. Costi, R. W. Helmes, D. Rasch, and A. Rosch, Science 322, 1520 (2008)
- R. Jördens, N. Strohmaier, K. Günter, H. Moritz, and T. Esslinger, Nature 455, 204 (2008)
- S. Trotzky, L. Pollet, F. Gerbier, U. Schnorrberger, I. Bloch, N. V. Prokof’ev, B. Svistunov, and M. Troyer, Nature Phys. 6, 998, (2010)
- R. Jördens, L. Tarruell, D. Greif, T. Uehlinger, N. Strohmaier, H. Moritz, T. Esslinger, L. De Leo, C. Kollath, A. Georges, V. W. Scarola, L. Pollet, E. Burovski, E. Kozik, and M. Troyer, Phys. Rev. Lett. 104, 180401 (2010)
- D. Stamper-Kurn, Physics 2, 80 (2009)
- F. Gerbier, and Y. Castin, Phys. Rev. A 82, 013615 (2010)
- H. Pichler, A. J. Daley, and P. Zoller, Phys. Rev. A 82, 063605 (2010)
- S. R. White and A. E. Feiguin, Phys. Rev. Lett. 93, 76401 (2004)
- A. J. Daley, C. Kollath, U. Schollwöck, and G. Vidal, J. Stat. Mech. 2004, P04005 (2004)