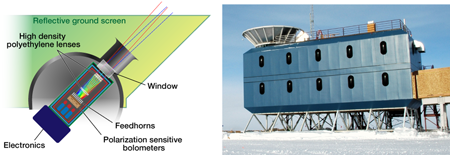
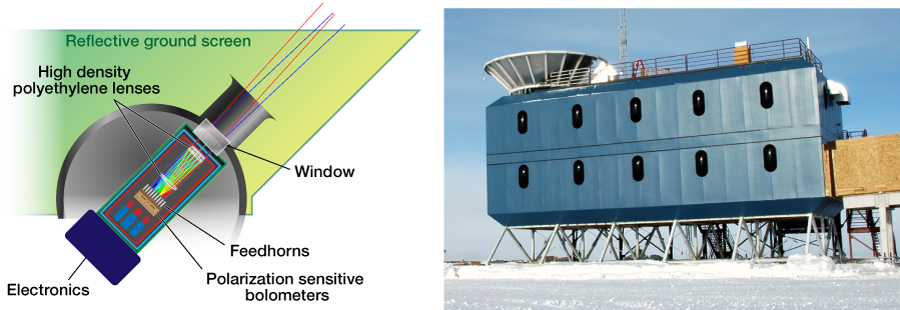
Can we test inflationary expansion of the early universe?

Arthur Kosowsky

Viewpoint

Can we test inflationary expansion of the early universe?

Department of Physics and Astronomy, University of Pittsburgh, Pittsburgh, PA 15260, USA

December 6, 2010• Physics 3, 103

A set of proposed relations among observable quantities may allow strong tests of whether a rapid expansion of the very early universe produced the seeds of the large-scale structure we see today.

Figure caption — **Figure 1:** (Left) Design drawing of the BICEP instrument that measures variations in the polarization of the cosmic microwave background at a level of one part in ten million. The $25$ - $cm$ aperture telescope, with an angular resolution of around a half degree at a microwave frequency of $150 GHz$ , is designed to limit spurious polarization signals from the instrument at this stringent level. BICEP uses 100 polarization-sensitive bolometers cooled to a fraction of a degree Kelvin to detect the polarized radiation. (Right) Photo of the Background Imaging of Cosmic Extragalactic Polarization (BICEP) experiment, which has given the current best limits on the tensor-scalar ratio $r$ from measuring microwave background polarization alone [12]. Such experiments are working towards measurements of $r$ with precision of $0.01$ that are necessary for strong tests of the bootstrap relations proposed by Boyle and Steinhardt. The image shows the Dark Sector Lab at the South Pole, atop which sits BICEP behind the metal ground shield.

Inflation in the early universe is a period of exponential expansion that stretched a small causally-connected patch of the universe by a factor of at least $e^{60}$ into a size large enough to encompass the visible universe today. At the same time this expansion drives the spatial geometry of the expanding region to flatness (for pedagogical reviews, see Refs. [1,2]). Such a period would explain why the universe today appears very nearly spatially flat on average (even though the dynamics of the expanding universe generically drive it away from flatness). It would also explain why the microwave background today has almost the same temperature in all sky directions (even though in the standard cosmology, most points of origin for the microwave radiation we see today were never within each others’ causal horizon, meaning they were never in causal contact). But is it possible to test whether such an inflationary episode actually occurred? In a paper in Physical Review Letters, Latham Boyle of the Canadian Institute for Theoretical Astrophysics in Toronto and Paul Steinhardt of Princeton University propose connections between observable quantities as a check of inflation [3].

The “flatness” and “horizon” problems are intractable enough in the standard cosmology that most cosmologists believe inflation occurred, even though we have no fundamental physical theory that provides a mechanism to drive inflation. If general relativity is correct, accelerating expansion occurs when $p < - ρ / (3 c^{2})$ , where $p$ is the mean pressure and $ρ$ the mean energy density of the universe. Energy density is positive by definition, but pressure can be negative in principle (think of a collection of masses connected by springs stretched from their equilibrium). Cosmologists often invoke a classical scalar field $φ$ , dubbed the “inflaton” field, with some potential $V (φ)$ as a model for the contents of the universe during inflation. The condition for inflation would be satisfied if the potential energy of the scalar field dominates over its kinetic energy. But we do not know what scalar field the inflaton might be, or the energy scale of the field’s potential energy, or indeed if fundamental classical scalar fields even exist in nature.

Inflation is compelling for another reason as well: during a period of exponential expansion, quantum fluctuations in both the energy density and in the gravitational field will inevitably be stretched by the expansion into small-amplitude classical fluctuations on scales larger than the horizon (see Ref. [4] for a review). Any causal influences must propagate at a velocity limited by the speed of light. If the wavelength of a virtual quantum fluctuation gets stretched to a length greater than $c t$ , with $t$ the age of the universe, then the fluctuation’s spatially separated parts cannot communicate, which prevents it from disappearing, and it becomes frozen as a classical perturbation whose wavelength continues to stretch with the expansion of the universe. These fluctuations can be precisely those we see today in the temperature fluctuations of the microwave background; the inflation-generated density fluctuations grow via gravitational instability into the observed large-scale distribution of galaxies, while the gravitational field fluctuations become a stochastic background of very-long-wavelength gravitational waves. The perturbations we can probe today with cosmological observations would have been generated during inflation when the universe was around $e^{60}$ times smaller than it was at the end of inflation. If inflation occurred, it must have started when the universe was less than $10^{- 12}$ seconds old, corresponding to the electroweak energy scale of $100 GeV$ , and may very well have begun at an age smaller than $10^{- 32}$ seconds, corresponding to the scale at which grand unification of the strong and electroweak forces is conjectured to occur (see Chapter $3$ of Ref. [5] for a classic discussion of the thermal history of the universe).

The density perturbations reflected in the observed temperature fluctuations of the cosmic microwave background and the large-scale distribution of galaxies are consistent with a power law as a function of scale, $P (k) = A_{s} k^{n_{s}}$ , with observables of the amplitude $A_{s}$ and power law index $n_{s}$ , where $k$ is the wave number corresponding to a given Fourier mode of the primordial density perturbations. We also consider the possibility that the power law index itself can vary with scale, a quantity defined as $α_{s} = d n_{s} / d ln k$ [6]. The gravitational wave perturbations (or “tensor” perturbations of the metric in general relativity) can likewise be described as a power law with power spectrum $P (k) = A_{t} k^{n_{t} + 1}$ (with, unfortunately, a different historical convention for the power law index!). The precise definition of amplitudes depends on the scale at which they are defined. Assuming that both power spectra are given by these forms, the set of observables we can hope to probe is $A_{s}$ , $n_{s}$ , $α_{s}$ , $A_{t}$ , and $n_{t}$ , with the first two of these being well measured already. (A variation with scale of the tensor power law is almost surely unobservable.) It has long been appreciated that in simple models of inflation driven by a single scalar field, the two power spectra describing the density and gravitational-wave perturbations are not independent. Expanding expressions for measurable quantities in series of so-called “slow-roll” parameters (formed from the expansion rate and its derivatives during inflation) gives a relation between $r = A_{t} / A_{s}$ , the ratio of density to tensor perturbation amplitudes, and $n_{t}$ [7]. Unfortunately, obtaining precise measurements of $n_{t}$ seems unlikely.

Boyle and Steinhardt [3] employ a simple alternate strategy: expand the Hubble parameter (which quantifies the expansion rate of the universe) during inflation, $H (φ)$ , as a Taylor expansion in the value of the inflaton field $φ$ around the field value generating the perturbation scales of interest, $φ_{*}$ [as in Eq. (2) of their paper]. (Note that, while convenient to parameterize the evolution of $H$ in terms of the effects of some effective inflaton field $ϕ$ , this is not necessary and the results do not depend on inflation being driven by an actual fundamental scalar field.) Then, by truncating this expansion after two, three, or four terms and requiring that the resulting functional form for $H (φ)$ be valid until the end of inflation, Boyle and Steinhardt have generated “bootstrap tests”—sets of numerical relations between the observables $r, n_{s}$ , and $α_{s}$ . Current microwave background temperature power spectrum measurements by, for example, the WMAP satellite combined with the higher-resolution ACT experiment, give $n_{s} = 0.962 \pm 0.013$ for a model with $α_{s} = 0$ , and $n_{s} = 1.032 \pm 0.039$ and $α_{s} = - 0.034 \pm 0.018$ for models with both $n_{s}$ and $α_{s}$ as free parameters [8].

The tensor-scalar ratio $r$ can be probed by looking for the “curl” mode in the gradient-curl decomposition of the polarization of the microwave background [9]. So far, WMAP plus ACT measurements give an upper limit $r < 0.25$ at $95 %$ confidence level, based mostly on microwave background temperature fluctuations. Further improvement requires polarization measurements; upcoming experiments (Fig. 1) will likely have the sensitivity and systematic error control to detect a tensor amplitude as small as $r = 0.01$ (provided that contaminating polarized emission at microwave wavelengths from our own galaxy can be understood well enough) [10].

The largest observational hurdle for decisive tests of the full set of bootstrap relations may be measuring $α_{s}$ to sufficient precision; note that the first bootstrap test predicts $α_{s} = - 5.3 \times 10^{- 4}$ . Future measurements of $21$ - $cm$ radiation from neutral hydrogen prior to the reionization of the universe by the first stars and galaxies can, in principle, probe $α_{s}$ at the level of $10^{- 4}$ [11], although this exciting prospect depends partly on control of systematic errors that are not well understood at present.

The bootstrap tests presented here involve only quantities that are potentially observable with current or upcoming experiments. In the absence of inflation, there is no reason why $r, n_{s}$ , and $α_{s}$ should have any particular relationships between them. If the relationships presented in this paper are observed, this is strong evidence in favor of an inflationary origin for primordial perturbations, and constitutes a direct probe of physics at an energy scale far beyond the reach of any particle accelerator. If they are not satisfied, inflation may still be the leading contender for explaining the flatness and horizon problems, but the evolution of $H$ during inflation is then not determined by the first few terms in a Taylor expansion around the field value $φ_{*}$ , corresponding to the scales which we can directly probe observationally. In the words of Boyle and Steinhardt, this “sad circumstance” would mean that the evidence in favor of inflation occurring in the early universe would likely remain circumstantial.

References

A. R. Liddle, in 1998 ICTP Summer School in High Energy Physics and Cosmology, edited by A. Masiero, G. Senjanovic, and A. Smirnov (World Scientific, Singapore, 1999)[Amazon][WorldCat]
W. Kinney, arxiv:0902.1529
L. Boyle and P. J. Steinhardt, Phys. Rev. Lett. 105, 241301 (2010)
J. E. Lidsey et al., Rev. Mod. Phys. 69, 373 (1997)
E. W. Kolb and M. S. Turner, The Early Universe (Westview Press, Boulder, Colorado, 1994)[Amazon][WorldCat]
A. Kosowsky and M. S. Turner, Phys. Rev. D 52, 1739 (1995)
A. A. Starobinsky, Sov. Astron. Lett. 11, 133 (1985); E. J. Copeland, E. W. Kolb, A. R. Liddle, and J. E. Lidsey, Phys. Rev. D 49, 1840 (1994)
J. Dunkley et al., arxiv:1009.0866
M. Kamionkowski, A. Kosowsky, and A. Stebbins, Phys. Rev. Lett. 78,2058 (1997); U. Seljak and M. Zaldarriaga, 78, 2054 (1997)
Y. D. Takahashi et al., Astrophys. J. 711, 1141 (2010)
Y. Mao et al., Phys. Rev. D 78, 023529 (2008); M. Tegmark and M. Zaldarriaga, 82, 103501 (2010)
H. C. Chiang et al., Astrophys. J. 711, 1123 (2010)

About the Author

Arthur Kosowsky is Professor of Physics and Astronomy at the University of Pittsburgh, and Associate Director of the Pittsburgh Particle Physics, Astrophysics, and Cosmology Center (PITT PACC). His research focuses on cosmology, gravitation, and high-energy physics. He is known particularly for work on the cosmic microwave background radiation, and he is a member of the Atacama Cosmology Telescope and the Simons Observatory collaborations to map the microwave sky.