Unraveling Electron Chirality in Graphene

Thomas  Pichler

Viewpoint

Unraveling Electron Chirality in Graphene

University of Vienna, Faculty of Physics, Strudlhofgasse 4, 1090 Vienna, Austria

October 10, 2011• Physics 4, 79

Photoemission performed with circular polarized light can detect the chirality of electronic wave functions in single- and double-layer graphene.

Figure caption — **Figure 1:** Photoemission can be used to detect the chirality of electron wave functions in a doped single layer of graphene. The shaded region in each sketch indicates the part of the graphene electron energy spectrum (the “Dirac cones”) that is accessible for a particular optical polarization: (a) right-circularly polarized (RCP); (b) vertically polarized (VP); (c) left-circularly polarized (LCP); and (d) horizontally polarized (HP). The accessible regions are determined by dipole selection rules. Arrows on the cones indicate the chirality of accessible electrons. The vertical arrow in (a) indicates the binding energy relative to the Fermi level. The phase angle $θ$ is depicted at the Dirac point, where the cones meet.

Carbon atoms in graphene sit on a honeycomb lattice, a seemingly simple structure that nonetheless underlies the electronic and optical properties that have made graphene a world-famous material in recent years [1]. In this lattice, a property of the electrons with the smallest binding energy (i.e., those near the Fermi level) is that they follow a linear dispersion relation confined to two dimensions. The direction along which an electron propagates and the amplitude of its wave function are not independent, so the electrons are said to possess the property of chirality, or handedness. Pinning down fundamental details about these exotic electron states is important for developing graphene-based devices, but measuring this property directly has, however, remained elusive. Now, in a paper appearing in Physical Review Letters, Yang Liu and colleagues at the University of Illinois at Urbana-Champaign have used photoemission with circularly polarized light to detect the chirality, as well as the so-called phase factor, of the wave functions describing electrons in doped single- and bilayer graphene [2]. In addition to establishing a “fingerprint” of graphene electron chirality in photoemission measurements, Liu et al. demonstrate a technique with wide applicability to other two-dimensional materials.

Graphene was discovered in 2004. Since then, experimentalists have found, in this one material, evidence for an unconventional room-temperature quantum Hall effect (QHE), a fractional QHE, ambipolar transport, and exceptionally high charge mobility—the latter an important property for engineering ultrafast devices. Such phenomena, among others, make graphene a promising platform for all-carbon nanoelectronics [1,3].

These properties of single- and bilayer graphene, which are both semiconductors with a vanishing band gap if they don’t interact with the environment, depend crucially on the quasiparticle band structure and the unusual chiral phase relations of the wave functions describing the valence and conduction electrons [4]. Close to the Fermi level, the energy of a graphene electron moving in the honeycomb lattice varies linearly with its momentum—this is why the electrons are said to behave as massless Dirac particles. In addition to possessing a physical (magnetic) spin, the charge carriers in graphene also possess a pseudospin, which is a quantum labeling associated with the fact that the unit cell of the honeycomb lattice consists of two different sublattices.

In addition to spin and pseudospin, a third quantum label for graphene electrons is the two-component isospin degree of freedom, also called the valley index. Graphene’s conduction and valence bands are defined by two inequivalent sets of “Dirac cones,” which sit at the points $K$ and $K^{'}$ in the Brillouin zone. The isospin degree of freedom arises from the degeneracy of the electronic states associated with these inequivalent zone corners. It is the property of isospin that gives electrons in graphene a chirality: their energy spectrum is isotropic, but the relative phases of the two components of the spinor eigenstates (up or down) rotate with the propagation direction of the electron, which tends to align the pseudospins (parallel or antiparallel, respectively) to this direction. In monolayer graphene, the direction of this pseudospin vector tracks the rotation of the electron momentum, resulting in a Berry’s phase of $π$ [5,6], but in bilayer graphene, the pseudospin rotates twice as fast, resulting in a Berry’s phase of $2 π$ [3].

The ability to unravel these electronic properties is a crucial ingredient for the design and optimization of novel graphene-based devices. Therefore, angle-resolved photoemission spectroscopy (ARPES), which in its simplest form measures the energy and momentum of electrons kicked out from the surface by an incident photon, has been employed extensively to directly probe the quasiparticle band structure of graphene systems. ARPES has allowed experimentalists to determine the conical band dispersion relations [7,8] and Dirac cone [9], as well as basic correlation effects, such as the quasiparticle dynamics and the strength of the coupling between electron-plasmon and electron-phonon [8,10].

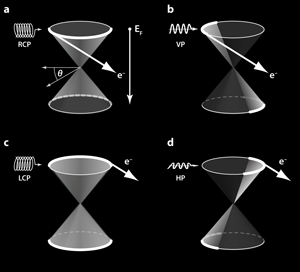
ARPES measurements that are sensitive to changes in the polarization of the incident light can also be a powerful tool for probing the phases of electronic wave functions. This technique is well developed for studying molecular gases, but in solid-state systems, hybridization between electron orbitals or electron correlation effects greatly complicate the analysis [11]. In their work, Liu et al. use monolayer and bilayer graphene as model systems with which to study circular dichroic effects in ARPES applied to weakly correlated, 2D solid-state systems. In agreement with previous studies that use linear polarized light, Liu et al. show that the intensity patterns in an ARPES measurement have a maximum along the direction between the Dirac cone and the center of the Brillouin zone of graphene. Linearly polarized light can probe the phase relation of the wave function but is insensitive to the chirality of the wave function, as the resulting intensity variations don’t depend on the sign of the so-called phase angle $θ$ . This angle is defined as the angle between a line pointing from the Dirac cone to the Brillouin zone center and the momentum transfer of the electrons on the Dirac cone [see Fig. 1(a)], and is directly related to the phase factors associated with the two graphene sublattices: $e^{\pm i θ / 2}$ . It changes sign depending on whether the electron is in the valence or conduction band, and determines the chiral properties of the electronic states. The phase factor gives rise to a Berry’s phase of $π$ by incrementing the azimuthal angle $θ$ by $2 π$ . Liu et al. present a straightforward model to calculate this photoemission intensity as a function of the phase angle $θ$ , and prove that using circularly polarized light allows them to unambiguously determine the chirality of the graphene electrons.

Figure 1 provides a sketch of the angle dependence of the ARPES photoemission intensity as a function of light polarization. The fact that the ARPES intensity pattern depends on the handedness of the incident light (left or right circularly polarized) is evidence that the electron wave functions also possess a handedness. Figure 1 is specific to single-layer graphene, in which the intensity pattern rotates by $π / 2$ as the polarization of the incident light shifts from right-hand circular, to vertical, to left-hand circular, to horizontal. This rotation is what is expected for a Berry’s phase of $π$ in single-layer graphene. In addition, Liu et al. also analyze the chirality of electrons in bilayer graphene. In this case, theoretical predictions show that the Dirac cone is split into two pieces and the phase factors associated with the Dirac point is replaced by $e^{\pm i θ}$ and the pseudospin rotates twice as fast as it does for monolayers, resulting in a Berry’s phase of $2 π$ . Liu et al. observe the expected intensity pattern modulation of $π / 4$ confirming the Berry phase of bilayer graphene.

Using ARPES, Liu et al. have provided a significant step towards the unambiguous visual determination of the chirality of wave functions of the electronic states in weakly correlated solid-state systems. Their work proves that ARPES with circularly polarized light is a reliable tool to probe the chirality of electronic states in solid-state systems. This method should be useful in studying other simple 2D solids, as well as a class of materials called topological insulators, where Dirac Fermions and nontrivial Berry’s phases play an important role [12].

References

A. H. Castro Neto, F. Guinea, N. M. R. Peres, K. S. Novoselov, and A. K. Geim, Rev. Mod. Phys. 81, 109 (2009)
Y. Liu, G. Bian, T. Miller, and T-C. Chiang, Phys. Rev. Lett. 107, 166803 (2011)
K. S. Novoselov et al., Nature Phys. 2, 177 (2006)
K. S. Novoselov and A. Gaim, Nature Mat. 6, 183 (2007)
K. S. Novoselov et al., Nature 438, 197 (2005)
Y. Zhang, Y.-W. Tan, H. L. Stormer, and P. Kim, Nature 438, 201 (2005)
S. Y. Zhou et al., Nature Phys. 2, 595 (2006)
A. Bostwick et al., Nature Phys. 3, 36 (2007)
A. Grüneis et al., Phys Rev. B 80, 075431 (2009)
A. Grüneis et al., Phys. Rev. B 79, 205106 (2009)
D. V. Vyalikh et al., Phys. Rev. Lett. 100, 056402 (2008)
A. A. Taskin and Y. Ando, Phys. Rev. B 84, 035301 (2011)

About the Author

Thomas Pichler is a professor at the University of Vienna. He obtained his Ph.D. in 1993 at the University of Vienna and later spent 15 years at the Institute for Solid State Research IFW-Dresden before he returned in 2008 to Vienna. His research interests are in material science, solid-state physics and spectroscopy, mesoscopic physics, correlated systems, carbon nanostructures (nanotubes, fullerenes, graphene), and low dimensional quantum solids. In 2009 he was recognized as an Outstanding Referee by the American Physical Society.