Complex fluids at work

Paulo E. Arratia

Viewpoint

Complex fluids at work

Department of Mechanical Engineering \& Applied Mechanics, University of Pennsylvania, Philadelphia, PA 19104-6396, USA

January 31, 2011• Physics 4, 9

Researchers show how the judicious choice of fluid filler can suppress the turbulent flow that severely hinders the “top-kill” plugging of a blown-out oil well.

Figure caption — **Figure 1:** (Top left) Kelvin-Helmholtz instability that develops when two fluids of different densities slip past each other. (Top right) Plateau-Rayleigh instability that causes a fluid stream to break up into drops. (Bottom) Laboratory experiments mimicking a vertical counterflow in which oil is flowing from below and a mock fluid is injected at the top. (Left) Oil (light) and another Newtonian solution (green) mix; the green fluid is not able to descend. (Right) Oil and a shear-thickening, viscoelastic solution (e.g., cornstarch); the Newtonian fluid and the cornstarch do not mix, and the green fluid is able to descend.

Complex fluids are a broad class of materials found almost everywhere in our lives. Such fluids or materials are present in places like kitchens, petroleum and chemical processes, and the human body. Examples include milk, yogurt, chocolate, cosmetics, colloidal suspensions, blood, and mucus. But what do all these fluids have in common? Complex fluids can be considered homogeneous at the macroscopic (or bulk) scale, but are disordered at the “microscopic” scale, and possess structure at an intermediate scale. What makes complex fluids so interesting is that they exhibit many useful mechanical properties stemming from the variety of these structures. In colloidal crystals, for example, the intermediate scale is set by the size of the organized crystalline structure: If one considers a cup of cornstarch suspension, then the microscopic scale is the matrix that includes both the water molecules and the cornstarch grains ( $\sim 1 μ m$ ), and the macroscopic scale is the size of the cup ( $\sim 10 cm$ ), while the intermediate scale is set by the structural length scale (if any) of the cornstarch grains. The cornstarch suspension will respond quite differently to an applied stress, depending on the grain size, concentration, and on the grain arrangement in the suspending liquid. That is, the macroscopic flow behavior (rheology) of complex fluids is a strong function of the fluid microstructure. One of the main goals in the study of complex fluids is to understand the physical and chemical forces controlling their structural scale in order to engineer materials with unique mechanical properties.

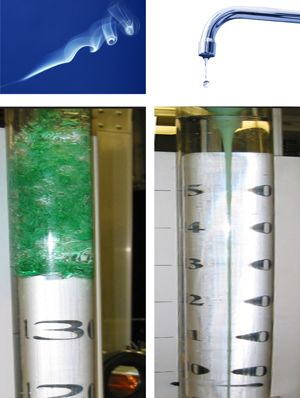
The rheological properties of complex fluids are an important operating parameter in many industrial processes and often affect the quality of the final product. For example, paints are shear-thinning so that they can easily flow under brushing, while shaving creams possess significant yield stress so that they can keep a solidlike shape under static conditions. A single fluid can be shear-thinning, shear-thickening, and viscoelastic depending on the type and magnitude of the applied stress. The extraordinary capabilities of some complex fluids, and their potential uses, are front and center in a new study appearing in Physical Review Letters by Peter Beiersdorfer and collaborators at the Lawrence Livermore National Laboratory and Washington University in St. Louis, both in the US [1]. The researchers propose the use of shear-thickening, viscoelastic fluids to suppress the well-known Kelvin-Helmholtz instability that usually develops at the interface between two fluids of different densities slipping past each other (see Fig. 1, left), and the Plateau-Rayleigh instability that causes a stream of fluid to break up into drops [2,3] (see Fig. 1, right). By using a mock fluid such as cornstarch that thickens at moderate shear rates and is also viscoelastic, the authors propose a strategy that could even have been used to “top kill” the Macondo well that blew out in the Gulf of Mexico in $2010$ , in what is widely acknowledged as one of the most damaging environmental disasters in US history.

In short, the top-kill procedure is the introduction of heavy fluid (drilling mud) at the top of an oil well with the hope of filling or plugging an opened oil well. The less dense oil flows upward in the well, and a counterflow sets in between the oil and the descending mud. As more mud is added, it may eventually go down, holding back the oil. Successful top kill requires that the heavy drilling mud descend despite the fast upward oil flow. However, because of the fast upwelling oil speed ( $> 1 m/s$ ) in the Macondo well, and the rheological properties of the drilling mud that was actually used, the investigators suggest that much of the mud was broken into packets or drops due to the Kelvin-Helmholtz and Plateau-Rayleigh instabilities; even a very dense fluid, when dispersed into small drops, is easily pushed out of the way. As a result, the mud was carried out with the upwelling oil, and the top-kill procedure eventually failed.

The authors’ strategy was to design a drilling mud with desirable rheological properties under the well operating conditions so as to inhibit the Kelvin-Helmholtz and Plateau-Rayleigh instabilities. We know that most drilling muds are not simple Newtonian liquids like water. That is, they do not obey Newton’s law of viscosity, which states that the stress is proportional to the strain rate by a constant of proportionality called viscosity. Drilling muds are often shear-thinning and possess an effective yield stress. Such a fluid remains “rigid” when the shear stress is of smaller magnitude than the yield stress $τ_{0}$ , but flows like a shear-thinning fluid when the shear stress exceeds $τ_{0}$ . These flow properties allow the fluid to remove debris while cutting and drilling, yet keep the debris suspended during interruptions in drilling. In the Macondo well, the oil upwell speed $U$ was approximately $3.7 m/s$ through a bore with diameter $2 R = 0.18 m$ [4]. The Reynolds number, $Re = U R / ν$ was roughly $40 \times 10^{3}$ , where $ν$ is the fluid kinematic viscosity ( $10^{- 5} m^{2} / s$ ); inertial forces dominate over viscous forces, therefore turbulence is expected. By using the Kolmogorov model for turbulence [5], the authors were able to estimate important quantities including the shear stress and shear rates. They noted that the turbulent Reynolds stress $ρ (ε ν)^{1 / 2}$ is larger than $10 Pa$ ( $ε = U^{3} / R$ is the energy dissipation rate of the descending mud), and that the Kolmogorov inner-scale shear rate $(ε / ν)^{1 / 2}$ is approximately $8000 s^{- 1}$ . The Kolmogorov inner scale refers to shearing that is happening at the scale of a fluid particle. Such values of shear stress and shear rate imply that the yield-stress, shear-thinning drilling mud was operating above $τ_{0}$ and probably at a relatively low effective viscosity. Under such conditions—high $Re$ , high shear rates, the presence of density differences and fluid interfaces—the vertical counterflow of two immiscible fluids is ripe for hydrodynamic instabilities that lead to mixing and breakup of fluid parcels.

Beiersdorfer and collaborators suggest the use of a shear-thickening, viscoelastic fluid rather than a shear-thinning, yield-stress fluid in order to suppress both the Kelvin-Helmholtz and Plateau-Rayleigh instabilities (see Fig. 1, bottom) The most obvious effect of using a fluid like a cornstarch suspension is that as the shear rate increases the fluid viscosity will also increase. As a result, the Reynolds number will decrease considerably and turbulence may be avoided. The investigators used a cornstarch suspension as a mock fluid in their experiments to illustrate the principle. The rheological properties of cornstarch suspensions are fairly complex and difficult to obtain [6]. From low ( $0.1 s^{- 1}$ ) to medium ( $10 s^{- 1}$ ) shear rates, the fluid shear-thins. Above a certain shear rate ( $> 10 s^{- 1}$ ), the fluid shear-thickens to viscosities on the order of $10^{3} Pa \cdot s$ (a million times the viscosity of water). The authors suggest that under the Macondo well flow conditions, using a shear-thickening drilling mud would create conditions for a $Re$ of order 1. Hence the Kelvin-Helmholtz instability should be damped by viscosity.

Aside from being shear-thickening, cornstarch suspensions also display viscoelastic behavior. That is, the suspension possesses both fluidlike and solidlike behavior and time-dependent rheology. A very simple constitutive model for viscoelastic fluids is the Maxwell model, which is represented by a purely viscous damper and a purely elastic spring connected in series [7,8]. Elasticity can give rise to behavior that is markedly different from that of Newtonian fluids, particularly in two-phase flows. Consider a viscous Newtonian fluid filament or thread falling through a quiescent bath, while being squeezed by the action of capillary forces. These forces cause the filament diameter to break up into drops, by constricting parts of it at a constant rate proportional to $σ / μ$ , where $σ$ is the surface tension and $μ$ is the inner fluid thread viscosity [9]. Instead, for viscoelastic fluids, the filament diameter decreases exponentially in time, at a rate that is inversely proportional to the fluid relaxation time $λ$ or elastic modulus, resulting in longer breakup times and elongated fluid threads [10–12]. In some cases, the elastic stresses inside the fluid thread become so large that they are able to suppress capillary instability and drop breakup altogether. That seems to be what the investigators observed in their bench-top experiments (see Fig. 1, bottom).

By constructing a fluid with desirable rheological properties tuned to specific operating conditions, the researchers thus eliminated two different instabilities in one stroke, engineering a material (complex fluid) that could be successfully used in the “top kill” process of an oil well, similar to the Macondo well. This is a provocative example of how the understanding of rheology of complex fluids can be used to solve very challenging problems in science and technology. It should come as no surprise that the design of fluids with unique material properties is attracting excited attention in physics, engineering, and even medicine.

References

P. Beiersdorfer, D. Layne, E. W. Magee, and J. I. Katz, Phys. Rev. Lett. 106, 058301 (2011)
G. K. Batchelor, An Introduction to Fluid Dynamics (Cambridge University Press, Cambridge, 1967)[Amazon][WorldCat]
J. S. Turner, Buoyancy Effects in Fluids (Cambridge University Press, Cambridge, 1979)[Amazon][WorldCat]
T. J. Crone and M. Tolstoy, Science 330, 634 (2010)
H. Tennekes and J. L. Lumley, A First Course in Turbulence (MIT Press, Cambridge, Massachusetts, 1972)[Amazon][WorldCat]
F. S. Merkt et al., Phys. Rev. Lett. 92, 4 (2004)
R. B. Bird et al., Dynamics of Polymeric Liquids: Vol 1, Fluid Mechanics (John Wiley & Sons, New York, 1987)[Amazon][WorldCat]
R. G. Larson, The Structure and Rheology of Complex Fluids (Oxford University Press, New York, 1999)[Amazon][WorldCat]
J. Eggers, Rev. Mod. Phys. 69, 865 (1997)
Y. Amarouchene et al., Phys. Rev. Lett. 86, 3558 (2001)
P. E. Arratia, J. P. Gollub, and D. J. Durian, Phys. Rev. E 77, 036309 (2008)
G. H. McKinley and T. Sridhar, Annu. Rev. Fluid Mech. 34, 375 (2002)

About the Author

Paulo E. Arratia is an Assistant Professor of Mechanical Engineering and Applied Mechanics at the University of Pennsylvania. He obtained a B.S. in chemical engineering from Hampton University in 1997, and a Ph.D. in chemical and biochemical engineering from Rutgers University in 2003. He worked as a postdoctoral research associate in the Department of Physics and Astronomy at Haverford College and then at the University of Pennsylvania. He is the recipient of the National Science Foundation Career Award in Fluid Dynamics. His research focuses on the dynamics of complex fluids, swimming, microfluidics, and interfacial phenomena.