Proposed Device Would Shape Magnetic Fields
Just as a light beam bends when it hits water, magnetic field lines become distorted when they penetrate an object with magnetic properties. Writing in Physical Review Letters, a team of theorists makes use of that elementary fact to propose a cylindrical device that could sculpt a magnetic field, concentrating its energy in a given region of space. The device could increase the sensitivity of a detector or transfer magnetic energy with increased efficiency from one place to another.
The technique known as transformation optics, established about 15 years ago, has become a versatile tool for designing novel materials to manipulate light. Starting with a light ray traveling in a straight line through a uniform medium, the technique involves imagining how to squish and/or stretch the medium in whatever way is necessary to turn the light’s straight line into a more complex path of some desired shape. This geometrical “transformation” is then mathematically converted into a recipe for the spatially varying electromagnetic properties the original, undistorted medium must have to bend light in the specified way [1].
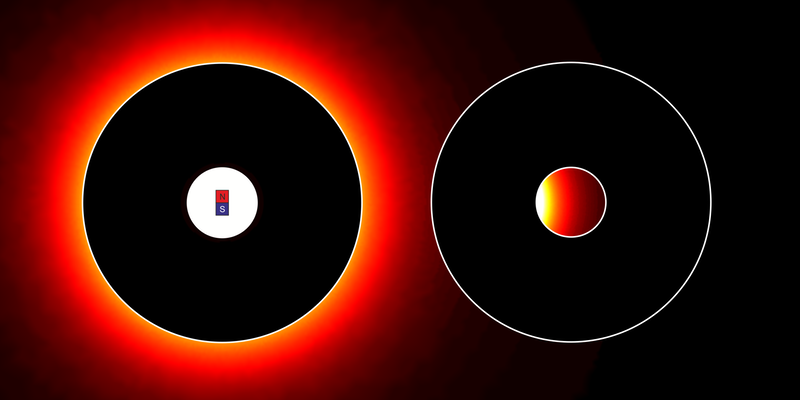
Alvaro Sanchez and his colleagues at the Autonomous University of Barcelona applied the same thinking to static magnetic fields. They wanted to design a device that would squeeze part of a magnetic field into a smaller space, magnifying its energy density and making it easier to detect.
They imagined placing into the field an infinitely long hollow cylinder with an infinitesimally thin shell. Then, keeping the cylinder’s outer diameter fixed, they worked out a transformation that gave the shell a finite thickness and shrank the inner diameter. This transformation compressed the magnetic field in the interior into a smaller volume, increasing its intensity. But because the original thin shell contained no magnetic energy, the finite shell after the transformation also remained free of field energy.
Not surprisingly, the shell generated in this way has odd properties. A magnetic field at the outer surface needs to pass through the shell without hindrance and appear at the inner surface, a feat that requires the property called magnetic permeability to be infinite in the radial direction. The permeability in the direction around the circumference, on the other hand, has to be zero, which would completely suppress any field passing circularly around the shell.
No such material exists. But Sanchez and his colleagues say that a good approximation of the shell could be built by alternating wedges of a ferromagnetic material with wedges of a superconductor. The former transmits a field easily, while the latter blocks it. Such a device, placed in a field generated by an external source, would “harvest” the magnetic field passing through it, concentrating it in the shell’s interior.
The team then showed that if a source of magnetic field, such as a bar magnet, were placed inside the shell, the field outside would be magnified above the value it would otherwise have. Sanchez explains that the shell expels the magnetic field energy to whichever region—interior or exterior—does not contain the source.
Such shells may offer a means of improving the efficiency of wireless energy transmission. A source placed inside one shell will have a greater field strength outside it; another shell not too far away will then concentrate a portion of that field in its interior. Strictly static magnetic fields involve no energy transfer, but the team suggests that the energy of a slowly varying field, such as those used for charging devices wirelessly, could be captured inside the second shell.
“The physics is definitely new and interesting,” says Steven Cummer of Duke University in Durham, North Carolina. He notes that the new proposal is more versatile than existing ways to manipulate fields using magnetic materials. Especially if room temperature superconductors are developed, he adds, building the required devices may eventually be easy.
–David Lindley
David Lindley is a freelance science writer in Alexandria, Virginia.
References
- A. J. Ward and J. B. Pendry, “Refraction and Geometry in Maxwell’s Equations,” J. Mod. Opt. 43, 773 (1996)