Keeping it Cool

Ctirad Uher

Viewpoint

Keeping it Cool

Department of Physics, University of Michigan, Ann Arbor, MI 48109, USA

July 8, 2013• Physics 6, 76

New calculations predict that boron arsenide could become a competitor to diamond as a high-thermal-conductivity material.

Figure caption — **Figure 1:** (a) Structure of cubic boron arsenide (BAs). (b) Phonon dispersion curves for BAs (black) and silicon (red). According to the predictions of Lindsay *et al.* [1], the larger gap between optic and acoustic phonons and the bunching of the three acoustic branches (LA, TA $_{1}$ , TA $_{2}$ ) are among the factors that should lead to a thermal conductivity comparable to that of diamond.

Materials with extreme properties are not only of great theoretical interest but often also hold the key for unique applications. Solids at the two extremes of heat conductivity are an important example. On one hand, very low thermal conductivity is highly desirable for heat-insulation applications, or for thermoelectric energy conversion devices such as coolers, as well as power generators that convert waste industrial heat to electrical energy. On the other hand, there is a great demand for materials with extremely high thermal conductivity, able to rapidly and effectively withdraw heat from local hotspots that could limit the operation of devices such as microprocessors and high-power radio frequency (rf) circuits. As the trend toward smaller and more powerful microchips continues, efficient heat management becomes more and more critical. The theoretical calculations reported in Physical Review Letters by Lucas Lindsay at the US Naval Research Lab in Washington, DC, and co-workers suggest that boron arsenide (BAs), if grown in crystals of sufficiently high quality, should have a thermal conductivity above 2000 watts per meter kelvin (W/m·K), as high as that of diamond [1].

Currently, the best heat conductors are carbon based: diamond and graphite feature room-temperature thermal conductivities of about 2300 W/m·K. Isotopically pure diamond, i.e., diamond grown from carbon possessing a single isotope ( $^{12} C$ ) (as opposed to natural diamond, composed of a mixture of mostly $^{12} C$ and about 1% $^{13} C$ isotopes), improves conductivity by an additional $50 %$ [2]. Natural diamond is expensive, however, and its synthetic forms are often of inferior quality because of the presence of impurities. The next best thermal conductors are in the class of III-V boron-based compounds such as c-BN and BP (cubic boron nitride and boron phosphide), whose experimental room-temperature conductivity is inferior to that of diamond by about a factor of $3$ and $5$ , respectively. The thermal conductivity of other more readily available materials, such as copper and silver—the best among metals—and silicon is an order of magnitude lower than that of diamond.

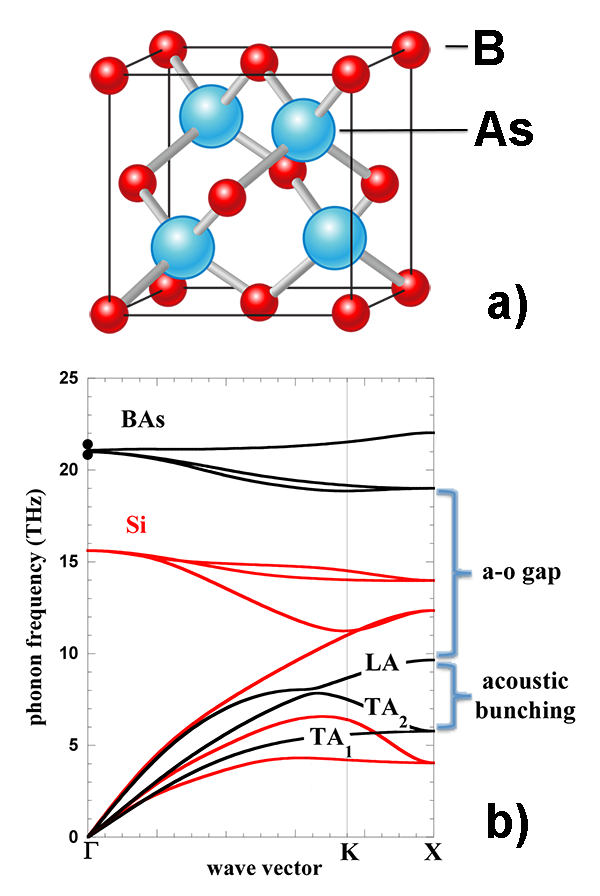
Within this context, it is surprising and exciting to read about Lindsay et al.’s theoretical predictions of diamondlike conductivity values for BAs, a cubic III-V compound [see Fig. 1(a)]. Given the price of its constituent elements, BAs has the potential to be a much cheaper alternative to diamond, should a proper growth technique become available. The result is unexpected: according to a commonly accepted set of criteria for finding high-thermal-conductivity materials, one would never anticipate BAs to be such an efficient heat conductor. Such criteria include low atomic mass, a simple crystal structure, strong interatomic bonding, low anharmonicity of lattice vibrations, and isotopic purity. Instead, BAs contains As (which has a medium-large atomic mass) and a large mixture of B isotopes and has greater anharmonicity than diamond. Previous calculations based on these criteria had suggested a conductivity of 200 W/m·K for BAs, one order of magnitude lower than predicted by the authors.

The new prediction of high conductivity of BAs is based on computations by recently developed ab initio techniques, to whose development the authors provided significant contributions. In nonmetallic crystals (like BAs), heat is primarily transported by lattice vibrations (phonons). The authors applied first-principles calculations and density-functional theory to calculate the fundamental vibrational properties of BAs: phonon frequencies, velocities, lifetimes, as well as the anharmonic couplings responsible for energy transfer between different phonons. From these, the thermal conductivity as a function of temperature can be derived. As the authors explain, the diamondlike value of the thermal conductivity of boron arsenide is the consequence of a fortuitous combination of several factors [see Fig. 1(b)]: a large frequency gap separating acoustic and optic phonon branches, a grouping of the three acoustic phonon branches, and a surprisingly weak influence of isotopes on phonon scattering. It can be shown that these features, which are unique of BAs, reduce the impact of phonon scattering, increasing the lifetimes of phonons and thus allowing them to transport away heat more efficiently. All these aspects combine to give BAs a conductivity that should exceed 2000 W/m·K.

It would have been desirable, of course, if theoretical predictions had been confirmed by experimental measurements of the thermal conductivity of BAs. But boron arsenide samples are not easily available in sizes large enough to carry out reliable room-temperature measurements of the thermal conductivity. It is here that a possible major stumbling block and a challenge to the experimental community lies.

The synthesis of large BAs samples is challenging because of the properties of its constituent elements. Boron has a very high melting point (2075 °C). Elemental arsenic sublimates (614 °C) before it melts (at its melting temperature of 817 °C, its vapor pressure is some $28$ atmospheres) and has a vapor pressure that rises rapidly with temperature, already reaching $1$ atmosphere at around 640 °C. Finding crystal growth strategies compatible with these different properties is a formidable challenge, and the volatility of poisonous As could pose considerable hazards to enthusiastic but inexperienced researchers.

Attempts to grow high-quality BAs crystals have nevertheless been carried out over the past $50$ years, motivated by both potential applications (BAs belongs to the family of III-V semiconductors, whose optical properties are interesting for photovoltaic and photoelectrochemical applications [3,4]) and theoretical interest (theorists suggested that in BAs, being the most covalent of all III-V compounds, boron might even play the role of an anion rather than acting as a typical cation [5]). Several partly successful outcomes are described in the literature [3,6,7].

Attempted growth methods fall into two categories. The first relies on vapor transport: arsenic is placed at one end of a closed quartz tube and kept at around 640 °C, generating a sufficiently high vapor pressure; powdered boron is heated to about 900 °C at the other end of the tube. Over a prolonged period of time (typically $100$ hours or more) the BAs compound forms. The resulting crystals are very small and their 1:1 stoichiometry depends critically on maintaining constant temperature and minimizing impurities, some of which could also leach out from contact with the quartz tube. The second approach relies on the reaction of boron powder with excess of arsenic in a sealed evacuated quartz tube heated to around 800 °C. The much higher temperature to which arsenic is exposed necessitates the use of a thick quartz tube to withstand the vapor pressure of arsenic. Stoichiometric control is more challenging in this case and the resulting material often contains a different phase, typically with B $_{6}$ As composition, which would affect the thermal properties of the crystal. Millimeter-sized crystalline pieces of BAs have been prepared using this approach, but the content of impurities is not known. In either case, only very small BAs have been realized, and likely conductivity measurements would not deliver reliable values because of the presence of impurities.

The exciting predictions of Lindsay et al. may now give a strong impetus towards the improvement of growth techniques, leading to the production of larger, reasonably pure single crystals. The ball falls now in the court of the experimentalists to prove whether this tantalizing prospect will fulfill its expectations, providing an efficient and hopefully inexpensive material for passive heat management in a broad range of electronic and microwave devices.

References

L. Lindsay, D. A. Broido, and T. L. Reinecke, “First-Principles Determination of Ultrahigh Thermal Conductivity of Boron Arsenide: A Competitor for Diamond?,” Phys. Rev. Lett. 111, 025901 (2013)
T. R. Anthony, W. F. Banholzer, J. F. Fleischer, L. Wei, P. K. Kuo, R. L. Thomas, and R. W. Pryor, “Thermal Diffusivity of Isotopically Enriched $^{12}$ C Diamond,” Phys. Rev. B 42, 1104 (1990)
S. M. Ku, “Preparation and Properties of Boron Arsenides and Boron Arsenide Gallium Arsenide Mixed Crystals,” J. Electrochem. Soc. 113, 813 (1966)
S. Wang, S. F. Swingle, H. Ye, F.-R. Fan, A. H. Cowley, and A. J. Bard, “Synthesis and Characterization of a p-Type Boron Arsenide Photoelectrode,” J. Am. Chem. Soc. 134, 11056 (2012)
R. M. Wentzcovich and M. L. Cohen, “Theoretical Study of BN, BP, and BAs at High Pressures,” Phys. Rev. B 36, 6058 (1987)
J. A. Perri, S. LaPlaca, and B. Post, “New Group III-Group V Compounds: BP and BAs,” Acta Crystallogr. 11, 310 (1958)
F. V. Williams and R. A. Ruehrwein, “The Preparation and Properties of Boron Phosphides and Arsenides,” J. Am. Chem. Soc. 82, 1330 (1960)

About the Author

Ctirad Uher is the C. Wilbur Peters Collegiate Professor in the Physics Department of the University of Michigan. He received his Ph.D. degree in physics from the University of New South Wales. His research interests cover a wide area of topics in condensed matter physics, including thermoelectric materials, superconductors, and diluted magnetic semiconductors. For more information visit http://research.physics.lsa.umich.edu/Uher-Lab/cuher-home.html. Dr. Uher is a Fellow of the American Physical Society (APS) and in 2008 he was recognized by the APS as an Outstanding Referee.