All Together Now
The concert is about to start: Brahms’ First. The hall is cacophonous with tuning instruments. The oboe shrieks, the horn blurts out. An instant later, with the downbeat of the baton, perfect harmony sounds. The physics is simple. The conductor brings the instruments—harmonic oscillators—together, locking them in phase. Coherence sets in. Such classical analogies often serve to introduce coherent quantum phenomena, but miss a crucial point: quantum particles are fundamentally indistinguishable. In this orchestral analogy, we cannot ascertain which violin is playing and which one is not. The physicist Robert Dicke worked through this problem some 60 years back [1]. He found that the quantum phases of oscillators can be related even if only one is excited; two violins sound as if they are both playing when only one is bowed. While beautiful in conceptual simplicity, manifestations of such phase coherence (the “Dicke effect”) are challenging to find [2]. Now, Carlo Sirtori from the University of Paris Diderot, France, and colleagues provide evidence for the Dicke effect in infrared (IR) light emission from a semiconductor nanostructure at room temperature [3]. The device constitutes a kind of IR light-emitting diode (LED) with potential applications in biochemical sensing.
Dicke’s theory, one of Physical Review’s collection of papers for UNESCO’s International Year of Light, describes how phase-coherent collective emission emerges in a gas of noninteracting identical atoms. The energy of a photon from a light field shone on the gas can only be absorbed by one of the atoms, which transitions from its ground to its excited state. Because of quantum indistinguishability, it remains undefined which one. The collection of atoms that see the light field is therefore described as being in a quantum entanglement of ground and excited states—much like Schrödinger’s cat is simultaneously dead and alive, entangled between both states. The more atoms present, the more entangled the system becomes. From this entanglement, new atomic transitions emerge with different transition rates. Under specific conditions, each of the individual transitions is shared between the different atoms, so that the interaction rate of the ensemble with the light field increases and the atoms collectively emit light: superradiance occurs, the “atomic quantum-orchestra plays.”
Superradiance is tricky to demonstrate since several requirements must be met by the atoms, or by artificial, atomlike two-level systems. The probe volume must be small, of order the wavelength of light, to ensure that the light field seen by different atoms in the ensemble is the same. In addition, interactions between atoms must be negligible to prevent collisions, which can destroy coherence. Initial experiments studied atomic gases, in which interatomic spacing is high and the Dicke effect correspondingly weak. A striking demonstration of atomic superradiance involved two single trapped ions [4]. Optical superradiance from an ensemble of semiconductor nanocrystals known as quantum dots was also observed [5], and a related form of superradiance in the solid state has been demonstrated in superconductor nanocircuits, which exhibit narrow and discrete microwave transitions with the characteristics of a two-level system [6]. Interestingly, manifestations of Dicke-type coherence are not limited to superradiant light emission. The effect recently even showed up in the current of an organic light-emitting diode (OLED), in which the “up” and “down” spins of the charge carriers make up the coupled two-level systems [7].
In their study, Sirtori and colleagues observe Dicke physics in the light emission of a semiconductor nanostructure, a quantum well. Generally, the absorption and emission of a photon by a material can be described in terms of a harmonic oscillator. Each oscillator is characterized by a certain resonance frequency but also by the width of the resonance. In quantum mechanics, this width is, to a first approximation, determined by the time the photon energy absorbed spends on the atom, or atomlike system, before being released again. For most atomlike systems, reemission of the photon is slow, occurring over nanoseconds, so the resonance width is small. If the heterogeneity of the artificial atoms, that is, the variation in resonance frequency between the different oscillators, is greater than the resonance width of the individual oscillator, entanglement between the oscillators due to the Dicke effect is prevented. Rather than reducing heterogeneity, which is the conventional approach to couple the atomlike systems, the French team uses a particularly short-lived excitation of the quantum well, the plasmon, as the fundamental oscillator. Because of its short lifetime, the plasmon has a broad resonance, allowing coupling to occur between different plasmons and superradiance to arise.
A quantum well is a thin layer of a semiconductor medium, sandwiched between other semiconductor materials to restrict the movement of charges (electrons or holes) in the dimension perpendicular to the layer. When a current flows through a conventional quantum well LED, electrons and holes drop into the “well” and recombine, sending out light. But the dipoles of such electron-hole pairs are not the only means of generating light. When charges are added to the quantum well chemically by doping, a further type of electromagnetically active dipole can arise: the plasmon [8]. Plasmons are oscillations of mobile electrons around the fixed positive ions of a material and are well known from metals. Plasmons in a quantum well differ somewhat from those in metals because they involve transitions between the discrete energy levels of the nanostructure rather than the continuous sea of charges in a metal. And quantum well plasmons can be excited by passing a current. Such a “plasmon LED” emits light in a fairly narrow band of the IR, and it is the device that Sirtori and colleagues developed in their study.
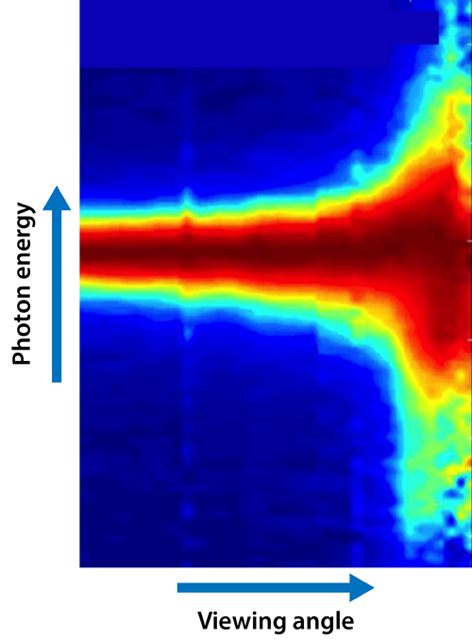
Plasmons contain many electrons oscillating at once, in phase, and are therefore described by a giant dipole. The larger the dipole, the faster light is emitted—the broader the resonance of the underlying oscillator. In a semiconductor nanostructure, different plasmons exist. These constitute the fundamental oscillators in the Dicke effect. Sirtori and co-workers realized that the effect of disorder between oscillators (the variation in resonance frequencies) is overcome by the large resonance width, enabling plasmon superradiance in the IR spectral region. The result is radiation from coupled plasmons with extremely short lifetimes, approaching 1 femtosecond. This lifetime is determined experimentally by the spectral width of the light generated by the plasmons (Fig. 1).
Plasmons are unlikely candidates for atomlike transitions to explore fundamental quantum-optical phenomena. They are smeared-out quasiparticles with broad resonances, quite the opposite of discrete atoms. Although plasmons are known to show all kinds of exotic interference phenomena [9, 10], Sirtori and colleagues’ work underlines that, in some circumstances, the behavior of plasmons branches out into the realm of quantum optics. Potential applications of the plasmonic Dicke effect include ultrafast solid-state IR radiation sources. Such sources are needed for IR spectroscopy and molecular fingerprinting to selectively identify molecules and molecular complexes through their distinct atomic vibrations. Many conventional IR sources rely on thermal black-body radiation, which is spectrally much broader than molecular vibrations and hard to generate in temporally compressed pulses. Finally, since plasmons involve mobile electrons, which always lose energy through Ohmic heating, they are usually not used to generate light directly in optoelectronic devices. Plasmon superradiance, however, changes the game now, since light emission can occur before heating sets in. Sirtori and co-workers’ work could well herald a whole new field of plasmon-based optoelectronics.
This research is published in Physical Review Letters.
References
- R. H. Dicke, “Coherence in Spontaneous Radiation Processes,” Phys. Rev. 93, 99 (1954).
- M. Gross and S. Haroche, “Super-radiance: An Essay on the Theory of Collective Spontaneous Emission,” Phys. Rep. 93, 301 (1982).
- T. Laurent, Y. Todorov, A. Vasanelli, A. Delteil, C. Sirtori, I. Sagnes, and G. Beaudoin, “Superradiant Emission from a Collective Excitation in a Semiconductor,” Phys. Rev. Lett. 115, 187402 (2015).
- R. G. DeVoe and R. G. Brewer, “Observation of Superradiant and Subradiant Spontaneous Emission of Two Trapped Ions,” Phys. Rev. Lett. 76, 2049 (1996).
- M. Scheibner, T. Schmidt, L. Worschech, A. Forchel, G. Bacher, T. Passow, and D. Hommel, “Superradiance of Quantum Dots,” Nature Phys 3, 106 (2007).
- J. A. Mlynek, A. A. Abdumalikov, C. Eichler, and A. Wallraff, “Observation of Dicke Superradiance for Two Artificial Atoms in a Cavity with High Decay Rate,” Nat. Commun. 5, 5186 (2014).
- D. P. Waters, G. Joshi, M. Kavand, M. E. Limes, H. Malissa, P. L. Burn, J. M. Lupton, and C. Boehme, “The Spin-Dicke Effect in OLED Magnetoresistance,” Nature Phys. (2015).
- A. Delteil, A. Vasanelli, Y. Todorov, C. Feuillet Palma, M. Renaudat St-Jean, G. Beaudoin, I. Sagnes, and C. Sirtori, “Charge-Induced Coherence between Intersubband Plasmons in a Quantum Structure,” Phys. Rev. Lett. 109, 246808 (2012).
- A. Christ, S. G. Tikhodeev, N. A. Gippius, J. Kuhl, and H. Giessen, “Waveguide-Plasmon Polaritons: Strong Coupling of Photonic and Electronic Resonances in a Metallic Photonic Crystal Slab,” Phys. Rev. Lett. 91, 183901 (2003).
- N. Verellen, Y. Sonnefraud, H. Sobhani, F. Hao, V. V. Moshchalkov, P. Van Dorpe, P. Nordlander, and S. A. Maier, “Fano Resonances in Individual Coherent Plasmonic Nanocavities,” Nano Lett. 9, 1663 (2009).