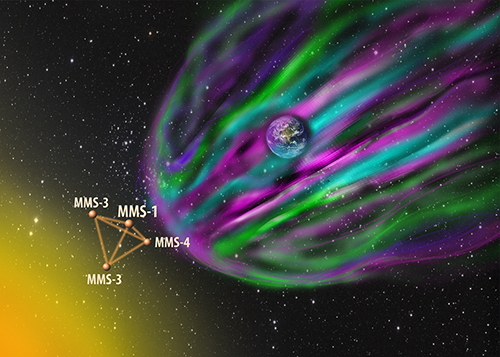
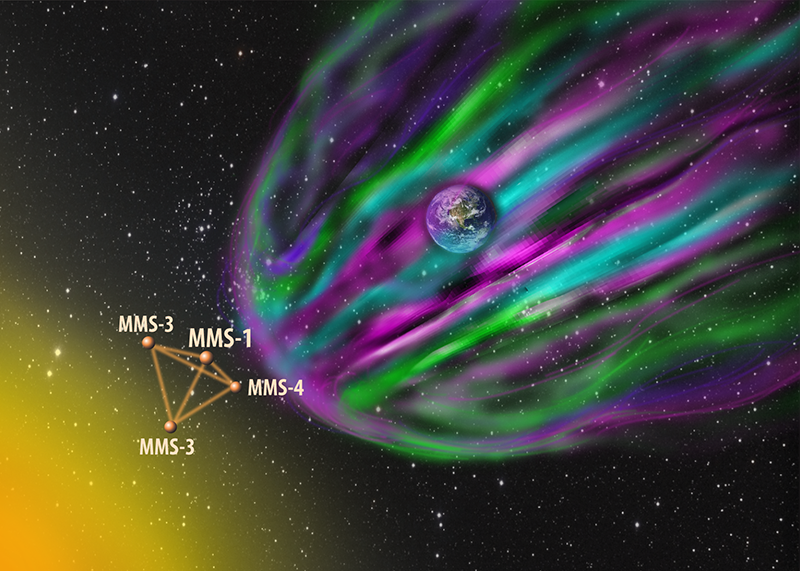
Inside a Plasma Shock
One of the dominant sources of cosmic rays—charged particles from space whose energies can exceed —are shockwaves in space plasma. These supersonic waves are produced by supernovae and other explosive phenomena in the Universe [1]. However, a microscopic picture of how a shockwave dissipates its energy, accelerating electrons and ions in the process, remains unclear. Using data from NASA's Magnetospheric Multiscale (MMS) mission (Fig. 1), which orbits Earth near the outskirts of its magnetosphere, Andreas Johlander of the Swedish Institute of Space Physics [2] and colleagues have characterized with unprecedented temporal resolution the wavelike flux of ions that carry energy away from a plasma shock. Their analysis provides one of the most detailed views of a shock’s internal structure to date, offering a unique opportunity to test the results of space plasma simulations and to better understand a key mechanism of cosmic-ray production.
Hot and dilute space plasma like that studied by Johlander et al. is often termed collisionless plasma because its constituent particles do not exchange energy or momentum through direct contact. As a result, collisionless plasmas tend to be far from thermal equilibrium. The rarity of collisions in such plasmas is what allows their constituent particles to propagate for a long time without losing energy. However, it does not mean that energy transfer between particles is completely shut off. Rather, charged particles in a collisionless plasma interact with each other through electromagnetic fields, which are generated by the collective motions of the particles themselves. This collective effect is complicated, introducing a variety of interesting dynamic phenomena. Most notably, it allows a small number of particles to be accelerated to enormous energies, far beyond the reach of humanmade particle accelerators.
This selective acceleration is what occurs when a shockwave, or shock for short, is generated in a collisionless plasma. This supersonic wave could form when, for example, a high-speed plasma created by a supernova explosion interacts with the surrounding medium. But unlike the shockwave from an airplane traveling at Mach speed, which dissipates its kinetic energy into heat through molecular collisions, a shockwave in a collisionless plasma (a collisionless shock) involves a more complicated dissipation mechanism. The energy is divided up between the plasma constitutents, with some going to ions, some to electrons, and a fraction to the generation of magnetic fields [3]. Formulating a clear picture of how a collisionless shock’s energy is converted to other forms of energy is crucial for a reliable estimate of the cosmic-ray production rate. However, parsing out where the energy goes is far from trivial because energy dissipation is controlled by collective plasma processes, which are highly nonlinear and occur on many length and time scales.
Theoretical and experimental work so far has shown that most of the energy in a shock is dissipated by reflected ions [4]. These are ions that are reflected by a compressed magnetic field or electrostatic potential in the shock. They make up only a small fraction of the ions (typically 10–20% in density), but they can carry away a relatively large fraction of energy. The reflection directly energizes the ions, which in turn excite plasma waves that heat the plasma upstream of the shock [5]. Researchers studying collisionless shocks have long recognized that ion reflection oscillates in time—that is, it is nonstationary. This can lead to new shock fronts in the upstream region, a process known as self-reformation [6]. However, the nonstationary ion reflection may also be associated with surface waves, or “ripples,” that propagate in the plane of the shock [7]. Researchers are interested in the form that nonstationarity takes (self-reformation or ripples) because it may influence the shock’s dissipation of energy [8, 9]. The in situ observation by Johlander et al. offers a rare chance to experimentally test these ideas, which have so far been based on numerical simulations or a limited number of observations and lab-based experiments.
MMS was launched on March 12, 2015. It consists of four identical spacecraft that orbit Earth as a group and carry detectors for measuring the velocities of nearby ions and electrons. The mission was designed to study the reconnection of magnetic field lines that occurs near Earth [10], another leading candidate for particle acceleration. However, the experiment can also, on occasion, study an event known as a bow shock. Such shocks are generated when the solar wind slams into Earth’s magnetic field, undergoing a sudden slowdown. Bow shocks occur outside Earth’s magnetosphere, and therefore beyond the orbit of MMS. But a fluctuation in the solar wind can temporarily compress the magnetosphere, leaving the MMS spacecraft in the path of a bow shock. This is what occurred on October 7, 2015, enabling the analysis by Johlander et al.
The team took advantage of two key features of MMS to determine the internal structure of a nonstationary shock in unprecedented detail. First, compared to previous spacecraft, MMS achieves an order of magnitude greater temporal resolution in its measurement of ion velocities. Second, the distance between the four spacecraft was only about 25 km at the time of observation. As a result, MMS could measure the ion velocities at multiple points within the plasma, allowing Johlander et al. to determine whether the observed profile arises from temporal or spatial variations. The European Cluster II mission also has four spacecraft and has successfully performed multipoint measurements. But the distances between its spacecraft (about 10 times that of MMS) are too large to quantify the small-scale nonstationarity of a shock. Indeed, the short wavelength (175 km) and fast period (1.7 s) of the nonstationary oscillations analyzed by Johlander et al. could not have been determined by any spacecraft preceding MMS. By comparing the time-varying shock profiles measured at the four spacecraft, the authors concluded that the nonstationary reflection they observed involved ripples propagating in the shock plane rather than self-reformation. This is the first clear identification of ripples in space plasma.
For now, it’s unclear whether the ripples they have identified are a generic feature of shocks, or simply a special case; there may be situations where self-reformation dominates over the ripples. Figuring this out would require statistical surveys of many more shock-crossing events. Ultimately, researchers need to understand the coupling between nonstationary ion reflection and plasma wave generation, and the associated energization of charged particles. With its improved temporal resolution, the MMS data will allow researchers to investigate this coupling more fully. Long term, we can expect that the insights gained from MMS measurements will help us understand astrophysical cosmic-ray accelerators.
This research is published in Physical Review Letters.
Correction (13 October 2016): An earlier version of the article stated that Andreas Johlander was at Uppsala University. He is in fact employed by the Swedish Institute of Space Physics.
References
- R. Blandford and D. Eichler, “Particle Acceleration at Astrophysical Shocks: A Theory of Cosmic Ray Origin,” Phys. Rep. 154, 1 (1987).
- A. Johlander et al., “Rippled Quasiperpendicular Shock Observed by the Magnetospheric Multiscale Spacecraft,” Phys. Rev. Lett. 117, 165101 (2016).
- Y. Uchiyama, F. A. Aharonian, T. Tanaka, T. Takahashi, and Y. Maeda, “Extremely Fast Acceleration of Cosmic Rays in a Supernova Remnant,” Nature 449, 576 (2007).
- M. M. Leroy, D. Winske, C. C. Goodrich, C. S. Wu, and K. Papadopoulos, “The Structure of Perpendicular Bow Shocks,” J. Geophys. Res. 87, 5081 (1982).
- C.S. Wu, Winske, Y. M. Zhou, S. T. Tsai, P. Rodriguez, M. Tanaka, K. Papadopoulos, K. Akimoto, C. S. Lin, M. M. Leroy, and C. C. Goodrich, “Microinstabilities Associated with a High Mach Number, Perpendicular Bow Shock,” Space Sci. Rev. 37, 63 (1984).
- D. Biskamp, “Collisionless Shock Waves in Plasmas,” Nucl. Fusion 13, 719 (1973).
- R. E. Lowe and D. Burgess, “The Properties and Causes of Rippling in Quasi-perpendicular Collisionless Shock Fronts,” Ann. Geophys. 21, 671 (2003).
- N. Shimada and M. Hoshino, “Effect of Strong Thermalization on Shock Dynamical Behavior,” J. Geophys. Res. Space Phys. 110, A02156 (2005).
- M. Scholer and S. Matsukiyo, “Nonstationarity of Quasi-perpendicular Shocks: a Comparison of Full Particle Simulations with Different Ion to Electron Mass Ratio,” Ann. Geophys. 22, 2345 (2004).
- J. L. Burch et al., “Electron-Scale Measurements of Magnetic Reconnection in Space,” Science 352, 2939 (2016).