Superfluids Hit the Street
The von Kármán vortex street is a classical flow pattern of swirling vortices, arising in clouds, streams, and rivers. Yong-il Shin from Seoul National University, Korea, and colleagues have now created its quantum analog in an ultracold gaseous superfluid [1], marking a major step in unraveling the deep connections between these extraordinary fluids and their everyday counterparts.
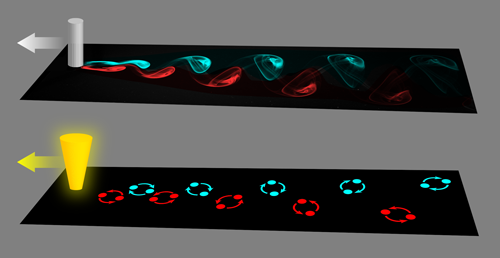
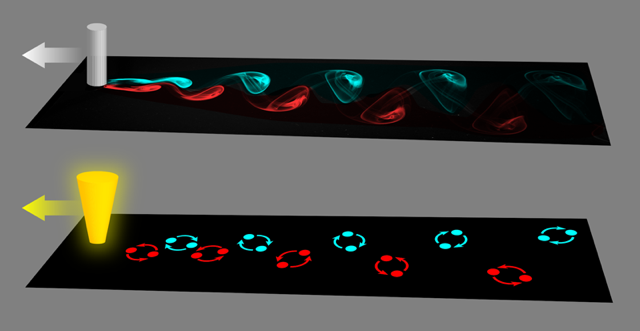
Ordinary viscous fluids, such as air or water, give rise to a plethora of stunning flow patterns [2], which have captured our scientific curiosity since Leonardo da Vinci’s pioneering studies of fluid motion. The textbook example is incompressible fluid flow past a cylinder, whose distinct regimes are captured by a single parameter, the Reynolds number Re =vd∕𝜈, where v is the flow velocity, d is the cylinder's diameter, and 𝜈 is the fluid's viscosity. For low Re (Re ), the flow pattern is steady and regular, preserving a number of underlying symmetries in space and time. For high Re (Re ), the wake is an irregular, turbulent mess of swirls and waves, in which these symmetries are completely broken [3]. Within this transition region, and occupying a huge range of Re, is the von Kármán vortex street. Here, vortices peel alternatingly off each side of the cylinder to form a downstream pattern of staggered vortex “footprints,” marking a dramatic reduction in the symmetry of the flow (Fig. 1). Following observations by Arnulph Mallock and Henri Bénard in the early 1900s, Theodore von Kármán’s 1911 seminal analysis [4] showed that only such a staggered configuration of vortices was stable. The von Kármán vortex street is a spectacular display of fluid motion in everyday fluid flows. But it also belies a dangerous side: the alternating vortex shedding can drive vibrations with the power to destroy towers, wings, and bridge supports, if not properly accounted for.
Looking for this vortex pattern in superfluids is one way physicists can gain a deeper understanding of the relationship between classical and quantum fluids. Superfluids have been engineered under several guises—liquid helium below 2.2 K, atomic gases at nanokelvin temperatures, and light-matter systems at up to room temperature. In all of these systems, quantum mechanics emerges on a macroscopic scale and gives rise to their remarkable properties. A superfluid has zero viscosity and can flow forever once set into motion. However, Lev Landau’s famous criterion tells us that if the flow exceeds a critical speed, the superfluidity breaks down: excitations (here in the form of vortices) are created in the fluid and dissipate energy from the flow, like an effective viscosity. Moreover, the circulation of the fluid is quantized, meaning that any vortices in the superfluid are restricted to exist as tiny whirlpools of fixed size and strength, unlike in an ordinary fluid, where they can have any size and circulation.
These intrinsic differences between superfluids and conventional fluids raise fundamental questions about how they differ when they flow. Here, the cylinder paradigm provides a natural scenario in which to address these questions. For low flow speed, the superfluid undergoes steady, smooth flow; remarkably, it exerts zero drag on the cylinder, setting it apart from ordinary laminar flow. Above the critical speed, pairs of quantum vortices of opposite circulation—vortex dipoles—are shed simultaneously and carried downstream, as first predicted by pioneering simulations in 1992 [5] and confirmed experimentally in atomic superfluids since 2010 [6, 7]. Although a regime analogous to that of a von Kármán vortex street has been proposed for superfluids exceeding this critical speed [8–10], its experimental confirmation has been elusive until now.
In their study, Shin and co-workers prepared an atomic Bose-Einstein condensate—a quantum gas that behaves like a compressible superfluid—of around five million sodium atoms, 200 m across and at around 200 nanokelvin. They used a laser beam to punch a circular hole 10 m wide into the gas, mimicking a cylindrical obstacle, and dragged this cylinder through the gas at a speed fast enough for the gas to shed quantum vortices each about 1 m across. They then photographed the gas to reveal its density profile, after expanding it briefly under free fall to make the tiny vortices visible. Surprisingly, the authors observed large density holes, which they attributed to closely positioned vortices blurring into one during the condensate’s expansion. The finding indicates that the quantum vortices occur in clusters. Indeed, the frequency distribution of the number of vortices per cluster, inferred from the area of each density hole, was clearly peaked at integer values, representing from one to five vortices per cluster.
To build a more detailed picture, Shin and co-workers repeated the experimental procedure for different speeds of the dragged cylinder. For low speeds, they found that the vortices were shed individually from each side of the cylinder, forming vortex dipoles downstream [6, 7]. At higher speeds, two-vortex clusters were emitted in a periodic, staggered manner—this was the signature of the quantum analog of the von Kármán vortex street. And at even higher speed, larger clusters also formed, the shedding rate increased, and the emission became highly irregular, marking the transition to turbulent shedding.
The researchers were able to explore the von Kármán regime, which occurs over a narrow speed range [8, 10], by finely controlling the cylinder’s speed and employing a favorable beam shape. Direct observation of the vortex street was hampered by the small extent of the fluid, whose boundaries deflected the vortex trajectories downstream. Nonetheless, by switching to a narrower laser beam, the authors observed a striking trail of several staggered two-vortex clusters, evocative of the classic von Kármán street.
These observations help to complete the picture of how a superfluid flows past a cylinder. Like for ordinary fluids, this paradigm may provide a conceptual basis for a much wider range of flow scenarios. Along these lines, it is important to address whether the superfluid flow regimes are universal and how to parametrize them, given that the ordinary Reynolds number is no longer inapplicable. For a recently proposed “superfluid Reynolds number” [10], the transition to turbulence is suggestively close to that observed here, despite being based on an infinite-sized system. Moreover, the Strouhal number, a universal parameter representing the rate of vortex shedding, was inferred by Shin and colleagues to saturate to a value close to that of ordinary von Kármán flow. This developing picture points to a deep connection to ordinary flows. Indeed, on a microscopic scale, the shedding of quantum vortices in clusters may be intuitively interpreted as the superfluid's way of replicating ordinary flow, recovering the classical behavior for a large number of quanta [9]. Future experiments of this kind will be crucial to further unravel this intriguing interface between superfluids and ordinary fluids.
This research is published in Physical Review Letters.
References
- W. J. Kwon, J. H. Kim, S. W. Seo, and Y. Shin, “Observation of von Kármán Vortex Street in an Atomic Superfluid Gas,” Phys. Rev. Lett. 117, 245301 (2016).
- M. Van Dyke, An Album of Fluid Motion (Parabolic Press, Stanford, 1982)[Amazon][WorldCat].
- U. Frisch, Turbulence: The Legacy of A. N. Kolmogorov (Cambridge University Press, Cambridge, 1995)[Amazon][WorldCat].
- Th. von Kármán, Aerodynamics: Selected Topics in the Light of Their Historical Development (Cornell University Press, Ithaca, 1954)[Amazon][WorldCat].
- T. Frisch, Y. Pomeau, and S. Rica, “Transition to Dissipation in a Model of Superflow,” Phys. Rev. Lett. 69, 1644 (1992).
- T. W. Neely, E. C. Samson, A. S. Bradley, M. J. Davis, and B. P. Anderson, “Observation of Vortex Dipoles in an Oblate Bose-Einstein Condensate,” Phys. Rev. Lett. 104, 16040 (2010).
- W. J. Kwon, S. W. Seo, and Y. Shin, “Periodic Shedding of Vortex Dipoles from a Moving Penetrable Obstacle in a Bose-Einstein Condensate,” Phys. Rev. A 92, 033613 (2015).
- K. Sasaki, N. Suzuki, and H. Saito, “Bénard–von Kármán Vortex Street in a Bose-Einstein Condensate,” Phys. Rev. Lett. 104, 150404 (2010).
- G. W. Stagg, N. G. Parker, and C. F. Barenghi, “Quantum Analogues of Classical Wakes in Bose–Einstein Condensates,” J. Phys. B 47, 095304 (2014).
- M. T. Reeves, T. P. Billam, B. P. Anderson, and A. S. Bradley, “Identifying a Superfluid Reynolds Number via Dynamical Similarity,” Phys. Rev. Lett. 114, 155302 (2015).