Balls as 3D Gears
If you take a large collection of balls in contact and force some of them to spin, they can behave like a set of gears, with each ball rotating without slipping. Experiments and theory show that this synchronized motion can be predicted even when the initial motion involves seemingly random slipping between the spheres (like the skipping of gears). The researchers say that the ability to predict this collective motion could lead to three-dimensional gear systems in which manipulations of one or two spheres allows complete control of the rotational motion of the others.
The original motivation for studying large assemblies of different-sized spheres has been to model granular materials, such as the rocks and gravel that collect in the gaps between tectonic plates. Researchers want to understand how these packed grains are able to rotate in unison, since this rotation can affect the friction that keeps two tectonic plates from shifting.
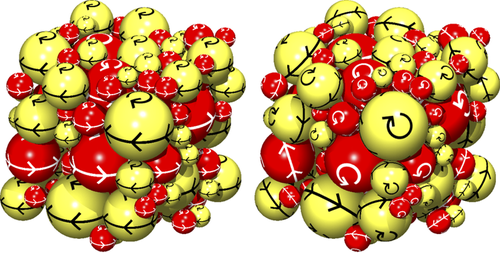
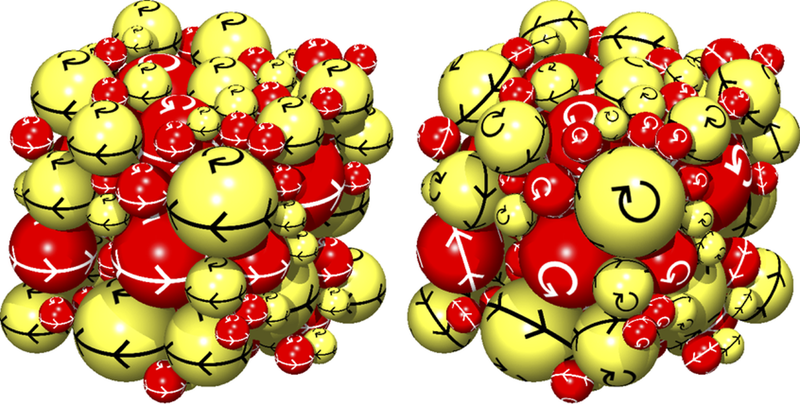
Previous studies have looked at special configurations in which every sphere touches at least two others, and these contacts form interconnected, closed loops with even numbers of spheres [1]. Such an assembly is called bichromatic because you can paint each sphere one of two colors, so that no two spheres of the same color touch. The spheres’ centers are fixed, but they can rotate freely around any axis. What is surprising is that—no matter the number of spheres or the complexity of the packing—there is always a set of rotations for which every sphere rotates without slipping at its contact points.
In their study, Dominik Stäger of the Swiss Federal Institute of Technology (ETH) in Zürich and his colleagues wanted to understand how bichromatic assemblies coordinate themselves when some of the spheres are forced to move by, for example, the sliding of a tectonic plate or the motion of a drive wheel. At the start, the spheres will slip, but they will eventually adjust their rotations, settling into a no-slip state where energy losses due to friction are minimized, Stäger explains. Predicting this final, no-slip configuration might seem hopeless, as the initial slipping motion depends on a multitude of factors including the slipperiness of each sphere.
The researchers first analyzed these systems mathematically and identified special sums of rotational variables whose time-independence imply that the no-slip configuration can be predicted for certain bichromatic systems. These predictable systems, in their no-slip condition, have four degrees of freedom (4DOF)—meaning that four spin or orientation parameters of specific spheres determine the final rotational behavior of all of the others.
Arrangements of spheres that have 4DOF can be systematically constructed in a simple way with an arbitrary number of spheres. For a general 4DOF assembly, the team found that the final no-slip state takes one of two forms: either all of the rotational axes are parallel, or all of the axes intersect at a single point.
The predictability of 4DOF assemblies implies that they are controllable, assuming one selects an appropriate set of four input parameters. As a demonstration of this control, the team formed a stack of four plastic balls and controlled the two balls at the bottom with motorized wheels. After some initial slipping, the top balls quickly synchronized with the bottom balls.
In agreement with the team’s predictions, turning the bottom balls in opposite directions caused the top balls to rotate at the driving speed, whereas having the bottom balls turn in the same direction made the top balls rotate at around twice the driving speed. In an experiment with six balls, the top balls rotated as much as 3 times faster. This “amplification” means that the balls can work like gears to transmit power, Stäger says. The system also offers control over the orientation of the rotational axes of the top balls. Stäger and his colleagues imagine this sort of three-dimensional gear system could eventually be useful in a robotic arm or some other mechanical machine.
Tomaso Aste from University College London finds this paper “very intriguing, simple, yet unexpected and rich with implications.” He says the fact that some assemblies can rotate without slipping was known before, “but the capability to predict and control the rotation of an assembly of spheres is unexpected.” He’s fascinated by the possibility of new mechanical devices based on this mechanism.
This research is published in Physical Review Letters.
–Michael Schirber
Michael Schirber is a Corresponding Editor for Physics Magazine based in Lyon, France.
References
- R. Mahmoodi Baram, H. J. Herrmann, and N. Rivier, “Space-Filling Bearings in Three Dimensions,” Phys. Rev. Lett. 92, 044301 (2004).