Dancing the Bose-nova with a twirl

Maciej Lewenstein

Viewpoint

Dancing the Bose-nova with a twirl

Institut de Ciències Fotòniques (ICFO), Parc Mediterani de la Tecnologia, 08860 Castelldefels, Spain and Institució Catalana de Recerca i Estudis Avançats (ICREA), 08010 Barcelona, Spain

August 18, 2008• Physics 1, 13

A Bose-Einstein condensate (BEC) can dramatically collapse and explode when the interactions between the atoms are sufficiently strong and attractive. Now, scientists have imaged the anisotropic, clover-leaf shape of such a collapsing gas when the attractive atomic interactions are strongly dipolar.

Figure caption — **Figure 1:** A series of images showing a dipolar condensate as it explodes after the collapse. From left to right, the images correspond to increasing times over which the BEC is held in the trap after the atomic interactions are tuned with the Feshbach resonance (the image at the far left corresponds to a holding time of $0.1 ms$ ; the one in the foreground to a holding time of $0.5 ms$ ; successive images are separated by $0.1 ms$ ). The anisotropic dipolar interaction between the atoms leads to an explosion with the same $d$ -wave symmetry.

Interactions between “elementary” objects, such as electric charges, are typically characterized by a high degree of rotational or translational symmetry. The same is usually not true for interactions between “composite” objects, such as magnetic or electric dipoles and ensembles of interacting dipoles can have quite complex behavior. In classical physics, for example, dipole interactions are responsible for the fascinating properties of colloidal suspensions of magnetic nanoparticles known as ferrofluids [1]. The viscosity of these liquids can be tuned with an external magnetic field—a property that some high-end car manufacturers have even put to use in the design of self-adjusting suspension systems.

Though they are unlikely to appear in an automobile any time soon, analogous effects can appear in a quantum degenerate gas—or Bose-Einstein condensate (BEC)—of atoms with large magnetic dipoles [2]. In the current issue of Physical Review Letters, a collaboration between Thierry Lahaye, Tilman Pfau, and coworkers at the Universität Stuttgart [3] and colleagues in Japan demonstrates a very spectacular effect when dipole interactions are present in a BEC of $^{52} Cr$ atoms. The group studies the gas in a regime where the attractive interactions are sufficiently strong that the BEC collapses. By carefully tuning the strength of the dipolar interactions and the trap that confines them, they show that the anisotropy of the collapsing gas reflects the symmetry of the underlying magnetic dipole-dipole interactions between the chromium atoms. The measurements and supporting calculations provide an important test, performed in extreme conditions, of whether the models that currently describe quantum gases where the underlying interactions are isotropic can be extended to dipolar gases.

In 1999, Randy Hulet’s group at Rice University showed [4] that a BEC with rotationally symmetric, attractive van der Waals interactions would first collapse on itself and then explode (a phenomenon whose likeness to supernova gave rise to the term “Bose-nova” [5]). The evolution is characterized by an isotropic implosion of the BEC, which is ultimately slowed down by three-body losses. The collapsing gas consists of three components: a remnant condensate that consists of multiple solitons [6], a burst of energetic atoms that are ejected from the condensate, and a certain fraction of atoms that escape due to energy losses in three-body collisions.

A similar Bose-nova in a dipolar gas has not been seen so far, but there has been significant progress in this direction. Theorists predicted quite early [7] that the physics of ultracold trapped Bose gases with magnetic dipole-dipole interactions between the atoms would depend crucially on the geometry of the trap that confines them. When two vertically polarized dipoles are stacked head to tail, they will attract each other. If they are located side by side, they will repel. It follows that cigar-shaped vertical traps favor the collapse of the gas because attractive forces dominate, while repulsive forces in a pancake-shaped horizontal trap stabilize the gas, and that by tuning the shape of the trap, it is possible to move between the stable and instable regimes [2,7,8].

To prepare the BEC of $^{52} Cr$ atoms—the only BEC gas with dipole-dipole interactions that can be stabilized so far—the team combine numerous techniques and tricks that they and others in the field of ultracold atom physics have developed, such as forced evaporative cooling of the atoms within a crossed optical dipole trap. In particular, the Pfau group had to find a way to reduce electric dipole-dipole (van der Waals) forces between the atoms. Although the atoms of $^{52} Cr$ have quite large magnetic dipoles, the repulsive van der Waals forces can completely dominate the attractive magnetic dipole-dipole interactions, even in the cigar-shaped trap geometry. In earlier work, the authors figured out how to greatly reduce the effect of van der Waals forces [8]. When two ultraslow atoms collide elastically, the collision typically produces a small phase shift in the scattered atoms’ wave functions, proportional to the so-called scattering length. If, however, the colliding atoms have an energy that is resonant with an excited molecular bound state (a Feshbach resonance), the value of the scattering length can be dramatically modified, and even reduced to zero. Although such miracles hardly occur by themselves, one can bring them into existence by applying a magnetic field that shifts the energy of the excited bound molecular state. In this way, the Stuttgart group was able to reduce the strength of van der Waals interactions to a level comparable with the dipolar ones [8].

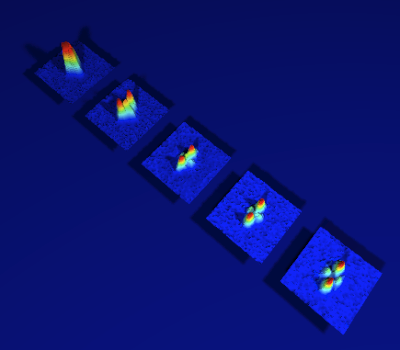
Prior to Lahaye et al.’s experiments, earlier theoretical work [9] indicated that a collapsing dipolar gas would not have rotational symmetry. The simplest way to analyze the collapse is to determine the condensate wave function and then look at small fluctuations around the condensate state, taking into account the dipolar interactions [10]. If small fluctuations grow exponentially then the gas is unstable to collapse, and the instability occurs when the fluctuations, in the form of phonons, have modes that have $d$ -wave symmetry. In the present paper, the authors go far beyond this analysis to describe the time dependent BEC wave function in three dimensions with fluctuations and accompanied by three-body losses.

The collapse observed by Lahaye et al. indeed exhibits beautiful $d$ -wave symmetry. Figure 1 shows the signature clover-leaf shape, which agrees with the theoretical results without any fitted parameters. The theory predicts one intriguing effect that has so far not been detected. Because of the anisotropy of the dipolar interaction, the BEC may start to explode radially while it is still imploding axially, giving rise to vortex rings. In contrast to the Bose-nova that have been observed so far in $^{85} Rb$ experiments, the Bose-nova of atoms that have dipolar interactions is apparently being danced with a twirl.

The work by Lahaye et al. is an excellent experiment supported by a convincing theoretical analysis. But where will it lead? Observing the predicted vortex or soliton-like structures would be particularly interesting. Another possibility that would have a strong connection to condensed matter physics would be to prepare dipolar gases that are confined in two dimensions and to apply a centrifugal force to the atoms. Analogous to the quantized orbits that form in a two-dimensional electron gas in a strong magnetic field (Landau levels), such a gas would form quantized rotating states. For clouds of atoms interacting via van der Waals forces, it may be possible to obtain analogues of the fractional quantum Hall state and Laughlin liquid. It has been predicted that a small admixture of dipolar interactions in such systems would lead to other analogues of exotic, strongly correlated quantum liquids (in particular, those with non-Abelian anyonic excitations [11]). It is always difficult to predict exactly which avenue the study of quantum dipolar gases will follow in the near future. One can be sure though that it will be full of explosive discoveries.

References

S. Odenbach Magnetoviscous Effect in Ferrofluids (Lecture Notes in Physics Monographs) (Springer, Heidelberg, 2007) [Amazon][WorldCat]
J. Stuhler, A. Griesmaier, T. Koch, M. Fattori, T. Pfau, S. Giovanazzi, P. Pedri, and L. Santos, Phys. Rev. Lett. 95, 150406 (2005)
T. Lahaye, J. Metz, B. Fröhlich, T. Koch, M. Meister, A. Griesmaier, T. Pfau, H. Saito, Y. Kawaguchi, and M. Ueda, Phys. Rev. Lett. 101, 080401 (2008)
C. A. Sackett, J. M. Gerton, M. Welling, and R. G. Hulet, Phys. Rev. Lett. 82, 876 (1999)
E. A. Donley, N. R. Claussen, S. L. Cornish, J. L. Roberts, E. A. Cornell, and C. E. Wieman, Nature 412, 295 (2001)
K. E. Strecker, G. B. Partridge, A. G. Truscott, and R. G. Hulet, Nature 417, 150 (2002)
L. Santos, G. V. Shlyapnikov, P. Zoller, and M. Lewenstein, Phys. Rev. Lett. 85, 1791 (2000); 88, 139904(E) (2002)
T. Lahaye, T. Koch, B. Fröhlich, M. Fattori, J. Metz, A. Griesmaier, S. Giovanazzi, and T. Pfau, Nature 448, 672 (2007)
K. Góral and L. Santos, Phys. Rev. A 66, 023613 (2002)
L. Santos, G. V. Shlyapnikov, and M. Lewenstein, Phys. Rev. Lett. 90, 250403 (2003)
E. H. Rezayi, N. Read, and N. R. Cooper, Phys. Rev. Lett. 95, 160404 (2005)

About the Author

Maciej Lewenstein graduated from Warsaw University and completed his Ph.D. at the University of Essen. He was a faculty member of the Centre for Theoretical Physics of the Polish Academy of Sciences until 1995, and then joined the Service des Photons, Atomes et Molécules of the Commissariat à l’Énergie Atomique (CEA) in France. In 1998 he moved to the University of Hanover, and since 2005 he has been the head of the quantum optics theory group at the Institute of Photonic Sciences (ICFO) in Castelldefels, Spain, and a Professor at the Catalan Institution for Research and Advanced Studies (ICREA). His interests include the quantum physics of cold atoms and other many-body systems, quantum information theory and mathematical physics and attosecond physics. He is an APS Fellow and a recipient of both the Humboldt Research Award and the European Research Council Advanced Grant.