Pile on the metal

Dung-Hai Lee

Viewpoint

Pile on the metal

Department of Physics, University of California, Berkeley, Berkeley CA 94720-7300, USA

September 15, 2008• Physics 1, 19

Discovering superconductivity above room temperature is a dream for modern science and technology. Now, theorists propose that for certain types of superconductors, contact with a metal layer could greatly increase the transition temperatures of these materials—in some cases by as much as an order of magnitude.

Figure caption — **Figure 1:** An example of how coupling a superconductor to a metal can enhance superconductivity. The left panel shows a Josephson junction array: a set of superconducting islands (gold squares) that are separated by insulating material (blue substrate). When the energy to add or remove one Cooper pair (two bound electrons) on a single island is large compared to the energy gained by allowing the electrons to move throughout the entire array, the Cooper pair wave functions (gray curves) are localized and do not have a well defined phase. Capacitively coupling the array to a metal (right panel) damps the fluctuations in the Cooper pair wave functions and they condense into a single superconducting state.

Designing ways to raise the superconducting transition temperature ( $T_{c}$ ) has always been an important goal of condensed matter research. In the past twenty years, two families of superconducting materials with transition temperature above 50 K have been discovered: the cuprates [1] and more recently, the iron-pnictides [2]. Many believe that some cuprate compounds should be very high temperature superconductors (that is, with a $T_{c} \sim 200 K$ ) were it not for the fact that the superconducting carriers, the Cooper pairs, have a low mobility [3]. Writing in Physical Review B, Erez Berg and Steve Kivelson of Stanford University and Dror Orgad of The Hebrew University in Jerusalem turn this logic around and suggest that making contact between a nominally low-mobility superconductor and a high-mobility metal will increase the mobility of Cooper pairs in the superconductor and raise $T_{c}$ [4].

Several factors determine how a normal metal behaves at low temperatures: the electron density, the fact that electrons obey Fermi statistics, and the electron spin and charge. The Bardeen-Cooper-Schrieffer (BCS) theory of superconductivity [5] and the modern interpretation of it [6], however, say this is not the complete story: the metallic state is “marginally unstable” with respect to the weakest attractive electron-electron interaction. In the presence of such an interaction, Cooper pairs with charge $2 e$ form and condense into a charged boson superfluid at low temperatures. The resulting state is superconducting, i.e., it exhibits dissipationless electrical conduction and repels magnetic flux.

At this point, readers may wonder why electrons attract instead of repel one another. In fact, how to generate the effective attractive interaction between electrons in a superconductor is often referred to as the “mechanism question” for superconductivity. In the BCS theory, the effective interaction comes from lattice vibrations (phonons), but in cuprates and pnictides, the mechanism remains unknown.

Even without knowing the mechanism, one can develop models based on two quantities: $Δ_{0}$ , which is the strength of the attractive interaction, and $ρ_{s}$ , which is known as the phase stiffness. In a two-dimensional superconductor, $ρ_{s}$ is proportional to the difference in the kinetic energy between a maximally localized Cooper pair (a high kinetic energy) and a Bose condensed Cooper pair (a low kinetic energy). The weaker of the two of these parameters determines the $T_{c}$ of a superconducting material. One way to think of this is that there is a certain energy scale for forming the Cooper pairs (bosons) and another energy scale that causes them to condense into a single phase (the superfluid). For conventional superconductors, $ρ_{s}$ exceeds $Δ_{0}$ and so once the temperature drops so that thermal fluctuations no longer destroy the pairing (i.e., $k_{B} T_{c} \propto Δ_{0}$ ) the superconducting state sets in. However, in many cuprate compounds, $ρ_{s} < Δ_{0}$ . In these materials, it is the pair condensation (i.e., the now bosonic Cooper pairs form a Bose condensate) rather than pair binding that limits $T_{c}$ , i.e., $T_{c} \propto ρ_{s}$ . Berg et al.’s proposal for increasing $T_{c}$ considers this second case, where the pairing is strong enough for the superconductor to have a very high transition temperature, but $T_{c}$ is lowered because the electron pairs do not easily condense.

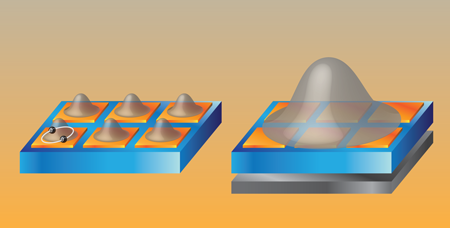
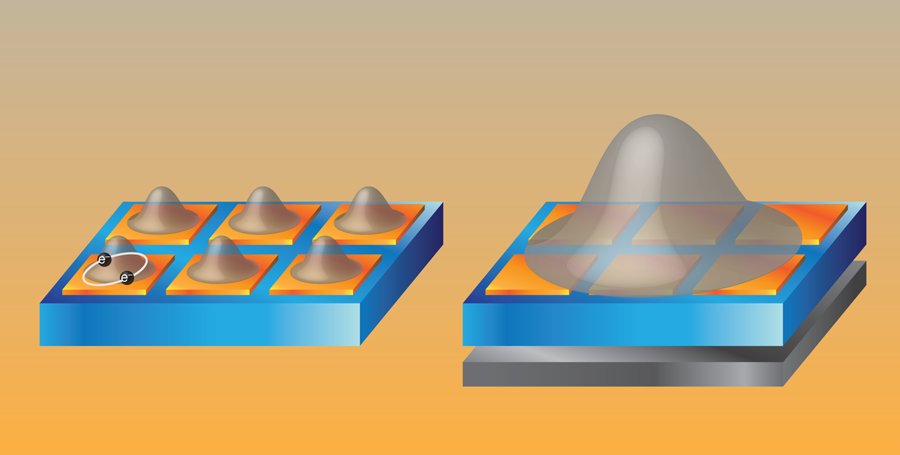
Specifically, Berg et al. consider a two-dimensional lattice where $Δ_{0}$ is the attraction between two electrons on the same site. To mimic the poor Cooper pair mobility, they either assume that the probability that electron pairs “hop” from site to site is zero, or the electron pairs can only hop in one direction. As constructed, this model exhibits finite $Δ_{0}$ but zero $ρ_{s}$ and cannot be superconducting—at least not in all directions—even at zero temperature. Since $T_{c}$ is set by $ρ_{s}$ , the trick is to find a way to increase $ρ_{s}$ by modifying the system so the electrons can move around more easily. Berg et al. therefore propose to put the two-dimensional lattice in contact with a normal metallic layer (Fig. 1). Electrons can now hop to the metal and $ρ_{s}$ increases. They demonstrate that for an appropriate choice of the electron transfer parameter between the two layers, the $T_{c}$ of the composite system can be raised to a substantial fraction of $Δ_{0} / k_{B}$ . Plugging in the numbers appropriate for the relevant cuprate compounds, this amounts to increasing $T_{c}$ from around 10 K to over 100 K, assuming the interfaces are “ideal” as in the model.

The fact that coupling a normal metal to a low $ρ_{s}$ superconductor can raise the $T_{c}$ actually has a close analogy to what is found in electronic devices called Josephson junction arrays (Fig. 1). A Josephson junction array consists of many superconducting islands connected by insulating materials. In such a system, the quantum tunneling of the Cooper pairs between neighboring islands eventually triggers superconductivity through the entire array. Once the superconducting island is smaller than a certain size, however, even a single extra Cooper pair would significantly raise the electrostatic energy (the so-called charging energy) of the island. When this extra charging energy overwhelms the kinetic-energy gain of allowing the Cooper pairs to spread out, the system becomes insulating. Under this condition the system has a finite $Δ_{0}$ (proportional to the bulk $T_{c}$ of the superconducting island) but zero $ρ_{s}$ —just as in the model from Berg et al.

It has been shown that coupling a Josephson junction array that is dominated by the charging energy to a nearby metal can restore superconductivity [7]. Moreover this can be achieved even when the coupling to the metal is purely capacitive (i.e., involving no electron transfer). The way this phenomenon is understood is as follows: In the limit of large charging energy, the number of Cooper pairs within individual islands stops fluctuating. In the opposite limit where the Cooper pair delocalization energy dominates, the Cooper pairs condense into a superfluid, which is equivalent to saying the phase of the Cooper pair wave functions lock together. It turns out that the number of Cooper pairs on an island and the phase of the Cooper pair wave function on the same island are “dual” variables to one another. In particular, when one variable is certain, the other is uncertain, exactly like the relation between the position and momentum of a particle in quantum mechanics. In classical mechanics, we know that static friction keeps a particle from moving. Analogously, the coupling between the metal and Josephson junction array introduces friction to the fluctuation of the phase of the Cooper pair wave function. As a result it helps lock-in the phase of the Cooper pairs and enhances their coherence into a superfluid.

As emphasized by Berg et al., allowing electron transfer between a low $ρ_{s}$ superconductor and a metallic layer has two opposite effects. It can increase the pair mobility (or damp the phase fluctuation) and enhance superconductivity. However, it also can decrease the pair binding strength $Δ_{0}$ , which is bad for superconductivity. The group takes effort to show that, within their model, there exists a range of parameters where the net effect of the metallic layer on superconductivity is positive. In the analogous case of the Josephson junction arrays, capacitive coupling damps phase fluctuations but, unlike electron transfer coupling, does not affect pair binding, so it would be interesting to consider capacitively coupling a normal metal to an “underdoped” cuprate superconductor.

It is possible that engineering interfaces (or a superlattice of interfaces) between high $Δ_{0}$ materials and high electron mobility materials along the line envisioned by Berg et al. is a worthy direction to try to raise the superconducting transition temperature. As suggested by the earlier discussions, to raise the $T_{c}$ of a superconductor it is best to cooperatively increase both $Δ_{0}$ and $ρ_{s}$ , while Berg et al.’s paper assumes a high pairing scale $Δ_{0}$ and focuses only on enhancing $ρ_{s}$ . Of course, figuring out how to increase $Δ_{0}$ also poses a challenging question. Research in cuprate superconductivity has yielded important ideas on this front. Among them, Anderson [8] argued that the antiferromagnetic correlations in many superconducting cuprates favors pairs of electron spins that form “singlets” (electron pairs with a total spin of zero). This creates an energy gap between spins in the singlet and the unpaired states, which is, roughly speaking, similar to $Δ_{0}$ . Though there is not yet a consensus on whether this is actually the pairing mechanism of the cuprate materials, like the paper by Berg, Orgad, and Kivelson, it is the type of idea that keeps the field of unconventional superconductivity—and the pursuit of a higher $T_{c}$ —moving forward.

References

J. G. Bednorz and K. A. Müller, Z. Phys. B - Con. Mat. 64, 189 (1986); M. K. Wu, J. R. Ashburn, C. J. Torng, P. H. Hor, R. L. Meng, L. Gao, Z. J. Huang, Y. Q. Wang, and C. W. Chu, Phys. Rev. Lett. 58, 908 (1987)
Y. Kamihara, H. Hiramatsu, M. Hirano, R. Kawamura, H. Yanagi, T. Kamiya, and H. Hosono, J. Am. Chem. Soc. 128, 10012 (2006); Z.-A. Ren, et al., arXiv:0804.2053
V. J. Emery and S. A. Kivelson, Nature 374, 434 (1995)
E. Berg, D. Orgad, and S. A. Kivelson, Phys. Rev. B 78, 094509 (2008)
J. Bardeen, L. N. Cooper, and J. R. Schrieffer, Phys. Rev. 108, 1175 (1957)
For a review, see, e.g., R. Shankar, Rev. Mod. Phys. 66, 129 (1994)
A. J. Rimberg, T. R. Ho, Ç. Kurdak, J. Clarke, K. L. Campman, and A. C. Gossard, Phys. Rev. Lett. 78, 2632 (1997)
P. W. Anderson, Science 235, 1196 (1987)

About the Author

Dung-Hai Lee obtained his B.S. degree from Tsinghua University in Taiwan. He completed his Ph.D. in Physics (1977–1982) and a first post-doc (1982–1984) at MIT. He did further post-doctoral work at the IBM T. J. Watson research center (1983–1985), where he remained as a Research Staff Member until 1994. Since 1994, he has been a professor in the Physics Department of the University of California, Berkeley. Dung-Hai Lee’s main research interest is in strongly correlated many-particle systems. He has contributed to the fields of magnetism and superconductivity and the study of quantum Hall systems and classical and quantum phase transitions. His current interest is in the physics of novel superconductors and topological states in condensed matter systems.