A Crumpled Sheet’s Remembrance of Things Past
What does a material “know” about its past? Exploring this question can yield insights into a material’s internal structure and dynamics. A new paper by Yoav Lahini and colleagues at Harvard University [1] shows that the act of crumpling a thin sheet—for instance, wadding up a candy wrapper—allows it to “remember” past events for days. This memory effect indicates that crumpled sheets are more similar to other structurally disordered systems, like glass, than previously thought.
A material that’s squeezed, poured into a container, or put into a refrigerator will eventually relax to an equilibrium state that matches its new conditions. The simplest form of this relaxation is exponential in time. Specifically, a variable that describes the system (like temperature) will evolve from its initial value to its equilibrium value according to the equation , where is the characteristic time of the relaxation. Exponential relaxation keeps things on schedule: after waiting just , relaxation is more than 99% complete. It is a good model for how silly putty gradually conforms to the shape of your hand or for how soup cools in a thermos over the course of a day.
However, some systems are more recalcitrant and relax logarithmically in time. At this pace, a soup that takes 10 seconds to cool 1 degree would take 100 seconds to cool 2 degrees, and 10 billion seconds (a few centuries!) to cool 10 degrees. This behavior is found when we track quantities like the density or dielectric susceptibility of glasses [2]—materials whose molecules are in a highly disordered arrangement. For all practical purposes, a glass will never reach equilibrium. The same is true for a host of nonequilibrium systems, including piles of grains, concentrated emulsions like mayonnaise, and crumpled sheets. Unlike the state of an equilibrium material, which depends only on the conditions of the present (say, the temperature of the room), the state of a nonequilibrium material can carry an imprint of the past. This stored information, or memory, can affect the behavior of a material as it evolves further in time.
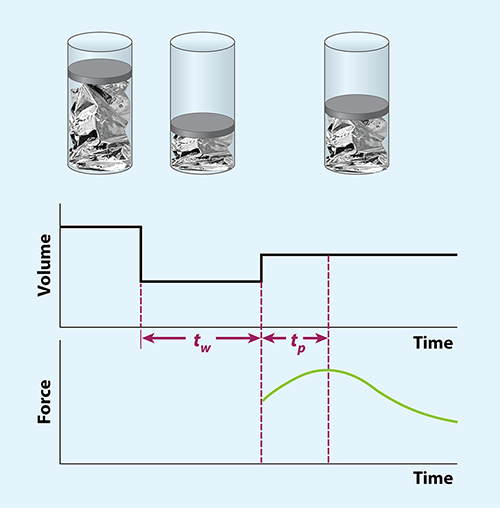
Lahini and colleagues decided to look for glass-like memory in crumpled sheets of Mylar, the shiny material found in candy bar wrappers and novelty balloons. Mylar might not appear to have much in common with a glass, but the researchers’ instincts had some backing. Experiments have found that when a weight is placed on a crumpled sheet, the sheet’s volume decreases logarithmically for weeks [3] (see 1 February 2002 Focus story). It’s also known that crumpled sheets retain traces of their former uncrumpled states [4]. In their experiments, Lahini et al. uncovered a new form of this memory capacity. They placed a crumpled sheet of Mylar inside a cylinder with a moving lid and abruptly compacted the sheet to a prescribed volume (Fig. 1). After a “waiting time,” the lid was moved back slightly and the sheet was allowed to expand. The sheet exerted a force on the lid that increased for hours, peaked, and then started to fall again. The authors then showed that, as it reached its peak force, the material was in fact “recalling” how long it had waited for the lid to retract: in repeated measurements, the time the sheet took to reach the peak force was proportional to the waiting time. The material retained this memory faithfully even in the longest experiment attempted, which lasted nearly two days. The authors also demonstrated the same memory effect in pieces of compressed plastic foam.
This behavior is reminiscent of a memory effect discovered in the 1960s by André Kovacs in glass and glass-like materials that are subjected to successive changes in temperature [5]. To explain their new results, Lahini and co-workers started with an idea from the physics of glasses that treats the material as though it consists of many subsystems, each with its own relaxation time [6]. Such a model makes sense for materials with a lot of internal disorder—be it in the arrangement of creases in a crumpled sheet or of molecules in a glass. Without specifying what these subsystems looked like, the researchers simply assumed that the subsystems’ relaxation times varied widely—from fractions of a second to weeks or more—and that the slowest ones were much more numerous than the fastest. Using this picture, the authors showed that the sum of these subsystems leads to a crumpled sheet whose relaxation is logarithmic in time. Moreover, the diversity of relaxation times allows a division of labor in the crumpled sheet’s memory: slower parts of the material retain an imprint of the long-ago initial compaction, while faster parts are attuned to the more recent release of the lid. Together, these parts form a memory of the time that elapsed between the two events.
This work highlights two trends in physics. First, it further tears down the walls that separate disordered molecular materials like glass—in which quantum and thermal effects can’t be dismissed—from disordered mechanical systems like crumpled sheets and piles of grains [2]. This strengthens the prospects for using disordered mechanical systems to study fundamental aspects of “glassiness,” as new insights may be possible when one can listen to a relaxing system’s crackles [7] or map its interior folds [4]. For example, the many-subsystem model used by Lahini et al. offers a good explanation for the memory of crumpled sheets, but it does not reveal what the subsystems are or how they came to be. Because they are macroscopic, crumpled sheets might provide an opportunity to develop a clearer physical picture of these subsystems, one that could carry over to less easily analyzable materials.
Second, this work reinforces an important role for memory in physics: measuring what a material “knows” can reveal commonalities among seemingly disparate systems. For example, suspensions of particles in liquid share their memory behavior with the arrangement of charges in so-called charge-density wave conductors [8] (see 30 June 2011 Synopsis). And glasses have a memory behavior called rejuvenation, which is also seen in a computer program that sorts lists of numbers [9]. In each of these systems, memory seems connected to a very broad range of relaxation times. Recent work also poses another question for crumpled sheets [8, 10]: whether—and how—they can recall multiple pieces of information, such as a repeating series of deformations. The next time you crumple a candy wrapper, you'll be giving it a second life at the forefront of physics.
This research is published in Physical Review Letters.
Correction (28 February 2017): An earlier version of the article inadvertently implied that the model used by Lahini et al. was described in [6]. This reference was provided for general background, as the revised article now indicates.
References
- Y. Lahini, A. Amir, and S. M. Rubinstein, “Nonmonotonic Aging and Memory Retention in Disordered Mechanical Systems,” Phys. Rev. Lett. 118, 085501 (2016).
- L. Berthier and M. D. Ediger, “Facets of Glass Physics,” Phys. Today 69, 40 (2016).
- K. Matan, R. B. Williams, T. A. Witten, and S. R. Nagel, “Crumpling a Thin Sheet,” Phys. Rev. Lett. 88, 076101 (2002).
- A. D. Cambou and N. Menon, “Orientational Ordering in Crumpled Elastic Sheets,” Europhys. Lett. 112, 14003 (2015).
- A. J. Kovacs, “Transition Vitreuse dans les Polymères Amorphes. Etude Phénoménologique,” Adv. Polym. Sci. 3, 394 (1963).
- For a general reference on this idea see, for example, L. Berthier, “Dynamic Heterogeneity in Amorphous Materials,” Physics 4, 277801 (2011).
- J. P. Sethna, K. A. Dahmen, and C. R. Myers, “Crackling Noise,” Nature 410, 242 (2001).
- N. C. Keim and S. R. Nagel, “Generic Transient Memory Formation in Disordered Systems with Noise,” Phys. Rev. Lett. 107, 010603 (2011).
- L.-N. Zou and S. R. Nagel, “Glassy Dynamics in Thermally Activated List Sorting,” Phys. Rev. Lett. 104, 257201 (2010).
- D. Fiocco, G. Foffi, and S. Sastry, “Encoding of Memory in Sheared Amorphous Solids,” Phys. Rev. Lett. 112, 025702 (2014).