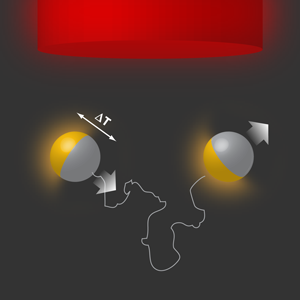
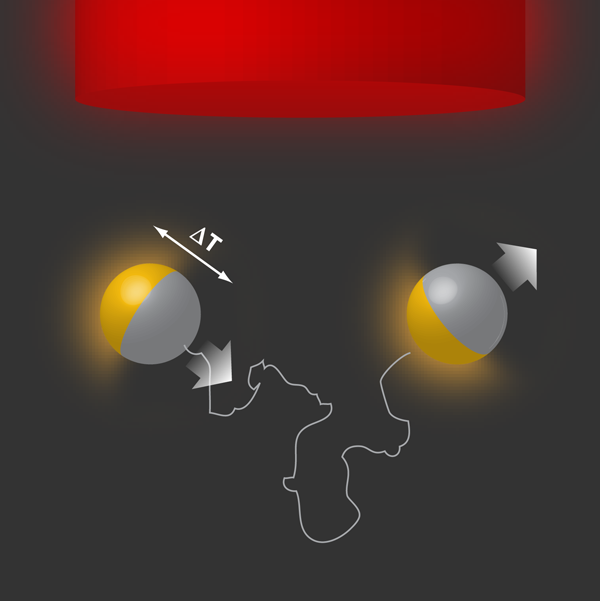
Debut of a hot “fantastic voyager”

Ramin Golestanian

Viewpoint

Debut of a hot “fantastic voyager”

Ramin Golestanian

The Rudolf Peierls Centre for Theoretical Physics, University of Oxford, 1 Keble Road, Oxford OX1 3NP, United Kingdom

December 20, 2010• Physics 3, 108

A temperature effect could allow us to dynamically change the stochastic behavior of certain colloidal particles.

Figure caption — **Figure 1:** Self-thermophoresis: The colloid has an engine—a hemispherical metallic coating—on board that can convert local radiation into heat via absorption. It then takes advantage of the asymmetrically created temperature profile, or more precisely, its gradient, to provide propulsion in a fixed direction relative to its own body by way of the Soret effect (see text). The active colloid will then follow a stochastic trajectory, but take its engine with it wherever it goes in the course of its motion. The mechanism will work anywhere along the trajectory so long as local radiation is available to provide “fuel” for the engine.

The busy life in living cells involves a great deal of transport activities and mechanical tasks, which are undertaken by motor proteins—molecular machines that convert chemical energy into mechanical work. In recent years, these remarkable machines have inspired artificial devices that deliver mechanical work [1] or propel themselves in a viscous environment [2]. We do not yet understand the mechanism behind the complex mechanochemical coupling in motor proteins. Standard rules used in macroscale engineering do not work at the nanoscale. New strategies are needed for the development of artificial nanoscale machines.

In a paper in Physical Review Letters, Hong-Ren Jiang and Masaki Sano of the University of Tokyo and Natsuhiko Yoshinaga of Kyoto University, both in Japan, demonstrate an innovative way to make self-propelled colloidal particles using partial metal coating and laser heating [3]. This work opens up new approaches to developing and studying these complex colloids.

Scientists have studied the motion of colloidal particles under the effect of external fields—phoretic transport—for more than a century. The external field could come from a gradient in electrostatic potential (electrophoresis), solute concentration (diffusiophoresis), or temperature (thermophoresis). The particles move because of the interaction of the ambient fluid with the modified interfacial structure near their surfaces. Though “driven” by these external fields, the colloidal particles experience no net force. In electrophoresis, the electric field applies equal and opposite forces on the charged colloid and the comoving counterions (neutralizing ions). In diffusiophoresis, the reactions of the forces exerted by the asymmetric distribution of solute particles on the colloid push back on the cloud that moves with it. In thermophoresis, similar mutual interactions are involved, depending on the constituents of the system (binary mixture, charged colloid, etc).

The force-free nature of phoretic transport mechanisms suggests that they could be used in designing self-propelled particles, provided we have a gradient that causes directed motion, e.g., by using Janus particles (colloidal particles made of two halves with different physical properties) with built-in sources [4]. This idea was used to propose self-diffusiophoretic colloids that achieve propulsion by asymmetrically catalyzing a chemical reaction on their surfaces [5]. This proposal was realized experimentally, using polystyrene Janus particles half-coated with platinum in a solution of hydrogen peroxide [6]. Now, Jiang et al. show that similar Janus particles (made of silica and half-coated with gold in their experiment) exhibit self-thermophoresis when irradiated with a defocused laser beam. The metal patch absorbs energy from the laser beam and converts it into heat, which is then released asymmetrically around the colloid. This leads to a sustained temperature gradient across the colloid and along its instantaneous orientation, which then drives the particle via thermophoresis (also known as the Soret effect), as illustrated in Fig. 1. This constitutes the self-propelled version of “hot Brownian motion” (Brownian motion under nonequilibrium conditions with locally enhanced temperatures) [7]. The authors report propulsion velocities of the order of a few microns per second for low to moderate laser powers, which are similar to the observed velocities for self-diffusiophoretic colloids.

Jiang et al.’s experiment is remarkable in two ways. In addition to demonstrating that the self-phoretic propulsion strategy works for a new class of phoretic mechanisms, namely, for thermophoresis, it provides an opportunity to study the Soret effect at the single-particle level. This is a significant improvement over conventional techniques [8], which mostly rely on the migration of colloidal particles in an imposed temperature gradient and cannot directly isolate single-particle effects. Jiang et al. have beautifully demonstrated an example of such miniature studies of the Soret effect, by adding surfactants that adsorb on the surface of the colloidal particles, leading to a sign change for the Soret coefficient, as shown recently [8]. Although the direct method based on self-thermophoresis is still not as accurate as conventional methods, the fact that it provides an entirely new probe could prove useful in tackling some unresolved aspects related to the Soret effect, such as the size dependence of the Soret coefficient and the validity of the thermodynamic descriptions of the phenomenon [8].

Self-diffusiophoretic colloids have been studied under confinement [9], hydrodynamic flow [10], and gravity [11]. The different circumstances point to exciting directions for future work through similarities between self-diffusiophoresis and self-thermophoresis, which amounts to replacing the concentration $C$ by the temperature $T$ , and the diffusiophoretic mobility $μ$ by the thermodiffusion coefficient $D_{T}$ [4]. Self-diffusiophoretic colloids exhibit stochastic motion that depends on the time scale [12]. Four dynamical regimes exist due to the presence of three distinct time scales in the problem—the vorticity diffusion time $τ_{h}$ , the solute diffusion time $τ_{d}$ , and the colloidal rotational diffusion time $τ_{r}$ .

The temperature of a self-thermophoretic colloid obeys the diffusion equation $\partial_{t} T - χ \nabla^{2} T = 0$ , where the thermophoretic conductivity $χ = κ / (ρ c_{p})$ (defined in terms of the thermal conductivity $κ$ , the mass density $ρ$ , and the specific heat at constant pressure $c_{p}$ ) replaces the solute diffusion coefficient. The equation for temperature is subject to the boundary condition that on the surface of the colloid the normal heat flux is proportional to the laser intensity $I$ with an absorption efficiency $ε$ . The stochastic motion of self-thermophoretic colloids is controlled by the three timescales noted above, with the solute diffusion time $τ_{d}$ replaced by the heat diffusion time, defined as $τ_{h d} = R^{2} / χ$ for a colloid of radius $R$ . For water at room temperature, $χ ≃ 10^{5} μ m^{2} / s$ , or equivalently, $τ_{h d} = 10^{- 5} (R / 1 μ m)^{2} s$ , which is one order of magnitude larger than the vorticity diffusion time $τ_{h}$ .

An entirely new feature of self-thermophoretic colloids (as compared to the other types of self-phoretic particles) is the dependence of the propulsion velocity on the intensity of the external laser. This suggests that the dynamical properties of the colloid could be modulated with intensity that is dependent on time. This variability could come from intrinsic fluctuations in the laser intensity or controlled intensity modulations (e.g., acousto-optic or holographic modulators). Moreover, spatial modulations of the laser intensity would also affect the dynamics of the colloids (artificial phototaxis). Therefore self-thermophoresis could give us a new control mechanism to dynamically change the stochastic behavior of active colloidal particles.

References

E. R. Kay, D. A. Leigh, and F. Zerbetto, Angew. Chem. Int. Ed. 46, 72 (2007)
S. J. Ebbens and J. R. Howse, Soft Matter 6, 726 (2010)
H-R. Jiang, N. Yoshinaga, and M. Sano, Phys. Rev. Lett. 105, 268302 (2010)
R. Golestanian, T. B. Liverpool, and A. Ajdari, New J. Phys. 9, 126 (2007)
R. Golestanian, T. B. Liverpool, and A. Ajdari, Phys. Rev. Lett. 94, 220801 (2005)
J. R. Howse, R. A. L. Jones, A. J. Ryan, T. Gough, R. Vafabakhsh, and R. Golestanian, Phys. Rev. Lett. 99, 048102 (2007)
D. Rings, R. Schachoff, M. Selmke, F. Cichos, and K. Kroy, Phys. Rev. Lett. 105, 090604 (2010)
R. Piazza and A. Parola, J. Phys.: Condens. Matter 20, 153102 (2008)
M. N. Popescu, S. Dietrich, and G. Oshanin, J. Chem. Phys. 130, 194702 (2009)
Y.-G. Tao and R. Kapral, Soft Matter 6, 756 (2010)
J. Palacci, C. Cottin-Bizonne, C. Ybert, and L. Bocquet, Phys. Rev. Lett. 105, 088304 (2010)
R. Golestanian, Phys. Rev. Lett. 102, 188305 (2009)

About the Author

Ramin Golestanian is a Professor at the Rudolf Peierls Centre for Theoretical Physics, University of Oxford. He obtained his B.Sc. from Sharif University of Technology, and his M.Sc. and Ph.D. from the Institute for Advanced Studies in Basic Sciences (IASBS), both in Iran. He has been a Visiting Scholar at MIT, Postdoctoral Fellow at the Kavli Institute for Theoretical Physics at UCSB, Joliot Chair and CNRS Visiting Professor at ESPCI, and Visiting Professor at College de France. Before joining Oxford, he held academic positions at IASBS and the University of Sheffield. His research interests are in statistical and mechanical properties of soft and biological matter.