Untangling the Orbitals in Iron-Based Superconductors

Daniel Podolsky

Viewpoint

Untangling the Orbitals in Iron-Based Superconductors

Physics Department, Technion, Haifa 32000, Israel

May 30, 2012• Physics 5, 61

Symmetry considerations point to a universal mechanism responsible for superconductivity in the iron pnictides and iron chalcogenides.

Figure caption — **Figure 1:** (Top) Lattice structure of the iron-based superconductors. Note that the atomic positions are symmetric under a rotation by 90 degrees in the $a b$ plane, followed by a reflection $c \to - c$ . This is the basic operation of the $S_{4}$ symmetry. (Bottom) The gauge transformation consists of multiplying by a minus sign the electronic creation and annihilation operators on the orange iron sites. The transformation changes the pairing symmetry from $s$ wave to $d$ wave, as shown.

The iron-based superconductors have generated great excitement since their discovery in 2008 [1]. They constitute a large and varied family of materials, including the iron pnictides and the iron chalcogenides, with superconducting temperatures reaching above $50 K$ . At first glance, there are many parallels between these materials and their older high transition temperature ( $T_{c}$ ) relatives, the cuprates (see 15 September Trends) [2]. For instance, the phase diagrams in both sets of materials are qualitatively similar, with antiferromagnetic parent compounds giving way to superconductivity upon doping. However, a deeper look reveals marked differences between the two, as would be expected from their drastically different chemistries. For example, one band of carriers is sufficient to capture the low-energy physical properties of the cuprates. In contrast, the band structure in the iron-based superconductors contains multiple electron and hole bands. In order to describe these bands, the common current belief is that one must include all five iron $d$ orbitals. In a paper in Physical Review X [3], Jiangping Hu and Ningning Hao at the Chinese Academy of Sciences, Beijing, and Purdue University, US, take a fresh approach on the minimal model for iron-based superconductors.

Early attempts to establish effective models for the iron-based superconductors, based on two [4] or three orbitals [5], were met with limited success. The low-energy dispersions of these models and the orbital character of the predicted Fermi surfaces did not yield good agreement with band-structure calculations based on the local-density approximation. This led to a consensus view that a microscopic treatment requires all five $d$ orbitals. However, this state of affairs is unsatisfactory for reasons that go beyond the obvious practical complications involved in working with five orbitals. Many of the physical properties of the iron-based superconductors are surprisingly robust. For example, angle-resolved photoemission spectroscopy (ARPES) studies have been able to map out the anisotropy in momentum of the superconducting energy gap, and they find a superconducting pairing symmetry of the form $cos k_{x} cos k_{y}$ across many members of this large group of superconductors [6]. Probes other than ARPES do find variations in physical properties that are material specific [7], but these are likely to be due to crossings of the material-dependent Fermi surfaces with the zeros of the order parameter, rather than a change in the pairing symmetry of the superconductivity. It is difficult to reconcile the robustness of the pairing symmetry with the vast phase space available in a five-orbital model. This hints to the existence of some underlying structure which greatly simplifies the microscopic Hamiltonian of real materials.

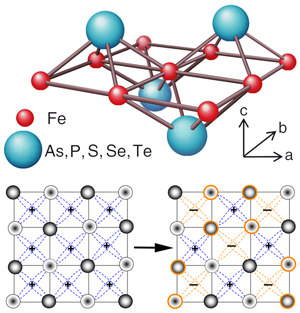
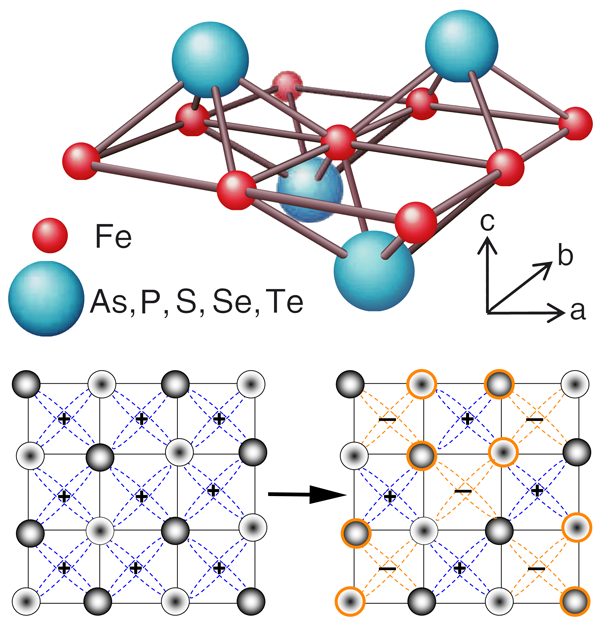
The starting point in Hu and Hao’s analysis is the observation that any microscopic treatment of iron-based superconductors must incorporate the $S_{4}$ lattice symmetry. The iron pnictides contain $FeAs$ layers, composed of iron atoms arranged in a square lattice on the $a b$ plane, and $As$ atoms at the center of the iron plaquettes, whose $c$ coordinate alternates in sign between positions above and below the iron planes [see Fig. 1 (top)]. (This same arrangement is found in the iron chalcogenides, once the arsenic atoms are substituted by sulphur, selenium, or tellurium.) The $S_{4}$ symmetry then corresponds to performing a 90 degree rotation on the $a b$ plane, while simultaneously reflecting $c \to - c$ , which is seen in Fig. 1 (top) to leave the lattice invariant. Note that if one were to ignore the $As$ atoms, the irons would form a simple square lattice with tetragonal symmetry, $C_{4 v}$ . However, the arsenic atoms play an important role in mediating the hopping between irons, and hence one must consider a lattice with two irons per unit cell and with $S_{4}$ symmetry.

The fact that hopping is primarily mediated through arsenic atoms allows the identification of $d_{x z}$ and $d_{y z}$ as the two most important iron orbitals. These states map into each other under an $S_{4}$ transformation, and hence form the basis of an $S_{4}$ “isospin” doublet. As Hu and Hao demonstrate, the two states in this isospin doublet mix weakly with each other, leading to a Hamiltonian composed of two almost-decoupled bands, each of which contains a single $d$ orbital per iron site. Remarkably, despite the simplicity of this description, the model gives good quantitative agreement with the low-energy band structure obtained in ab initio calculations. The model contains very few free parameters, yet it can accommodate the big changes seen in the band structure across different material classes. For instance, the iron pnictides contain holelike Fermi pockets that are not present in some of the iron chalcogenides. This change in Fermi surface topology is accounted for by tuning the value of a single hopping parameter in the model. These successes highlight the power of the two-orbital model.

The model’s underlying structure becomes fully transparent after performing a unitary transformation, in which the electron operators in one out of every two iron sites are multiplied by a minus sign, as shown by the orange sites in Fig. 1 (bottom). This gauge transformation amounts to a convenient change of basis, which makes the Hamiltonian easier to understand without altering its physical properties. It has two effects: First, it shifts the Fermi surfaces so that they all lie in a common region of the Brillouin zone. The second effect is to change the symmetry of the hopping, from $d$ wave to $s$ wave, while simultaneously modifying the superconducting pairing symmetry, from $s$ wave to $d$ wave. Thus, in the new gauge, the band structure becomes simple, and the superconducting pairing and hopping symmetries obtain a similar form as in the cuprate superconductors. Thus, in this gauge, the question of the pairing symmetry reduces to the well-studied problem of pairing symmetry in the cuprates. The reason for its robustness then becomes clear: a sign change in the superconducting order parameter is inevitable for a system with $s$ -wave hopping and repulsive interactions.

Most significantly, the analysis of Hu and Hao points to a common mechanism for superconductivity in both the iron pnictides and iron chalcogenides. Previous work has emphasized the role of interactions between electron and hole pockets in giving rise to superconductivity in the iron pnictides [8]. On the other hand, some of the iron chalcogenides do not have hole pockets, which has been used to suggest that an entirely different mechanism must be responsible for superconductivity in these materials. However, the current analysis shows that after the gauge transformation, the microscopic structure in both sets of materials becomes remarkably similar, pointing to a universal pairing mechanism. This helps explain the observation that the maximal measured $T_{c}$ , of order $50 K$ , is comparable for systems with and without hole pockets.

Further checks of the two-band model are needed to help establish it as a standard model to study the iron-based superconductors. As with other methods based on a finite number of orbitals, it is likely that the model will not fully comply with all the orbital symmetries at the Fermi surface. However, the simplicity and quantitative success of the current analysis in reproducing the low-energy band structure in the iron-based superconductors makes it a promising starting point for further analyses. The work introduces weakly interacting $S_{4}$ isospin doublets as the basic building blocks from which to build microscopic models. With the addition of correlation effects, this opens up many exciting possibilities for the improved future understanding of the iron-based superconductors.

References

Y. Kamihara, T. Watanabe, M. Hirano, and H. Hosono, “Iron-Based Layered Superconductor La[O{1-x} F{x}]FeAs (x = 0.05-0.12) with Tc = 26 K,” J. Am. Chem. Soc. 130, 3296 (2008)
M. Norman, “High-Temperature Superconductivity in the Iron Pnictides,” Physics 1, 21 (2008)
J. Hu and N. Hao, S4 Symmetric Microscopic Model for Iron-Based Superconductors, Phys. Rev. X 2, 021009 (2012)
S. Raghu, X.-L. Qi, C.-X. Liu, D. J. Scalapino, and S.-C. Zhang, “Minimal Two-Band Model of the Superconducting Iron Oxypnictides,” Phys. Rev. B 77, 220503 (2008); See also the accompanying Physics Synopsis http://physics.aps.org/synopsis-for/10.1103/PhysRevB.77.220503
M. Daghofer, A. Nicholson, A. Moreo, and E. Dagotto, “Three Orbital Model for the Iron-Based Superconductors,” Phys. Rev. B 81, 014511 (2010)
P. Richard, T. Sato, K. Nakayama, T. Takahashi, and H. Ding, “Fe-Based Superconductors: An Angle-Resolved Photoemission Spectroscopy Perspective,” Rep. Prog. Phys. 74, 124512 (2011)
P. J. Hirschfield, M. M. Korshunov, and I. I. Mazin, “Gap Symmetry and Structure of Fe-based Superconductors,” Rep. Prog. Phys. 74, 124508 (2011)
A. V. Chubukov, D. V. Efremov, and I. Eremin, “Magnetism, Superconductivity, and Pairing Symmetry in Iron-Based Superconductors,” Phys. Rev. B 78, 134512 (2008)
F. Wang and D.-H. Lee, “The Electron-Pairing Mechanism of Iron-Based Superconductors,” Science 332, 200 (2011)

About the Author

Daniel Podolsky received his Ph.D. from Harvard University in 2005. After postdoctoral fellowships at the University of California at Berkeley and the University of Toronto, he joined the Department of Physics at Technion – Israel Institute of Technology, where he has been an Assistant Professor since 2009. His current areas of interest are strongly correlated electronic and atomic systems, non-equilibirum dynamics, quantum magnetism, and superconductivity.