Two Traps are Better than One
Over the past 60 years, atomic physicists have developed sophisticated techniques to trap and isolate single neutral atoms and ions. Now they are tweaking these techniques so they can re-assemble isolated particles into pairs, triplets, and larger arrays. These carefully controlled multiparticle systems could be used to simulate the behavior of solids, or function as prototype quantum bits for storing information and performing computations. An important technological step toward preparing ions for these types of experiments is now reported in Physical Review Letters by Leon Karpa and colleagues at the Massachusetts Institute of Technology, Cambridge [1]. The team combined two key trapping technologies—optical lattices and Coulomb potentials—into a hybrid trap that keeps ions anchored into one position for up to times longer than existing lattice traps, even in the presence of external or stray electric fields. Ultimately, this highly stable trap could be used to capture arrays of ions to explore many-body solid-state effects.
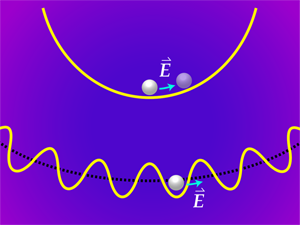
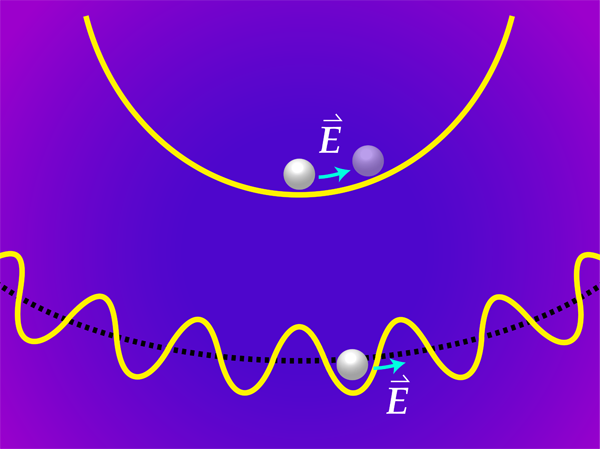
Techniques for storing charged particles were first developed in the 1950s, when Wolfgang Paul and Hans Dehmelt independently designed and developed ion traps. To this day, Paul’s quadrupole trap, which uses a combination of alternating (ac) and static (dc) fields to confine strings of ions, is a key component of mass spectrometers. A common type of quadrupole trap is the linear Paul trap, in which ac fields generate a two-dimensional, saddle-shaped potential that rotates at tens of millions of revolutions per second (radio frequencies.) The rotation is too fast for the ions to follow, so they experience an effective bowl-shaped potential that traps them at the minimum. Static fields along the third dimension provide additional confinement.
By the early 1980s, physicists had confined single barium and magnesium ions in quadrupole traps. (Simultaneous advances in laser cooling, which slows ions and neutral atoms as they are loaded into a trap, played an essential role in these early trapping experiments.) Over the following decades, researchers demonstrated they could manipulate and read out the quantum states of individual ions with ever-increasing precision. Today, the world’s most accurate clock is based on the frequency of a trapped aluminum ion; and many proposed quantum-computing platforms rely on trapped ions.
Given this success, why are ion trappers turning to new traps? An important goal is to gain more control over the shape of the trapping potential, especially on short length scales. Achieving this would offer greater freedom to position the ions in tailored configurations and explore different interactions between ions. While quadrupole traps create deep trapping potentials, on the order of electron volts ( kelvin), they only have a simple parabolic structure. Shaping the dc fields can produce more complex trap geometries. But the length scales of these potentials are still on the order of hundreds of micrometers, which limits how closely together two ions can be positioned if each is in its own local minimum.
In contrast, an optical lattice trap, which is formed by superimposing two counterpropagating laser beams to make a standing wave, can trap atoms a few hundreds of nanometers apart. In these traps, the particles are locked by dipolar forces into the local minima of the standing wave generated by the laser field. The trap shapes can be further tailored by tuning the angles and wavelengths of the beams. These traps work particularly well for isolating and manipulating neutral atoms. But with ions, the dipole forces from the laser fields are generally too weak to overcome the effects of stray electric fields on the charged particles. Researchers at the Max Planck Institute for Quantum Optics and the University of Freiburg in Germany have successfully trapped an ion in a focused Gaussian beam for a few milliseconds (ms) [2]. In 2012, the same group trapped an ion for up to microseconds ( ) in an all-optical lattice by first catching the particle in a standard quadrupole trap, then gradually turning off the radio-frequency fields while ramping up the laser beams [3].
Hybrid traps combine the stability of quadrupole traps with the flexibility of optical traps. Moreover, the radio-frequency and optical fields produce a combination of Coulomb and dipole forces, respectively, on the ion, which can be used to simulate many-body Hamiltonians. For example, one candidate model, the Frenkel-Kontorova Hamiltonian, describes nearest-neighbor interactions within a sinusoidal potential and exhibits both classical and quantum phase transitions [4]. There have already been a few experiments with hybrid traps. A team at Aarhus University in Denmark, for example, tried leaving their quadrupole trap on and showed the ion stayed within a single well of the optical lattice [5]. Several experiments used separate, but overlapping, quadrupole and laser traps for ions and atoms, respectively, to explore collisions between the charged and neutral particles at ultracold temperatures where quantum effects are dominant [6–9]. The idea is that if a single ion is trapped in a sea of atoms, its fluorescence can be a sensitive probe of how it interacts chemically with the surrounding particles. These experiments, however, grappled with the effects of micromotion, which is the residual motion of the ion at the radio frequency used to drive the quadrupole trap.
Like the researchers at Aarhus University, the MIT team uses a quadrupole trap in combination with an optical trap. The radio-frequency fields confine an ion tightly in two dimensions, while along the third axis the static fields are relaxed so that the ions see a relatively flat landscape. Karpa et al. introduce a one-dimensional lattice along this axis by driving an optical resonator with a laser field (Fig. 1.) The key step forward is that their hybrid trap locks an ion into more localized positions for a longer period of time, thanks to a built-in cooling mechanism. Specifically, an additional laser field removes one vibrational quanta after another from the trapped ion, a technique known as Raman sideband cooling. The cooling cycle leaves the ion in its vibrational ground state. The cooler ion stays spatially localized to within about nanometers (nm) and is pinned at a valley of the standing-wave structure. The team also shows they can use the same cooling and trapping method for up to three ions at a time.
To prove that their trap was stiff—meaning an ion wouldn’t be easily disrupted by local variations in the electric field—Karpa et al. pushed on the ions with a time-varying electric field. In the absence of the optical lattice, the ions swung back and forth with the field over hundreds of nm, but with the lattice on, they remained fixed in place. Only when Karpa et al. slowed down the oscillations in the field could the ions respond to the force. The researchers determined that the single-site pinning lasted for up to , which is times longer than the trap’s vibrational period. The vibrational period—classically, the time it takes an ion to roll back and forth in its potential well—sets the minimum time scale for which it makes sense to say that ion is trapped
In addition to other challenges, researchers working with hybrid traps will need to address the ion micromotion at radio frequencies. Although all-optical traps offer a cleaner option, they are presently limited to trapping times because laser fluctuations heat the ions [3]. Karpa et al. have already proposed one potential solution to micromotion: a hybrid trap design that uses static, as opposed to radio-frequency, fields. Exploring proposals such as this one could open the door to new approaches in a host of fields, ranging from ultracold quantum chemistry to quantum computing architectures to simulations of solid-state physics.
Correction (1 November 2013): Paragraph 5, sentence 4, “manipulating neutral ions” changed to “manipulating neutral atoms.”
References
- L. Karpa, A. Bylinskii, D. Gangloff, M. Cetina, and V. Vuletić, “Suppression of Ion Transport due to Long-Lived Subwavelength Localization by an Optical Lattice,” Phys. Rev. Lett. 111, 163002 (2013)
- C. Schneider, M. Enderlein, T. Huber, and T. Schaetz, “Optical Trapping of an Ion,” Nature Photon. 4, 772 (2010)
- M. Enderlein, T. Huber, C. Schneider, and T. Schaetz, “Single Ions Trapped in a One-Dimensional Optical Lattice,” Phys. Rev. Lett. 109, 233004 (2012)
- M. Johanning, A. Varón, and C. Wunderlich, “Quantum Simulations with Cold Trapped Ions,” J. Phys. B 42, 154009 (2009)
- R. B. Linnet, I. D. Leroux, M. Marciante, A. Dantan, and M. Drewsen, “Pinning an Ion with an Intracavity Optical Lattice,” Phys. Rev. Lett. 109, 233005 (2012)
- A. T. Grier, M. Cetina, F. Oručević, and V. Vuletić, “Observation of Cold Collisions between Trapped Ions and Trapped Atoms,” Phys. Rev. Lett. 102, 223201 (2009)
- C. Zipkes, S. Palzer, C. Sias, and M. Köhl, “A Trapped Single Ion Inside a Bose-Einstein Condensate,” Nature 464, 388 (2010)
- S. Schmid, A. Härter, and J. Hecker Denschlag, “Dynamics of a Cold Trapped Ion in a Bose-Einstein Condensate,” Phys. Rev. Lett. 105, 133202 (2010)
- W. G. Rellengert, S. T. Sullivan, S. Kotochigova, A. Petrov, K. Chen, S. J. Schowalter, and E. R. Hudson, “Measurement of a Large Chemical Reaction Rate between Ultracold Closed-Shell Ca Atoms and Open-Shell Yb Ions Held in a Hybrid Atom-Ion Trap,” Phys. Rev. Lett. 107, 243201 (2011)