Revisiting Thermodynamic Efficiency

Tomaž Prosen

Viewpoint

Revisiting Thermodynamic Efficiency

Tomaž Prosen

Faculty of Mathematics and Physics, Department of Physics, University of Ljubljana, Jadranska 19, SI-1000 Ljubljana, Slovenia

February 11, 2013• Physics 6, 16

Breaking time-reversal symmetry in a thermoelectric device affects its efficiency in unexpected ways.

Figure caption — **Figure 1:** A simple three-terminal thermoelectric device that converts heat into electrical current. The circle is a simple conductor in thermal and electrical contact with two temperature and charge reservoirs (the hotter reservoir is in red, the cooler reservoir in blue.) The third terminal is a probe (yellow), which, on average, does not exchange any heat or particles with the conductor. Brandner *et al.* show that turning on the magnetic field $B$ , can improve the efficiency of the device.

Thermodynamic engines convert heat into useful work. Testing the optimal efficiency of these machines has been at the forefront of scientific developments ever since 1824, when Sadi Carnot showed that in a simple engine undergoing a reversible thermodynamic cycle, the ratio between used and wasted heat must be less than the ratio of the absolute temperatures of the cold and the hot reservoir. This Carnot limit is simply a statement of the second law of thermodynamics.

On a practical level, knowing the ideal efficiency of engines and other devices helps us compare the advantages of using and developing one technology versus another. This is particularly important today, as the world faces energy challenges that could be mitigated by using available resources more efficiently. At the same time, studying the efficiency of thermodynamic engines helps us understand fundamental ideas, such as the relationship between the work an engine performs and the information gained or lost in the process, or the implications of a system being microscopically versus macroscopically reversible in time.

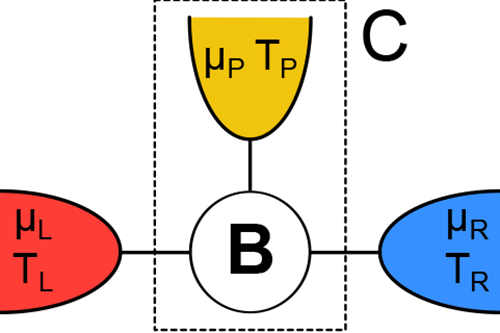
In this spirit, Kay Brandner at the University of Stuttgart, Germany, and colleagues [1] report in Physical Review Letters their calculated efficiency of a simple thermoelectric device that converts heat to electrical current (Fig. 1). They show that when the device operates in an external magnetic field—a condition that breaks time-reversal symmetry for the motion of electrons—the efficiency is significantly lower than previous studies predicted. The lower bound on efficiency occurs, they argue, because in addition to the requirement that entropy be greater than or equal to zero, charge must be conserved—a point that was missed in earlier work. Their findings improve our understanding of thermoelectric efficiency and may one day influence the design of thermoelectric devices for real-world applications.

The Carnot engine undergoes two isothermal and two adiabatic changes, and runs sufficiently slowly that the thermodynamic state of the engine at each step along the way is well defined. Carnot engines have an efficiency $η_{C}$ that depends on the temperatures of the two thermal reservoirs providing heat into and out of the engine ( $η_{C} = 1 - T_{cold} / T_{hot}$ ). As typically framed, the Carnot cycle is considered macroscopically reversible, since it is infinitely slow and produces no entropy.

In contrast, Brandner et al. consider the implications of reversibility and irreversibility on a microscopic scale, taking into account the individual trajectories of all of the electrons in a simple thermoelectric device (Fig. 1). Unlike the Carnot engine, this device operates in an out-of-equilibrium steady state, transforming the current of heat to an electric current at a finite voltage.

The efficiency of thermoelectric devices like the one Brander et al. consider depends on three material properties: the thermopower $S$ , which is the voltage produced by a temperature difference, the electric conductivity $σ$ , and the heat conductivity $κ$ . How the combination of these material properties combine to determine the thermoelectric’s efficiency can be found from the so-called Onsager matrix $L_{i j}$ , which connects the heat current ( $J_{q}$ ) and electrical current ( $J_{e}$ ), to a pair of thermodynamic gradients, namely of temperature $T$ , ( $F_{q} = - \nabla T / T^{2}$ ), and of electrochemical potential $μ$ , ( $F_{e} = \nabla μ / T$ ): $J_{q} = L_{q q} F_{q} + L_{q e} F_{e}$ and $J_{e} = L_{e q} F_{q} + L_{e e} F_{e}$ .

Lars Onsager [2], in work that led to his Nobel Prize in Chemistry, and later Hendrik Casimir [3], showed that the principle of microscopic time-reversibility means that $L_{q e} = L_{e q}$ , while breaking time-reversibility, say by applying a magnetic field $B$ , yields the general Onsager-Casimir relation $L_{q e} (B) = L_{e q} (- B)$ .

In the case that the system is microscopically reversible ( $B = 0$ ), the efficiency of the thermoelectric steady-state engine depends on the so-called figure of merit [4], $Z T = (σ S^{2} / κ) T = L_{e q}^{2} / det L$ . $Z T$ becomes larger the more singular the matrix $L$ becomes, and in the limit $Z T$ approaches infinity, one attains the Carnot efficiency. The only condition imposed by the second law of thermodynamics is that the entropy produced by the system be greater than or equal to zero, which simply implies the positivity of the matrix $L$ , or, positivity of $Z T$ . Optimizing $Z T$ is a significant challenge in material science [5]. So far, practical values of $Z T \sim 1$ are still too low for thermoelectric technology to compete with cycle-based engines or refrigerators. Notable exceptions are nanoscale devices [6] and heating or cooling devices where a specialized function, and not efficiency, is most important.

In view of these practical limitations, Benenti et al. [7] broke new ground by investigating bounds on thermoelectric efficiency in models where microscopic time-reversibility was broken (nonzero $B$ ). They derived a general expression for the thermoelectric efficiency $η$ in terms of two dimensionless parameters, the generalized figure of merit, $y = L_{q e} L_{e q} / (det L)$ , and the asymmetry parameter, $x = L_{e q} / L_{q e}$ . Considering only limitations imposed by the second law, they observed that a range of $x$ and $y$ values were possible. Significantly, they observed that it was possible to obtain Carnot’s efficiency for any value of asymmetry $| x | > 1$ . However, it remained unclear if their model contained all of the ingredients necessary to be considered realistic.

In their new work, Brandner et al. add this missing ingredient by focusing on a simple steady-state model with three terminals—or contacts—to heat and charge reservoirs (Fig. 1). In their model, only two of the terminals are connected to a source and drain of heat and electric charge, whereas the third terminal is merely a probe that does not, on average, exchange particles (in this case, charge) and energy with the environment. The reason that adding this third terminal is necessary is that the Onsager matrix for the simplest, two-terminal devices with noninteracting particles is always strictly symmetric ( $x = 1$ ), even in the presence of magnetic fields. The minimal model that can break this symmetry has to have three terminals. Brandner et al. essentially used a mathematical trick in which they re-parametrized the Onsager matrix in terms of a new matrix $K$ , the positivity of which is now a consequence of the law of conservation of charge. The requirement that $K$ be positive puts a constraint on the matrix elements of the Onsager matrix that is much stronger than the requirement from the second law and allows for no obvious anomalies, and means the efficiency cannot be as high as Benenti et al. predicted. Strong evidence that Brandner et al.’s work will apply to real world models comes from Ref. [8], in which the authors numerically calculated the efficiency of a specific, model device and found the same value that Brandner et al. now find as an upper bound.

Brandner et al.’s result is not completely bad news about the efficiency of thermoelectric devices. Systems with microscopic reversible dynamics can be shown to have a maximum theoretical efficiency (the Curzon-Ahlborn limit [9]) of $1 / 2 η_{C}$ if they operate at maximal output power. (Strictly speaking, this limits only holds when the difference of temperatures between hot and cold reservoirs is small with respect to absolute temperatures [10].) Using similar assumptions, Brandner et al. show that switching on a magnetic field or other vector potential, increases the optimal efficiency of their device at maximum power output to $4 / 7 η_{C}$ . This fundamental result has potentially interesting practical implications for developing small-scale heat engines or refrigerators with little compromise between efficiency and power.

Brandner et al.’s results immediately open up two other, challenging questions. For one, does a similar bound on efficiency exist in more general transport models with strong particle-particle interactions? Two, what should we expect in models with more than three terminals?

References

K. Brandner, K. Saito, and U. Seifert, “Strong Bounds on Onsager Coefficients and Efficiency for Three-Terminal Thermoelectric Transport in a Magnetic Field,” Phys. Rev. Lett. 110, 070603 (2013)
L. Onsager, “Reciprocal Relations in Irreversible Processes. I.,” Phys. Rev. 37, 405 (1931); "Reciprocal Relations in Irreversible Processes. II., ” 38, 2265 (1931)
H. B. G. Casimir, ”On Onsager’s Principle of Microscopic Reversibility,” Rev. Mod. Phys. 17, 343 (1948)
G. Mahan, B. Sales, and J. Sharp, “Thermoelectric Materials: New Approaches to an Old Problem,” Phys. Today 50, No. 3, 42 (1997)
W. Kim, J. Zide, A. Grossard, D. Klenov, S. Stemmer, A. Shakouri, and A. Majumdar, “Thermal Conductivity Reduction and Thermoelectric Figure of Merit Increase by Embedding Nanoparticles in Crystalline Semiconductors,” Phys. Rev.  Lett. 96, 045901 (2006)
T. E. Humphrey and H. Linke, “Reversible Thermoelectric Nanomaterials,” Phys. Rev. Lett. 94, 096601 (2005)
G. Benenti, K. Saito, and G. Casati, “Thermodynamic Bounds on Efficiency for Systems with Broken Time-Reversal  Symmetry,” Phys. Rev. Lett. 106, 230602 (2011)
B. Balachandran, G. Benenti, and G. Casati, “Efficiency of Three-Terminal Thermoelectric Transport Under Broken Time-Reversal Symmetry,” arXiv:1301.1570
F. L. Curzon and B. Ahlborn, “Efficiency of a Carnot Engine at Maximum Power Output,” Am. J. Phys. 43, 22 (1975)
C. Van den Broeck, “Thermodynamic Efficiency at Maximum Power,” Phys. Rev. Lett. 95, 190602 (2005)

About the Author

Tomaž Prosen has been a full professor of theoretical physics at University of Ljubljana since 2008, where he leads a group on nonequilibrium quantum and statistical physics. He obtained his Ph.D. in 1995 at the same university. His main current research interest is in fundamental problems in dynamical systems, quantum many-body dynamics, nonequilibrium statistical mechanics, and transport theory.