Light Avoids Anderson Localization
The confinement of waves in a disordered medium—Anderson localization [1]—has been observed for electromagnetic [2,3] and acoustic [4] waves in disordered dielectric structures, and for electron waves in condensed matter. Anderson localization arises as a result of the constructive interference between waves that follow time reversed paths as they loop back to a point as a result of scattering from defects. The effect is common in low-dimensional disordered systems because the restricted volume explored by scattered waves enhances the likelihood that waves will return to a point. Now, a new experiment conducted by Mohammad Hafezi at the Joint Quantum Institute of NIST and the University of Maryland, College Park, and collaborators reveals the virtually unimpeded flow of photons—the opposite of Anderson localization—in a one-dimensional channel along the edges of a two-dimensional disordered lattice. These findings, reported in Physical Review Letters [5], may inspire new ways of engineering photonics devices such as filters, switches, and delay lines that rely on the controlled propagation of light.
The first and best-known example of a system in which wave transport occurs despite disorder is the quantum Hall effect, in which a strong magnetic field acting on a two-dimensional electron gas creates topologically protected edge states. These states cannot scatter into other states and are therefore immune to backscattering and localization [6].
Although topological states were originally discovered in condensed-matter systems, analogous states based on light waves instead of electron waves could have useful applications, such as optical systems in which light does not backscatter upon encountering a defect. In 2008, theorists predicted that topological states might be found in photonic systems composed of magnetic materials [7]. Microwave measurements soon confirmed that magneto-optical effects in magnetic media can affect photons in a manner analogous to the influence of real magnetic fields on electrons [8]. Unfortunately, magneto-optical effects are weak at optical frequencies, and topological states could not be engineered for applications in the visible domain.
Recent discoveries of topological states in systems with time-reversal symmetry, such as the quantum spin Hall effect and topological insulators, stimulated the search for alternative ways to extend topological protection to photonics. Inspired by topological states in condensed-matter systems without magnetic fields, researchers have shown theoretically and experimentally that topological states of light can be implemented in a wide range of optical systems by engineering synthetic magnetic fields. These systems include coupled silicon resonators [9], chiral fibers [10], and bianisotropic metamaterials [11], as well as systems in which optical parameters can be modulated over time [12]. However, until now there has been no clear experimental evidence of topological protection against lattice disorder that would suppress Anderson localization in such photonic systems.
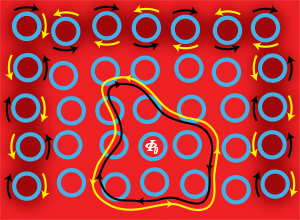
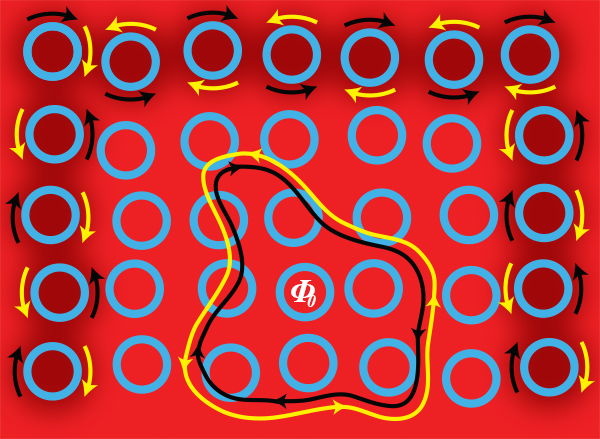
This is precisely what Hafezi and his group demonstrate, exploiting an approach proposed in their early theoretical work in which light traveling in an array of waveguides mimics spin states in the quantum spin Hall effect [13]. The researchers built a two-dimensional lattice of tiny, silicon, ring-shaped waveguides, each coupled to its neighbor by a linking waveguide (Fig. 1). When photons are injected into the lattice via an input port, they propagate through the lattice by “hopping” from one resonator to another. The waveguides have resonance frequencies in the near infrared and are designed such that light moving clockwise through a ring acquires a phase shift with respect to light moving counterclockwise—an effect analogous to that of a magnetic field acting on the spin-up and spin-down states of electrons moving in a quantum spin Hall system.
Hafezi and his collaborators deliberately introduced disorder into these lattices by varying the coupling between certain resonators and shifting the resonance frequency of certain ring waveguides. The researchers measured the time delay between when photons leave the lattice and when they are injected. They show that the distribution of delay times compared to the average is Gaussian for photons traveling along the edges. The distribution is asymmetric, however, for photons that propagate through the interior of the lattice (i.e., bulk states). These ensembles of delay times are consistent with bulk states that are localized by disorder—as expected—and edge-traveling photons that propagate nearly unimpeded. The researchers bolster their measurements with simulations, which show that the photonic edge states of the lattice are characterized by strong transmission. In contrast, waves in the bulk of the lattice are localized.
The synthetic magnetic field acting on photons gives rise to states that only propagate in one direction along the edges of the lattices. Specifically, photons on the edges of the lattice are limited to hopping around the perimeter of the lattice in the direction in which they were initially launched. The average time delay between when the photons are injected into the lattice and when they leave the lattice is linearly proportional to the number of ring resonators that the photons traverse, suggesting that the wave propagates via sequential resonant coupling between rings. Moreover, Hafezi and his team reported consistent results for lattices ranging in size from 4×4 to 18×18, demonstrating that the suppression of Anderson localization is not unique to a particular lattice size.
On a fundamental level, the work of Hafezi and collaborators opens up a new way to study Anderson localization with a high level of control over the experimental conditions. Indeed, the possibility of controlling the degree of disorder in random systems with synthetic gauge fields will stimulate researchers to test new fundamental aspects of Anderson localization in which light is biased to flow in a specific direction. This study lays the groundwork for exploring topological protection in photonics systems even with strong disorder.
Correction (20 August 2014): A revised version of the article was posted at 12.12 pm EST.
References
- A. Lagendijk, B. A. van Tiggelen, and D. S Wiersma, “Fifty Years of Anderson Localization,” Phys. Today 62, 24 (2009)
- A. A. Chabanov, M. Stoytchev, and A. Z. Genack, “Statistical Signatures of Photon Localization,” Nature 404, 850 (2000)
- T. Schwartz, G. Bartal, S. Fishman, and M. Segev, “Transport and Anderson Localization in Disordered Two-Dimensional Photonic Lattices,” Nature 446, 52 (2007)
- H. Hu, A. Strybulevych, J. H. Page, S. E. Skipetrov, and B. A. van Tiggelen, “Localization of Ultrasound in a Three-Dimensional Elastic Network,” Nature Phys. 4, 945 (2008)
- S. Mittal, J. Fan, S. Faez, A. Migdall, J. M. Taylor, and M. Hafezi, “Topologically Robust Transport of Photons in a Synthetic Gauge Field,” Phys. Rev. Lett. 113, 087403 (2014)
- K. Klitzing, G. Dorda, and M. Pepper, “New Method for High-Accuracy Determination of the Fine-Structure Constant Based on Quantized Hall Resistance,” Phys. Rev. Lett. 45, 494 (1980)
- F. Haldane, and S. Raghu, “Possible Realization of Directional Optical Waveguides in Photonic Crystals with Broken Time-Reversal Symmetry,” Phys. Rev. Lett. 100, 013904 (2008)
- Z. Wang, Y. Chong, J. D. Joannopoulos, and M. Soljačić, “Observation of Unidirectional Backscattering-Immune Topological Electromagnetic States,” Nature 461, 772 (2009)
- M. Hafezi, S. Mittal, J. Fan, A. Migdall, and J. M. Taylor, “Imaging Topological Edge States in Silicon Photonics,” Nature Photon. 7, 1001 (2013)
- M. C. Rechtsman, Julia M. Zeuner, Y. Plotnik, Y. Lumer, D. Podolsky, F. Dreisow, S. Nolte, M. Segev, and A. Szameit, “Photonic Floquet Topological Insulators,” Nature 496, 196 (2013)
- A. B. Khanikaev, S. Hossein Mousavi, W.-K. Tse, M. Kargarian, A. H. MacDonald, and G. Shvets, “Photonic Topological Insulators,” Nature Mater. 12, 233 (2013)
- K. Fang, Z. Yu, and S. Fan, “Realizing Effective Magnetic Field for Photons by Controlling the Phase of Dynamic Modulation,” Nature Photon. 6, 782 (2012)
- M. Hafezi, E. A. Demler, M. D. Lukin, and J. M. Taylor, “Robust Optical Delay Lines with Topological Protection,” Nature Phys. 7, 907 (2011)