Chasing the Exciton Condensate
Superfluids (fluids with zero viscosity) and superconductors (materials with zero resistance) have a common ingredient: bosons. These particles obey Bose-Einstein statistics, allowing a collection of them at low temperatures to collapse into a single quantum-mechanical state, or Bose-Einstein condensate. Bosons in superconductors consist of two paired electrons, but the pairing is weak and only occurs at low temperatures. In a quest to build devices that carry electricity with low dissipation at higher temperatures, researchers have therefore explored the possibility of engineering electrical condensates [1] out of strongly bound pairs of electrons and holes, or excitons. Now, two research groups have, independently, fabricated and characterized a graphene-based device that is thought to be a promising platform for realizing an exciton condensate [2, 3]. Neither group has yet found evidence for such a condensate—the ultimate goal of such experiments. But their measurements lay the groundwork for future searches.
Excitons form in semiconductors and insulators. The binding energy between the exciton’s electron and hole can be quite strong, greatly exceeding their thermal energy at room temperature. Unfortunately, excitons recombine quickly, too fast to allow a condensate to form. Although excitons coupled to light confined within a cavity can form hybrid particles (exciton-polaritons) that do live long enough to condense [4], such condensates require a continuous input of light.
Another option for preventing recombination is to simply separate the electron and hole in different semiconductor layers. This approach carries enormous technical challenges: the electron and hole must be closer to each other than to their neighbors in the same layer, requiring an interlayer spacing of a few nanometers. Exciton condensates have been realized in spatially separated semiconductor quantum wells. However, such devices either involved impractically high magnetic fields [5] or low temperatures [6].
The availability of atomically thin materials, such as graphene and insulating hexagonal boron nitride (hBN), which can be precisely assembled in layered structures, has renewed interest in searching for exciton condensates. Several years ago, theorists proposed that two graphene monolayers separated by an insulating layer could host a condensate above room temperature [7], though this prediction now appears optimistic [8]. More recent work has pointed out that for a condensate to form, strong electron interactions within each layer are needed. This condition can be satisfied by making each layer out of bilayer graphene, which consists of two graphene monolayers stacked in atomic registry [9].
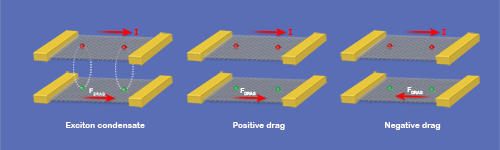
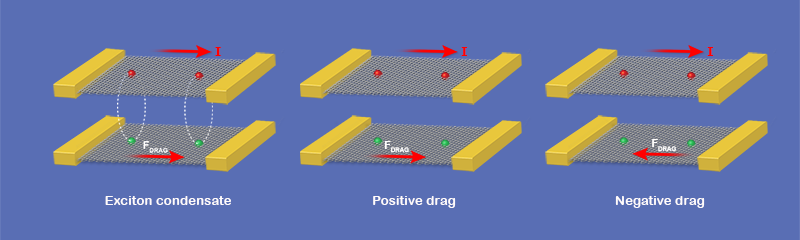
The new experiments, led by Cory Dean from Columbia University, New York, and by Emanuel Tutuc from the University of Texas at Austin, are significant because they are the first to explore such a system of two bilayer-graphene layers. Both teams prepared devices in which the bilayers are electrically isolated from one another by a hBN layer that is just a few nanometers thick. The only communication between the two bilayers is the Coulomb force between charge carriers. Their experiments entailed running a current through one bilayer, the “drive” layer, and measuring a voltage in the other bilayer, the “drag” layer (Fig. 1). The ratio of the voltage to current is called the Coulomb drag resistance, and it is a measure of how much the charges in one layer drag the charges in the other layer along with them. The quantity is a sensitive probe of the charge carriers and how they exchange energy and momentum with one another.
Both groups measured the drag resistance as a function of the carrier density in each of the two bilayers, which they controlled with voltages applied between the bilayers or to metallic gates above or below the bilayers. The telling signature of an exciton superfluid (Fig. 1, left) would be a drag resistance that diverges when the bilayer system enters the condensate phase. Although neither group found evidence for this divergence, they did find surprising results that are unanticipated theoretically and that differ dramatically from observations in devices with monolayer graphene.
Dean’s team studied devices at temperatures above 70 K, warmer than the approximately 10 K at which an exciton condensate is expected to form [9]. They observed three drag regimes as they varied the carrier density in the layers. In the first, associated with a high carrier density in both layers, the drag behavior is as expected for momentum exchange between charges in layers that are described as conventional Fermi liquids. The second regime corresponds to both layers having a carrier density near zero. Here, the authors saw a positive drag: holes in one bilayer pull electrons in the other bilayer in the same direction (Fig. 1, center). The magnitude of the drag is similar to that observed in monolayer-graphene devices, which has been explained as an energy-exchange effect between charges [10]. The idea is that disorder—from trapped charges or local strain fields—causes the formation of local puddles of electrons and holes, resulting in local hot and cold spots in the drive layer when a current is run through it (the Peltier effect). These spots induce local thermal gradients in the drag layer, in turn producing a voltage (the Seebeck effect). Finally, the authors identified a third regime at intermediate carrier density, in which charge carriers moving in the drive layer force carriers in the drag layer to move in the opposite direction (Fig. 1, right), regardless of the sign of the carriers. This inverted drag hasn’t been seen in other 2D systems and requires a new explanation.
Tutuc’s group focused on graphene bilayers with lower carrier densities and at lower temperatures, down to 1.5 K, a temperature at which an exciton condensate could have conceivably formed. They also observed unusual effects. First, they found that when the carrier density in both bilayers is zero, the drag resistance is huge—close to the resistance of bilayer graphene itself. (Normally, the drag resistance is a small fraction of either layer’s resistance.) Such a huge drag resistance is unanticipated by theories that describe the bilayers as Fermi liquids. The second surprising observation is that the drag is negative. Negative drag is expected when charge carriers of the same sign exchange momentum between the layers, thus any contribution from excitons can likely be ruled out. (See additional note in Ref. [11].)
To explain the anomalous drag, the Tutuc group also postulates that disorder-driven energy exchange plays a role [10]. Indeed, by independently measuring the carrier-density dependence of the Peltier effect between layers in samples with varying amounts of disorder, they were able to predict the onset of negative drag in several samples of double bilayer graphene. However, with graphene bilayers, the effect is orders of magnitude larger than with graphene monolayers, grows with lower temperature, and has an opposite sign. This may indicate a qualitatively different source of disorder than in monolayer-graphene devices and in the samples from Dean’s group, in which positive drag is seen when both bilayers were charge neutral. Until the sources of disorder in the two experiments are resolved, it remains an open question whether a detailed theory of energy or momentum exchange can explain both groups’ findings.
What do these observations mean for the search for exciton condensates with graphene? This first successful fabrication of double bilayer-graphene devices gives hope for future studies using cleaner samples, or systems with even stronger Coulomb interactions. The latter could be achieved either with a thinner dielectric between the two layers, or using materials in which electrons and holes have an increased effective mass, suppressing the zero-point kinetic energy of the charges that shakes excitons apart. These changes could lead to the observation of new many-body states and possibly the realization of exciton condensation at high temperatures.
Some of the differences between the two groups’ findings might be because they performed their measurements at different temperatures, or because their samples had different amounts of disorder and distinct geometries. Either way, even in the absence of seeing an exciton condensate, the many unexplained observations by both groups point to new physics to be discovered and understood in strongly interacting double bilayer-graphene systems.
This research is published in Physical Review Letters.
References
- Y. E. Lozovik, and V. I. Yudson, “Feasibility of Superfluidity of Paired Spatially Separated Electrons and Holes; A New Superconductivity Mechanism,” JETP Lett. 22, 274 (1975).
- J. I. A. Li, T. Taniguchi, K. Watanabe, J. Hone, A. Levchenko, and C. R. Dean, “Negative Coulomb Drag in Double Bilayer Graphene,” Phys. Rev. Lett. 117, 046802 (2016).
- K. Lee, J. Xue, D. C. Dillen, K. Watanabe, T. Taniguchi, and E. Tutuc, “Giant Frictional Drag in Double Bilayer Graphene Heterostructures,” Phys. Rev. Lett. 117, 046803 (2016).
- J. Kasprzak et al., “Bose–Einstein Condensation of Exciton Polaritons,” Nature 443, 409 (2006).
- J. P. Eisenstein and A. H. MacDonald, “Bose–Einstein Condensation of Excitons in Bilayer Electron Systems,” Nature 432, 691 (2004).
- A. A. High, J. R. Leonard, M. Remeika, L. V. Butov, M. Hanson, and A. C. Gossard, “Condensation of Excitons in a Trap,” Nano Lett. 12, 2605 (2012).
- H. Min, R. Bistritzer, J.-J. Su, and A. H. MacDonald, “Room-Temperature Superfluidity in Graphene Bilayers,” Phys. Rev. B 78, 121401 (2008).
- M. Y. Kharitonov and K. B. Efetov, “Electron Screening and Excitonic Condensation in Double-Layer Graphene Systems,” Phys. Rev. B 78, 241401 (2008).
- A. Perali, D. Neilson, and A. R. Hamilton, “High-Temperature Superfluidity in Double-Bilayer Graphene,” Phys. Rev. Lett. 110, 146803 (2013).
- J. C. W. Song and L. S. Levitov, “Energy-Driven Drag at Charge Neutrality in Graphene,” Phys. Rev. Lett. 109, 236602 (2012); R. V. Gorbachev et al., “Strong Coulomb Drag and Broken Symmetry in Double-Layer Graphene,” Nature Phys. 8, 896 (2012).
- An additional unusual observation is that the giant drag signal is not symmetric upon switching the roles of the drag and drive layers (Onsager reciprocity). This puzzling anomaly has also been reported in electron-hole systems in GaAs double quantum wells at low temperatures, see A. F. Croxall, K. Das Gupta, C. A. Nicoll, M. Thangaraj, H. E. Beere, I. Farrer, D. A. Ritchie, and M. Pepper, “Anomalous Coulomb Drag in Electron-Hole Bilayers,” Phys. Rev. Lett. 101, 246801 (2008); J. A. Seamons, C. P. Morath, J. L. Reno, and M. P. Lilly, “Coulomb Drag in the Exciton Regime in Electron-Hole Bilayers,” 102, 026804 (2009).