Cactus Patterns Buckle Up
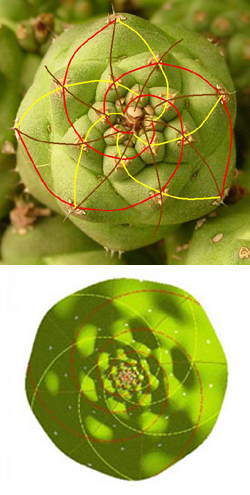
The intricate spiral patterns displayed in cacti, pinecones, sunflowers, and other plants often encode the famous Fibonacci sequence of numbers: 1, 1, 2, 3, 5, 8, … , in which each element is the sum of the two preceding numbers. Now a mathematical model published in the 23 April PRL suggests that these spiral patterns, and the Fibonacci relationships among the spirals, arise out of simple mechanical forces acting on a growing plant.
The round head of a cactus is covered with small bumps, each containing one pointy spike, or “sticker.” For some cacti, you can start at the center and “connect the dots” from each sticker to a nearest neighbor to create a spiral pattern containing 3, 5, or 8 branches. These are three consecutive numbers from the Fibonacci sequence. Other cacti, sunflowers, and pinecones display this or other triples of Fibonacci numbers.
One theory for these patterns is that they are driven by mechanics. New leaves on a plant emerge from a rounded growing tip that consists of an outer shell covering a squishy core. As the plant grows, the theory goes, the shell grows faster than the core, so spiral ridges form in the shell to accommodate the extra surface area, just as wrinkles form on skin when there is more skin than the flesh below requires. Where different sets of ridges intersect, they generate hills and valleys as they reinforce one another and cancel each other out. On a cactus, these hills become the locations for stickers. Although biologists have some experimental evidence for the theory, no one has shown exactly how a plant’s internal forces could generate the patterns.
To test the idea, mathematicians Patrick Shipman and Alan Newell of the University of Arizona in Tucson created a mathematical model of cactus growth that takes into account the elastic properties and stresses on the plant’s growing tip. The pair then computed the buckling patterns that are most stable.
Newell says the stable configurations have precisely three families of spiral waves, with spirals from all three families intersecting at each sticker. Once formed, the spirals tend to reinforce each other and suppress any other arrangement. The Fibonacci relationship then comes from geometry: The three sets of spirals divide the plant’s surface into triangles with curved sides, and according to textbook math, a surface “tiled” with such triangles has special properties. Of the three sets of spirals that form the triangles’ borders, the number of branches in two of the sets must add up to the number in the third.
Shipman cautions that not all sets of numbers with this additive relationship are members of the Fibonacci sequence, but the relationship does make the Fibonacci numbers plausible in plants. In computer simulations, the team produced patterns almost identical to those found in living cacti.
The work is “a very nice step forward in showing that buckling can play a tremendous role in pattern formation,” says Charles Steele, a mechanical engineer at Stanford University in Palo Alto, California, who has studied pattern formation in sunflowers. Jacques Dumais, a biologist at Harvard University, says models like Shipman and Newell’s are valuable to biologists provided that they suggest specific experiments, rather than simply producing pretty pictures of plant-like patterns. In response, Shipman says his model makes testable predictions about the thickness of the outer shell and other material properties of the plant.
–Erica Klarreich
Erica Klarreich is a freelance science writer in Berkeley, CA.
More Information
interactive web site with pictures of plant spirals


