Nobel Prize–Discovery of Quasicrystals

Don Monroe

Focus

Nobel Prize–Discovery of Quasicrystals

October 7, 2011• Phys. Rev. Focus 28, 14

The 2011 Nobel Prize in Chemistry recognizes the discovery of quasicrystals, in which atoms are ordered over long distances but not in the periodically repeating arrangement of traditional crystals.

Researchers have always assumed that every crystalline arrangement of atoms must have a pattern that repeats perfectly in all directions. But the 2011 Nobel Prize in Chemistry recognizes the discovery of a whole new category of crystals whose patterns don’t repeat in the traditional way. Daniel Schechtman of the Technion–Israel Institute of Technology received the Prize for work he published with collaborators in Physical Review Letters in 1984. The team’s description of the atomic structure of a metal alloy ultimately forced scientists to redefine the term “crystal.”

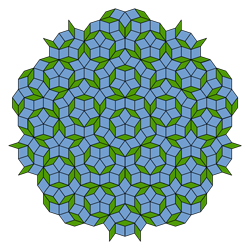
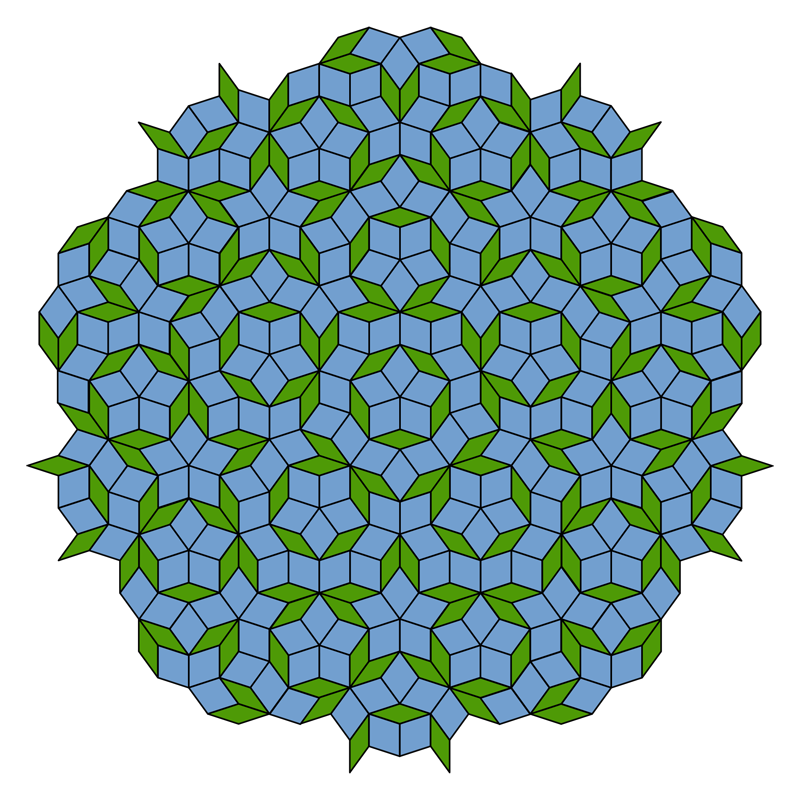
The periodically repeating arrangements of atoms in a crystal are analogous to patterns of tiles that cover a floor without gaps or overlaps. Mathematicians classify these structures by their symmetries: for each pattern, they find the transformations, such as rotations, reflections, and sideways shifts that result in an identical structure. There are some basic geometrical rules for compatible symmetries. For example, an infinite tiling pattern made of repeated equilateral triangles is unchanged by a three-fold rotation around the center of any triangle. But while five-fold symmetry may apply to a single pentagon, it can’t apply to a periodic tiling pattern.

In 1982, Schechtman was using electron diffraction experiments to directly reveal the symmetry and other structural details of metal samples. Working at the National Bureau of Standards (now the National Institute of Standards and Technology) in Gaithersburg, Maryland, he discovered a rapidly cooled alloy of aluminum and manganese that showed the forbidden five-fold symmetry. The surprising symmetry appeared in one direction, in which his data showed electron diffraction spots arranged in concentric rings with ten spots each, whereas in other directions, the rings contained six spots, indicating a conventional six-fold symmetry. Overall, the symmetry of the diffraction pattern was exactly that of an icosahedron.

Researchers knew that icosahedral arrangements of atoms readily occur in tight-packed metal structures. But they also knew that this symmetry, with its five-fold axis, was strictly forbidden for any periodic crystal. It took two years before the paper was published, as Schechtman and his colleagues did careful checks to rule out other possibilities, for example, that the unexpected spots could have come from crystalline regions with different orientations. In the end, they showed that the icosahedral symmetry extended over distances of microns, thousands of times larger than the atomic spacing.

Within six weeks after the publication, Dov Levine and Paul Steinhardt, then at the University of Pennsylvania in Philadelphia, published another paper in Physical Review Letters that resolved the mystery of a crystal with five-fold symmetry and introduced the term “quasicrystal” [1]. The icosahedral symmetry was permitted, they said, as long as the structure was only “quasi-periodic.” For example, if a pattern contains two elements that repeat with different periods, and the ratio of those periods is irrational, they will never “synchronize,” even over very long distances. Because they do not quite repeat, such patterns can evade the usual prohibitions on certain rotational symmetries.

Roger Penrose of Oxford University in England had toyed with such patterns in the 1970s, and the same mathematical rules appear in Islamic architecture from centuries earlier. But no one expected to see them appear in a real material, especially since they seemed to require painstaking construction of the whole, infinite pattern all at once. Subsequent researchers showed that quasiperiodic structures could be assembled atom-by-atom by following only the sort of local rules that must govern the growth of real crystals.

In spite of their unique atomic arrangement, quasicrystalline materials don’t behave very differently than traditional, related materials, although electrons scatter in different ways, which can affect their electrical and thermal resistivity. Instead, their major impact is mathematical. “It opened our minds to thinking about crystallinity in a new way,” says Peter Lu of Harvard University.

–Don Monroe

Don Monroe is a freelance science writer in Murray Hill, New Jersey.