The rules of disorder
Graphene, a single atomic layer of carbon, is effectively a sandbox for studying electrons in two dimensions. Much behavior familiar from other two-dimensional systems, such as the anomalous quantum Hall effect, also occurs in graphene. Researchers are intrigued by the extent to which graphene’s properties result from its peculiar band structure, the so-called Dirac cone.
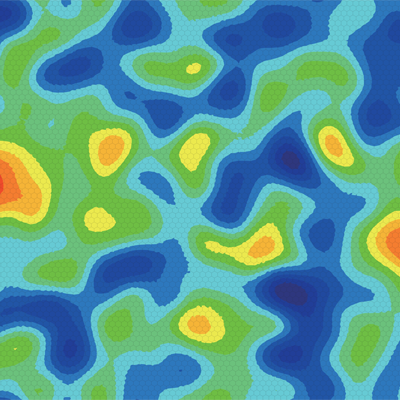
In an article appearing in Physical Review Letters, Tohru Kawarabayashi, Yasuhiro Hatsugai, and Hideo Aoki at Toho University, the University of Tsukuba, and the University of Tokyo, respectively, study theoretically how disorder may affect the behavior of Landau levels in graphene. Taking into account certain symmetries arising from graphene’s honeycomb lattice structure [1], they find that the quantum Hall plateau transition becomes extraordinarily sharp in the lowest Landau level for significantly strong bond disorder, as long as it is spatially correlated over a few lattice constants. The same does not hold for higher Landau levels. This behavior is not generally seen with random Dirac fermions, and is thus attributed to the symmetry properties peculiar to graphene. The authors argue that this effect should be visible in otherwise clean graphene where disorder arising from ripples in the two-dimensional sheet is spatially correlated. – Sami Mitra
[1] Y. Hatsugai, Phys. Rev. B 74, 205414 (2006).