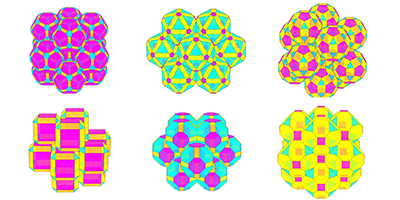
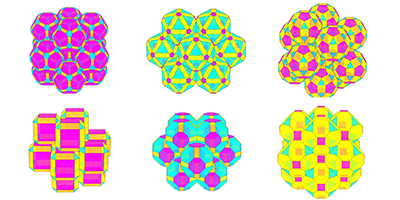
Packing Polyhedra
Since the 17th Century, when Johannes Kepler first studied how to pack perfect spheres into the tightest possible configuration, mathematicians have analyzed the packing properties of increasingly complex shapes. But this “packing problem” is notoriously difficult, and it is challenging to predict the packing density of one shape even if that of a similar shape is known. Elizabeth Chen at Harvard University, Daphne Klotsa at the University of Michigan, Ann Arbor, and colleagues have now expanded the study of particle packing to shapes, enabling a more detailed investigation of how shape affects packing. Reporting in Physical Review X, the team finds that minor shape deformations can have a significant effect on packing density. The result could have applications in nanotechnology and biology, where high packing densities are often required.
Chen, Klotsa, and their colleagues generated the multitude of shapes by using computers to interpolate between symmetric solids such as cubes, tetrahedrons, octahedrons ( faces), dodecahedrons ( faces), and icosahedrons ( faces). Using analytical and computational methods, they investigated the densest possible packing arrangements of each shape in an infinite, periodic three-dimensional box.
A surprising finding of the research is how sensitive the packing density is to certain small changes in shape. For example, truncating the edges of a dodecahedron results in a decreased packing density, but truncating the vertices hardly affects the packing density at all. The team concludes that rather than focus on a specific shape, future studies of packing properties should instead examine families of shapes related by small deformations. – Katherine Kornei