A New Twist on Relativistic Electron Vortices
Vortices are rotational currents around a stagnant point. They can appear in everyday contexts, like a whirlpool in a river. They can also form in a purely quantum entity like a free electron, where what rotates is the probability-density current associated with the particle’s quantum-mechanical wave function. Electron vortices have several applications: they can be used to probe nanoscale magnetic materials and to manipulate nanoparticles. However, a fundamental question is whether a vortex can be formed from an electron at relativistic energies. Two papers, one by Iwo Bialynicki-Birula from the Center for Theoretical Physics in Warsaw, Poland, and Zofia Bialynicka-Birula from the Institute of Physics, also in Warsaw [1], the other by Stephen Barnett from the University of Glasgow, UK [2], address this question by developing a mathematical framework to describe relativistic electron vortices. Their approaches are different, and they reach differing opinions about whether a true vortex can be made from a relativistic electron, as experiments have claimed.
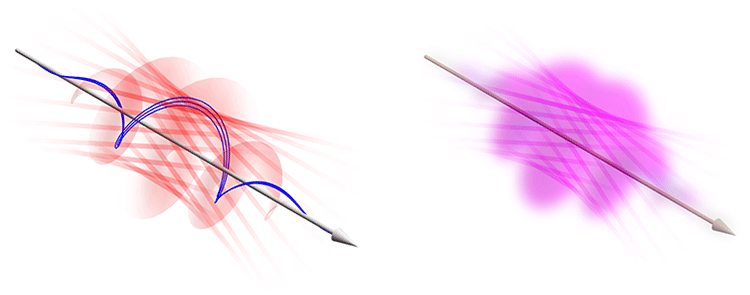
An electron vortex is essentially a sum of electron plane waves, or a wave packet, with a special shape: a central null point surrounded by a coiling probability-density current. For nonrelativistic electrons, the vortex can be made with a wave packet that has a helical wave front and thus appears to spiral like a corkscrew (Fig. 1, left). In general, wave fronts consisting of ℓ intertwined helices, where ℓ is an integer, are said to have a vortex of strength ℓ. In most (but not all) cases, the helical wave front can be directly related to the orbital angular momentum carried by the electron [3]. Moreover, the orbital and spin angular momentum of nonrelativistic electrons are independently conserved because the two momenta are not coupled in the Hamiltonian that describes free electrons. This means that an electron vortex can be described as a product of two wave functions, one that carries information about the electron’s orbital angular momentum and defines the vortex, and a second that carries information about the electron’s spin.
The formalism for describing nonrelativistic electron vortices was established in 2007 [4]. This led to a surge in experimental efforts to generate electron vortex beams, with researchers using devices such as spiral phase plates [5] and pitchfork holograms [6, 7] to impart a helical wave front, and thereby a nonrelativistic vortex, on the electron wave packet. These (ultimately successful) efforts were largely motivated by an interest in using the beams as nanoscale magnetic probes [8]. But they also prompted other ideas, such as using the electron’s orbital angular momentum to generate spin-polarized electron beams [9] and employing the electron’s coiling probability-density currents to nondestructively measure its orbital angular momentum [10].
Our discussion so far pertains to electron wave packets built from wave functions that are solutions to the Schrödinger equation, which only describes nonrelativistic particles. To describe relativistic electron vortices, one must instead turn to the considerably more difficult-to-solve Dirac equation. Researchers first attempted to do this in 2011 [11], finding that the vortex of a relativistic electron has new features compared to that of its low-speed counterpart. Namely, because spin is directly built into the Dirac equation, there is an intrinsic coupling between the electron’s spin and its orbital angular momentum, and these two quantities are no longer separately conserved. However, a limitation of this early work with relativistic electron vortices was that it entailed expressing the electron wave functions in terms of Bessel functions, which extend to infinity and don’t reflect the finite nature of real electrons.
In the two new papers, the Bialynicki-Birula duo and Barnett go beyond previous work by looking for finite wave packet vortex solutions to the Dirac equation. But exactly how they do this varies between the two studies. The Bialynicki-Birula pair constructed helical solutions to the Klein-Gordon equation, a relativistic extension of the Schrödinger equation that is simpler to solve than the Dirac equation. The researchers then transformed these solutions into spinor wave functions, which they assembled to form solutions to the Dirac equation. Once they had these solutions, they derived expressions with helical wave fronts, which they used to construct finite wave-packet states that converge to standard vortex wave packets [4] in the nonrelativistic limit. In contrast, Barnett used the so-called Foldy-Wouthuysen transformation of the Dirac equation, which describes the electron in its rest frame. Following a series of fairly common approximations, he arrived at a form of the equation that could be more easily solved to find an expression for the electron vortices. He then transformed this expression so that it could be represented in an observer’s rest frame. In addition, he transformed the spin and orbital angular momentum operators to the observer’s rest frame and found two angular-momentum-like quantities that are separately conserved.
The wave packets described in the two papers both have the quintessential structure of vortices. And in agreement with [11], they both display forms of spin-orbit coupling. Moreover, they also have a coiling probability-density current about the wave’s propagation axis, thus confirming the presence of an entity similar to a vortex. However, Bialynicki-Birula and Bialynicka-Birula additionally calculated the electron’s vorticity along the propagation axis—an indicator of the vortex’s strength—and found that the spin and orbital contributions to the vorticity canceled one another out. In simpler terms, this means there is no null point in the center of the vortex. As such, they question whether it’s possible to construct vortices from relativistic electrons. If correct, their conclusions might affect the interpretation of experiments that have used vortices made from relativistic electrons.
Regardless of which study [1, 2] presents the right picture, the new results aren’t likely to affect practical applications of electron vortices. That’s because applications primarily rely on the strength of the coiling probability-density current in the wave packet, and both papers agree that this can still be high at relativistic energies. Regardless of the practical implications, the question of whether it’s possible to make relativistic electron vortices is of fundamental interest, as it surrounds the nature of vortices themselves. This in itself could motivate experimental works to expand on the claims of both papers.
This research is published in Physical Review Letters.
References
- I. Bialynicki-Birula and Z. Bialynicka-Birula, “Relativistic Electron Wave Packets Carrying Angular Momentum,” Phys. Rev. Lett. 118, 114801 (2017).
- S. M. Barnett, “Relativistic Electron Vortices,” Phys. Rev. Lett. 118, 114802 (2017).
- M. V. Berry, “Paraxial Beams of Spinning Light,” Proc. SPIE Int. Conf. on Singular Optics 3487, 6 (1998).
- K. Y. Bliokh, Y. P. Bliokh, S. Savel’ev, and F. Nori, “Semiclassical Dynamics of Electron Wave Packet States with Phase Vortices,” Phys. Rev. Lett. 99, 190404 (2007).
- M. Uchida and A. Tonomura, “Generation of Electron Beams Carrying Orbital Angular Momentum,” Nature 464, 737 (2010).
- J. Verbeeck, H. Tian, and P. Schattschneider, “Production and Application of Electron Vortex Beams,” Nature 467, 301 (2010).
- B. J. McMorran, A. Agrawal, I. M. Anderson, A. A. Herzing, H. J. Lezec, J. J. McClelland, and J. Unguris, “Electron Vortex Beams with High Quanta of Orbital Angular Momentum,” Science 331, 192 (2011).
- J. Harris, V. Grillo, E. Mafakheri, G. C. Gazzadi, S. Frabboni, R. W. Boyd, and E. Karimi, “Structured Quantum Waves,” Nat. Phys. 11, 629 (2015).
- E. Karimi, L. Marrucci, V. Grillo, and E. Santamato, “Spin-to-Orbital Angular Momentum Conversion and Spin-Polarization Filtering in Electron Beams,” Phys. Rev. Lett. 108, 044801 (2012).
- H. Larocque, F. Bouchard, V. Grillo, A. Sit, S. Frabboni, R. E. Dunin-Borkowski, M. J. Padgett, R. W. Boyd, and E. Karimi, “Nondestructive Measurement of Orbital Angular Momentum for an Electron Beam,” Phys. Rev. Lett. 117, 154801 (2016).
- K. Y. Bliokh, M. R. Dennis, and F. Nori, “Relativistic Electron Vortex Beams: Angular Momentum and Spin-Orbit Interaction,” Phys. Rev. Lett. 107, 174802 (2011).