Microscopic Theory for Peeling Tape
Peeling packing tape is often a struggle—first the tape refuses to budge; then it unspools too far. Researchers have learned that even when tape rolls out steadily, it continues to stop and start at high frequency on the microscopic scale. Now researchers have developed a theory—based on observations of the peeling of adhesive tape—that explains how energy provided by the peeling force is converted to kinetic energy in the moving tape. Characterizing this effect could lead to a reduction in the noise produced when peeling tape (a serious concern for industry, where the sound can be deafening) as well as improvements for future adhesive tapes.
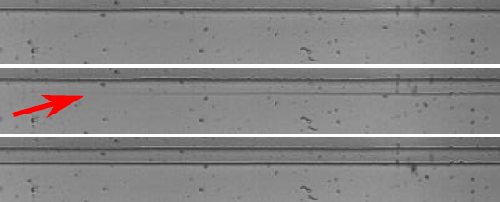
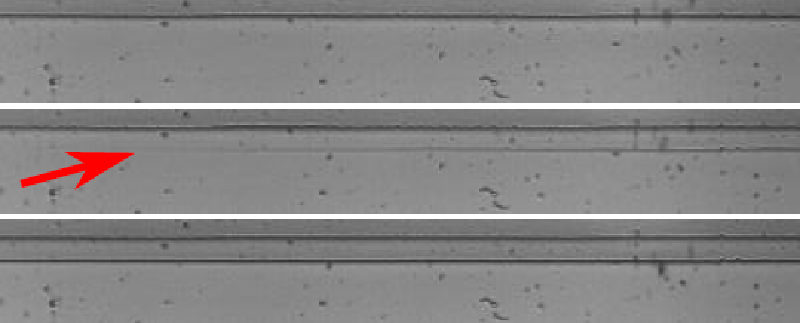
Adhesive tapes are used in thousands of industries and are continually improved, but researchers still do not fully understand how they operate at the microscopic level, including the peeling process. When you peel tape from a roll, it may start and stop every few millimeters, a phenomenon known as macroscopic stick-slip. But in 2010, researchers using a high-speed camera to observe tape peeled from a surface discovered that within each millimeter-scale slip, there are many so-called micro-stick-slip events separated by a few hundred micrometers or less [1]. The researchers also found that the separation front—the line dividing the attached tape from the separated tape—is not a straight line during the microslips. Instead, the separation advances in each microslip as a wave moving rapidly across the width of the tape, perpendicular to the main direction of peeling. As seen from above, this sideways separation wave produces a moving kink in the otherwise straight-line front.
In 2015, Stéphane Santucci of the École Normale Supérieure in Lyon, France, and his colleagues found that these micro stick-slips were controlled by a release of bending energy in the tape near the separation front [2]. But the researchers did not fully explain the mechanism driving the microslips.
In their latest research, Santucci and his colleagues reprised their previous technique, in which a motor peels one layer of Scotch tape off of another layer that is stuck down on a transparent surface. The researchers varied several parameters, such as the peeling angle and velocity and the tape’s bending stiffness. A high-speed video camera mounted on a microscope recorded the peeling.
The team measured the time between microslips and the distance the separation front advanced with each slip. They found that the longer the duration of the “stick,” the more distance was covered during the following slip. “The more you wait, the longer jumps should be,” says Santucci. “During this time you’re storing elastic energy in the bending of the ribbon.” But the relationship between these two quantities wasn’t a direct proportionality: the slip distance was proportional to the cube root of the time between slips.
The researchers propose a theoretical model for the micro-stick-slip process and compare it with their data. In their model, elastic potential energy is stored in the adhesive and the bend of the tape—both act like springs that store energy as they are stretched. At the moment of slip, pent up potential energy is converted to kinetic energy. This description of the energy balance leads to an equation showing the cube-root relationship between the microslip distance and duration. The equation also includes the effects of the tape’s bending stiffness and mass density, which agree with the team’s experiments comparing single- and multilayer tapes. Additionally, the model predicts that the rapid waves that travel sideways—which the team calls crack fronts—propagate at 650–900 meters per second, in agreement with the data.
Santucci and his colleagues draw parallels between the motion of the tape separation front and the propagation of cracks in materials because both create new surfaces as they progress. This analogy makes sense, says Jay Fineberg, a soft matter physicist at the Hebrew University of Jerusalem. “I’m continually surprised that a diverse range of seemingly unrelated questions in physics turn out to be quite related to rapid fracture processes,” he says. “These problems range from peeling problems to the basic physics of friction and earthquake propagation.” He suggests that the latest research is “another piece to this rather diverse puzzle” of fracture.
Many questions remain. For example, it’s unclear what causes the sideways crack fronts, Santucci says. “The stick-slip instability is much more complicated than what we originally thought.”
This research is published in Physical Review Letters.
–Dan Garisto
Dan Garisto is a science journalist based in New York City.
References
- S. T. Thoroddsen, H. D. Nguyen, K. Takehara, and T. G. Etoh, “Stick-slip substructure in rapid tape peeling,” Phys. Rev. E 82, 046107 (2010).
- M. Dalbe, P. Cortet, M. Ciccotti, L. Vanel, and S. Santucci, “Multiscale stick-slip dynamics of adhesive tape peeling,” Phys. Rev. Lett. 115, 12830 (2015).