Squeezing down the Theory Space for Cosmic Inflation

Daniel Meerburg

Viewpoint

Squeezing down the Theory Space for Cosmic Inflation

Faculty of Science and Engineering, University of Groningen, Groningen, Netherlands

October 4, 2021• Physics 14, 135

An updated search for primordial gravitational waves has not found a signal, which implies that some popular early Universe models are becoming less viable.

Figure caption — **Figure 1:** An aerial view of the BICEP2 experiment at the South Pole.

Remarkably, the large-scale Universe can be adequately described by a model involving only a handful of parameters. This lambda cold dark matter (LCDM) model postulates that the expansion of the Universe is driven by the presence of two dark components—dark energy and dark matter—and that the galactic structure we observe today was sourced by small density variations in the very early Universe. However, cosmologists expect that these primordial density fluctuations were accompanied by fluctuations in the fabric of spacetime itself. These gravitational waves could be observed through a predicted signal in the cosmic microwave background (CMB). The BICEP/Keck Collaboration, which has been a frontrunner in the search for this illustrious signal, reports on its latest data set, finding no evidence of gravitational waves [1]. The resulting limits further constrain a class of popular model predictions, which suggests we are either closing in on a detection or reaching a crossroads where different inflation models and perhaps alternative scenarios need to be considered. In addition, the analysis shows that researchers properly understand the astrophysical contaminants that obscure the search for this relic signature. By reducing uncertainties about this contamination, we should have greater confidence in any future claims of a detection.

A robust detection of relic gravitational waves would confirm that our Universe was likely shaped by a mechanism known as inflation. Inflation was introduced in the early 1980s to solve some of cosmology’s biggest conundrums, and to this day, it is the most widely accepted and plausible theory of the early Universe. If that theory is correct and gravitational waves filled the early Universe, then cosmologists expect a distinct pattern, called B modes, should be imprinted in the CMB [2]. In 2014, the BICEP experiment at the South Pole (Fig. 1) reported possible evidence of B modes, but the signal turned out to be merely a result of dust in our own Galaxy producing CMB-like radiation. While this was a disappointment, it concentrated efforts on the dust problem (see Hunting Season for Primordial Gravitational Waves).

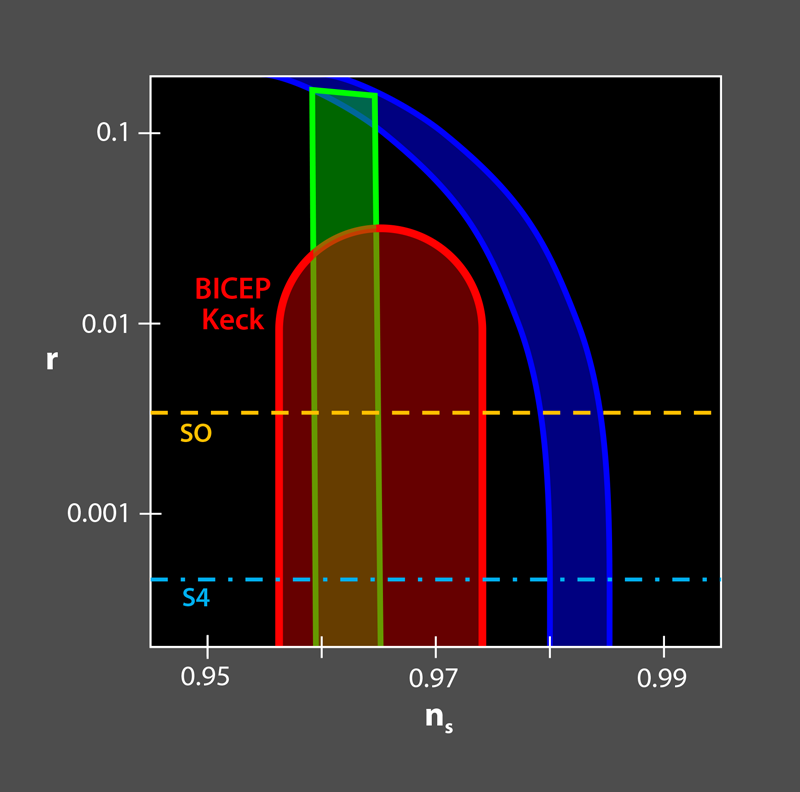
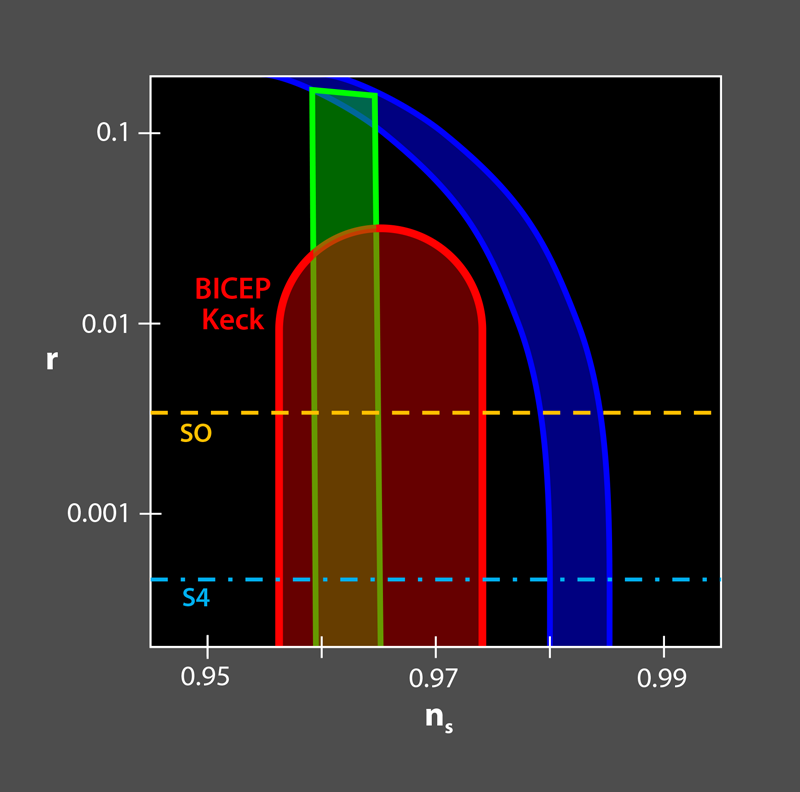
In principle, the CMB and dust can be distinguished because they differ spectrally. Over the years, researchers from the BICEP experiment and a joint project called the Keck Array have developed dust models using data at different frequencies. By removing the estimated dust contribution, the BICEP/Keck Collaboration has been able to place a progressively tightening bound on the gravitational-wave contribution. This bound is typically given in terms of the tensor-to-scalar ratio $r$ , which characterizes the amplitude of gravitational waves relative to that of density waves. Before BICEP/Keck, the tensor-to-scalar ratio was known to be less than 0.11, based on CMB observations by the Planck satellite [3]. With BICEP/Keck data, the bound dropped to $r < 0.09$ in 2016 [4] and then to $r < 0.07$ in 2018 [5]. Earlier this year, an analysis of Planck data pushed the bound lower [6], and now the latest results from BICEP/Keck provide the tightest constraint yet, $r < 0.036$ [1]. The BICEP/Keck team have shrunk this limit by combining data at three frequencies (96 GHz, 150 GHz, and 220 GHz) from their own experiment, complemented by archival data from the WMAP and Planck satellites.

Before commenting on the theoretical implications of this new upper limit, it is important to note how promising the latest analysis is for detecting a signal in the future. Since the foregrounds are the limiting factor to detection, accurate dust modeling is critical. Dust models are primarily based on high-frequency observations by the Planck satellite (the 353-GHz data to be precise). Dust becomes less important at lower frequencies, but for a small tensor-to-scalar ratio $r$ , dust is still the strongest signal on the sky. If dust is more complicated than presently assumed, its modeling would need even more frequencies, or worse, perhaps the dust is too complicated to be modeled at all! For the first time, the observations place constraints on all the parameters of the dust model, and the team’s analysis shows that the model is accurately capturing the behavior of the dust. This is exciting news, as it suggests that we are in good shape when it comes to removing dust contamination and well equipped to make a detection if the signal is large enough.

These experimental efforts on dust removal are paying off, as the new bounds on the tensor-to-scalar ratio are having an impact on inflationary models. Different inflation models make different predictions for $r$ , as the ratio is tied to the energy scale of inflation. One way to compare models with data is in a $n_{s}$ vs $r$ plot, where $n_{s}$ is the scale dependence of the density fluctuations coming out of the inflation epoch (Fig. 2). The value that $r$ takes within a particular inflation model is partly dependent on the amount of expansion before the end of inflation, characterized by the number $N$ of $e$ -folds (one $e$ -fold corresponds to a stretching of space by a factor of $e \approx 2.71$ ). To solve the cosmological conundrums that inflation was designed to solve, $N$ has to be at least 40, while values larger than 60 are inconsistent with measurements of $n_{s}$ . For a large class of popular models broadly referred to as monomial or power-law models, the tensor-to-scalar ratio is relatively large and scales as $1 ∕ N$ [7]. We can now comfortably start to rule out this class of models. For another class of inflationary models, inspired by the work of Alexei Starobinsky in the early 1980s [8], the tensor-to-scalar ratio scales as $1 ∕ N^{2}$ , leading to a smaller value of r that is within the new bounds from BICEP/Keck. Other models exist having $r \propto 1 ∕ N^{t}$ with $t > 2$ ; however, those models tend to predict a value of $n_{s}$ inconsistent with current bounds.

So, some inflation models remain viable, but most of the popular models predict $r > 1 0^{- 4}$ . While the new BICEP/Keck bound is still over 2 orders of magnitude away from this threshold, the rapid improvement over the last few years, combined with a much better understanding of the foregrounds, suggest we should eventually reach this theoretically well-motivated threshold. The future thus looks bright. Besides continued efforts by BICEP/Keck in close collaboration with the South Pole Telescope (SPT), the Simons Observatory (SO) in Chile, and later on, the CMB-S4 experiment (a joint effort by the SO and SPT/BICEP groups) will guarantee that we will reach the experimental sensitivity needed to measure $r$ as low as $1 0^{- 3}$ perhaps before the end of this decade. In addition to these ground-based experiments, a Japanese-led satellite mission, called LiteBIRD, will be launched in 2028. Satellites can observe the largest scales in our Universe, allowing them to probe a slightly later epoch than possible with ground observatories. If a detection is made, the combination of ground and space experiments will be paramount for confirmation. If, however, future measurements continue to find no gravitational waves, we will need to abandon some of the most popular models for inflation and consider further modifications [9]. And while the inflationary paradigm cannot be falsified from these observations alone, a non detection could contribute to a paradigm shift towards noninflationary models [10].

Corrections (5 October 2021): (1) The original text suggested that the most recent update to the $r$ bounds was in 2018 [5], when in fact there was an update in March 2021 [6]. This latter reference was added as a correction. (2) Some of the wording about the experimental sensitivity for $r$ has been corrected. Reaching the theoretical threshold $1 0^{- 4}$ will not happen “soon,” as indicated in the original text. However, experimental sensitivity should reach $1 0^{- 3}$ before the end of this decade.

Correction (8 October 2021): The text was modified to clarify that inflation cannot be falsified through $r$ measurements alone and to acknowledge inflationary models that predict lower values of $r$ than monomial and Starobinski-inspired models.

Correction (20 October 2021): Figure 2 was updated to provide an accurate visualization of the viable parameter space for Starobinsky-inspired models.

References

P. A. R. Ade et al. (BICEP/Keck Collaboration), “Improved constraints on primordial gravitational waves using Planck, WMAP, and BICEP/Keck observations through the 2018 observing season,” Phys. Rev. Lett. 127, 151301 (2021).
M. Kamionkowski et al., “A Probe of Primordial Gravity Waves and Vorticity,” Phys. Rev. Lett. 78, 2058 (1997).
P. A. R. Ade et al. (Planck Collaboration), “Planck 2013 results. XXII. Constraints on inflation,” Astron. Astrophys. 571, A22 (2014).
P. A. R. Ade et al. (Keck Array and BICEP2 Collaborations), “Improved constraints on cosmology and foregrounds from BICEP2 and Keck Array cosmic microwave background data with inclusion of 95 GHz band,” Phys. Rev. Lett. 116, 031302 (2016).
P. A. R. Ade et al. (Keck Array and BICEP2 Collaborations), “Constraints on primordial gravitational waves using Planck , WMAP, and new BICEP2/Keck observations through the 2015 season,” Phys. Rev. Lett. 121, 221301 (2018).
M. Tristram et al., “Planck constraints on the tensor-to-scalar ratio,” Astron. Astrophys. 647, A128 (2021).
V. Mukhanov, “Quantum cosmological perturbations: predictions and observations,” Eur. Phys. J. C 73, 2486 (2013).
A. A. Starobinsky, “A new type of isotropic cosmological models without singularity,” Phys. Lett. B 91, 99 (1980).
R. Kallosh and A. Linde, “B-mode targets,” Phys. Lett. B 798, 134970 (2019).
J. Khoury et al., “Ekpyrotic universe: Colliding branes and the origin of the hot big bang,” Phys. Rev. D 64, 123522 (2001).

About the Author

Daniel Meerburg obtained his Ph.D. from the University of Amsterdam in 2011. After several years of postdoc study at Princeton University, the University of Toronto, and the University of Cambridge, UK, he started a faculty job at the University of Groningen, Netherlands, in 2019. He was part of the Planck team and currently has a leading role in next-generation ground-based CMB experiments, including the Simons Observatory and CCATp. He is also involved in REACH, a global 21-cm experiment. His research focuses on the interplay between theoretical and observational cosmology, with a strong emphasis on the cosmic microwave background.