Disturbing the Fermi Sea with Rydberg States
Currently, one of the best ways to model complex quantum systems is through atomic quantum simulations. Controlling interactions between atoms is key to such simulations, something that can be achieved in atomic lattices using the well-established “Feshbach-resonance” approach. While that approach can be used to vary the strength of short-range interactions between atoms, it does not carry over to long-range interactions, leaving some interesting quantum systems outside of the technique’s scope. Elmer Guardado-Sanchez at Princeton University and colleagues have now shown that such long-range interactions can be controlled using “Rydberg dressing” in a lattice of lithium ( 6Li) atoms [1]. The team’s demonstration opens up unprecedented opportunities for exploring systems that exhibit rich fermionic many-body physics.
In the Feshbach-resonance approach to interaction control, a variable magnetic field is used to tune the scattering dynamics of colliding atoms. The use of this technique has led to the experimental observation of the crossover between the Bose-Einstein-condensation (BEC) regime—in which strongly interacting fermions form bosonic molecules—and the Bardeen-Cooper-Schrieffer (BCS) regime—in which weakly interacting fermions form loosely bound Cooper pairs. Quantum phenomena that can be simulated using such interactions range from the electron correlations behind high-temperature superconductors to the quantum kinematics taking place in distant neutron stars. Despite this versatility, there remains an important class of systems beyond the reach of simulations based on local interactions. Those systems are ones composed of spinless fermions, which the Pauli exclusion principle forbids from sitting on top of one another, making local interactions largely irrelevant. Instead, it is the long-range interactions that must be controlled.
One way to engineer such long-range interactions between spinless atomic fermions is to excite the atoms to Rydberg states, in which an electron occupies a high orbital. This method has been proposed theoretically as a way to mediate correlated topological density waves within a fermionic system [2]. Guardado-Sanchez and colleagues now employ the technique experimentally, which they do with an ensemble of spinless, fermionic 6Li atoms.
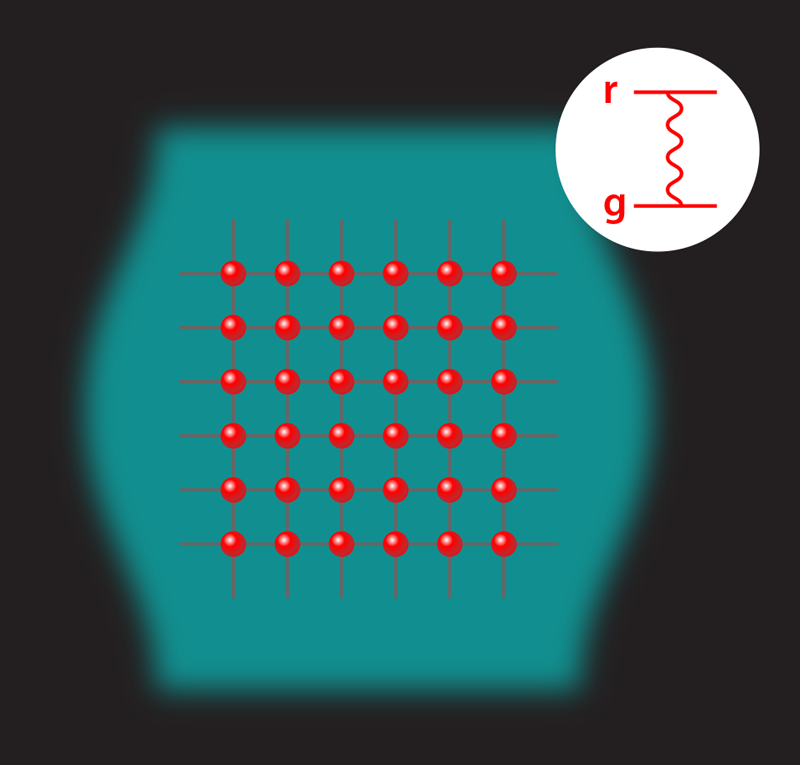
The team cooled a dilute gas of 6Li atoms in an optical lattice to a “quantum degenerate” temperature, one where each atom’s de Broglie wavelength becomes larger than the interatomic spacing. Unable to reach the ground state simultaneously (because of the Pauli exclusion principle), the atoms “freeze” one by one at the lowest momentum available, forming a “Fermi sea” (Fig. 1). In this “sea” state, the atoms barely interact, and there are both minimal thermal and minimal quantum fluctuations.
The team’s next step was to use a laser to implement a Rydberg dressing scheme, which mixes the system’s internal ground state with a highly excited Rydberg state. An atom in a Rydberg state exhibits a larger electric dipole moment than one in the ground state because of the greater distance between its ion core and its outermost electron. This dipole-moment enhancement produces an effective “soft-core interaction” between Rydberg-dressed atoms, meaning that the interaction strength remains roughly constant as the interparticle distance increases, before dropping off above a threshold length scale [2–4]. The researchers show that they can manipulate the strength and the range of this interaction by varying the intensity and frequency of the laser. Although the Rydberg-dressing-induced interaction is isotropic across the two-dimensional system, the motion (by quantum tunneling) of the fermions is restricted to one dimension. This limited freedom of motion hinders the infamous “Rydberg-avalanching-loss” process by which Rydberg atoms collide, gain kinetic energy, and escape the trap.
The long-range interaction and the consequent hopping motion of the fermions generate many-body excitations—commonly called quantum fluctuations—on top of the Fermi sea. These collective quantum fluctuations can have tremendously rich features, yielding many kinds of quantum-correlated states of matter. The types of phenomena that arise in such a system of interacting fermions depend on the way in which the fermions “pair up,” or, more precisely, on the momenta of the participating fermions and the Cooper pairs that result. These momentum-dependent interactions, in turn, are governed largely by the range of the interaction relative to the lattice spacing. A soft-core interaction with a tunable length, such as that realized by Guardado-Sanchez and colleagues, could lead to abundant momentum-dependent behaviors, generating, for example, topological density waves [2] and chiral p+ip superfluidity [5]. Such p+ip superfluids support topological Majorana vortices and offer a plausible route toward realizing topological quantum computation.
Even more exotic and counterintuitive phenomena may arise when different pairing possibilities occur simultaneously. For example, although mean-field theories typically predict that superfluidity appears in the presence of purely attractive interactions, functional renormalization group calculations suggest that a complex combination of different fermion pairings should generate unconventional “f-wave” superfluidity even with atomic repulsion [6]. Guardado-Sanchez and colleagues have so far only demonstrated attractive interactions, but tuning from attraction to repulsion is experimentally feasible [7]. Interesting effects should also arise when the interaction strength completely dominates the kinetic energy, with the system then being driven toward a Wigner crystal or fractional quantum Hall state [8, 9].
In the team’s experiment, with its lattice-hopping fermions, the dynamical aspects of the system are more easily observed than the quantum many-body equilibrium states. Uncovering how to probe such states in a nonequilibrium setting should stimulate future theoretical investigation. On the application side, as well as the above-mentioned potential for topological quantum computing, long-range interaction control is a key step toward performing quantum simulations of quantum chemistry problems. Such simulations represent one arena ripe for applications employing the so-called “quantum advantage” to solve problems that would be intractable using classical computers. One strength of the team’s scheme in realizing applications is that, unlike previously developed Feshbach-resonance techniques, it is magnetic-field-free. This aspect provides extra freedom to integrate the technique with certain magnetic-field-sensitive cold-atom quantum technologies, such as artificial gauge fields.
References
- E. Guardado-Sanchez et al., “Quench dynamics of a Fermi gas with strong nonlocal interactions,” Phys. Rev. X 11, 021036 (2021).
- X. Li and S. Das Sarma, “Exotic topological density waves in cold atomic Rydberg-dressed fermions,” Nat. Commun. 6, 7137 (2015).
- G. Pupillo et al., “Strongly correlated gases of Rydberg-dressed atoms: Quantum and classical dynamics,” Phys. Rev. Lett. 104, 223002 (2010).
- N. Henkel et al., “Three-dimensional roton excitations and supersolid formation in Rydberg-excited Bose-Einstein condensates,” Phys. Rev. Lett. 104, 195302 (2010).
- B. Xiong et al., “Topological superfluid by blockade effects in a Rydberg-dressed Fermi gas,” Phys. Rev. A 90, 013631 (2014).
- A. Keleş et al., “f -wave superfluidity from repulsive interaction in Rydberg-dressed Fermi gas,” Phys. Rev. A 101, 023624 (2020).
- M. Saffman et al., “Quantum information with Rydberg atoms,” Rev. Mod. Phys. 82, 2313 (2010).
- T. Graß et al., “Fractional quantum hall phases of bosons with tunable interactions: From the Laughlin liquid to a fractional Wigner crystal,” Phys. Rev. Lett. 121, 253403 (2018).
- D. N. Sheng et al., “Fractional quantum Hall effect in the absence of Landau levels,” Nat. Commun. 2, 389 (2011).