Steps toward Quantum Gravity in a Realistic Cosmos

Jordan Cotler

Viewpoint

Steps toward Quantum Gravity in a Realistic Cosmos

Society of Fellows, Harvard University, Cambridge, MA, USA

July 18, 2022• Physics 15, 107

Theorists have modeled an expanding spacetime—akin to our Universe—by taking inspiration from a string theory framework in which spacetime is emergent.

Figure caption — **Figure 1:** Three-dimensional de Sitter space is an expanding universe, which can be imagined as a lake whose width expands from a narrow bottom to a wide top. The bottom of the lake corresponds to earlier times when the universe is smaller, and the top of the lake corresponds to later times when the universe is larger. The surface of the lake can be identified with the Wess-Zumino-Witten (WZW) conformal field theory, which describes gravitational fluctuations that are represented as waves. The calculations of Hikida and colleagues establish that a WZW conformal field theory produces correct answers for cosmological entropies.

Experimental discoveries in the late 1990s established that we live in a Universe undergoing accelerating expansion [1]. Our prevailing understanding of cosmology posits that this accelerating expansion is due to empty space possessing an energy, described by a positive cosmological constant. A positive cosmological constant is a major challenge for quantum gravity theorists to understand. They have had more success with the imagined setting of a negative cosmological constant. While quantum gravity models with a negative cosmological constant do not describe our Universe, they do have a surprising feature: the gravity-filled volume of spacetime can be described by a field theory without gravity, often envisioned as a hologram living on a surface. Inspired by this framework, Yasuaki Hikida from Kyoto University in Japan and colleagues studied a simplified model of an expanding universe and found that it also has holographic properties [2]. Their findings may offer a new direction for theorists trying to build models of quantum gravity with a positive cosmological constant consistent with our own Universe.

There are many strategies in quantum gravity for constructing an expanding universe. One approach is to find an appropriate “string compactification”—a type of architectural map for strings and membranes—which builds up a universe with a positive cosmological constant. It is possible to construct nearly stable universes in this manner [3], but these constructions possess features that make them unrealistic. The difficult problem of constructing more realistic model universes is a topic of ongoing and active research.

Another strategy is to generalize the so-called AdS/CFT correspondence [4], which arguably furnishes our best understanding of quantum gravity to date. AdS stands for anti-de Sitter, which is shorthand for a spacetime with constant, negative curvature. Such a spacetime is a solution to Einstein’s equations with a negative cosmological constant. The correspondence stipulates that string theory in an AdS spacetime is (miraculously) equivalent to a theory without gravity in one less spatial dimension.

Appreciating how striking this statement is requires some unpacking. On the one hand, we have a theory of quantum gravity, say, in 3 + 1 dimensions—3 space dimensions and 1 time dimension. On the other hand, we have a so-called “conformal field theory” (CFT) in 2 + 1 dimensions. This CFT does not include gravity; it is a theory that has the basic trappings of the standard model of particle physics but additionally possesses exaggerated symmetries. The AdS/CFT correspondence means that a dimension of space emerges as we toggle between the gravity-free CFT world and the gravity-filled AdS world.

The emergence of a dimension of space is often called “holography,” by analogy with how a 3D image emerges from a 2D hologram. Many physicists have been drawn to studying the AdS/CFT correspondence because it provides a rich model of emergent spacetime. To interrogate the gravity-filled world of AdS, one can calculate specific quantities in the gravity-free world of CFT. Conversely, one can learn properties of CFT via gravity calculations in AdS (see Research News: Holographist by Trade).

It would be compelling to generalize the AdS/CFT correspondence to the setting of a positive cosmological constant, namely to so-called de Sitter (dS) spacetimes. Over the past 20 years, there have been a multitude of proposals attempting such a generalization [5–7]. A feature common to several of the proposals is that time is emergent, instead of space being emergent as per the AdS/CFT correspondence. Emergent time is more difficult to fathom, as it entails the emergence of quantum mechanics itself. Indeed, the basic mathematical structures of quantum mechanics have time ingrained within them. Accordingly, a dS/CFT correspondence—if it were fully realized—would equate quantum gravity in de Sitter spacetime to a non-quantum-mechanical conformal field theory without time.

For AdS/CFT, we have the luxury of numerous explicit constructions. In contrast, there are only a few constructions for dS/CFT (for example, Refs. [8, 9]). Hikida and colleagues have worked toward constructing new examples of a dS/CFT correspondence [2]. They consider the setting of classical Einstein gravity in 2 + 1 dimensions—this spacetime has one spatial dimension less than our own Universe but is often a touchstone in studies of quantum gravity since there are more mathematical tools available to study it. While in our Universe of 3 + 1 dimensions there are localized gravitational waves, gravity in 2 + 1 dimensions has a different character. In 2 + 1 dimensions, there are no localized gravitational waves but there can be gravitational edge modes, which can be thought of as wave-like fluctuations living on or near the “boundary” of the spacetime.

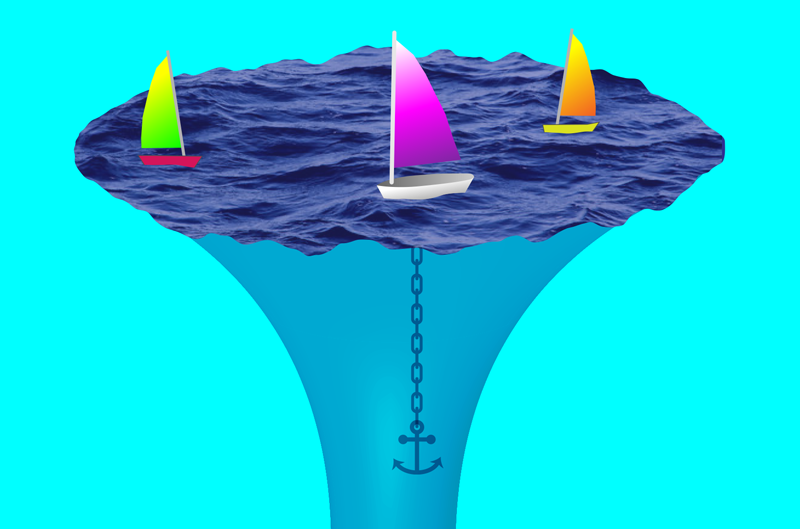
The starting point for understanding the work of Hikida and colleagues is that classical Einstein gravity in three dimensions can be written as a Chern-Simons theory [10]. This is a topological theory that describes fluctuations near the surface of the spacetime. Many Chern-Simons theories are equivalent to conformal field theories known as Wess-Zumino-Witten (WZW) models. While there is no obvious way to create a correspondence between Einstein gravity and a WZW model, Hikida and colleagues ask whether there is a WZW model that reproduces features of 3D de Sitter gravity. We can imagine such a 3D de Sitter world as a lake that starts very narrow at the bottom and then expands in width toward the top (Fig. 1). The surface of the lake would represent a candidate WZW model, and the waves on the lake would be fluctuations in this 2D model.

The researchers focus their attention on “Euclidean” versions of both Einstein gravity and the WZW model in order to study some tractable properties of 3D de Sitter. On the basis of symmetry considerations, Hikida and colleagues look at the family of so-called ${SU(2)}_{k}$ WZW models indexed by a positive integer $k$ . The size of $k$ usually describes the strength of interactions. By pattern-matching to the AdS/CFT correspondence, the researchers infer that $k$ is equal to $- 2$ plus a small imaginary number, which is peculiar since $k$ is supposed to be a positive integer. Undeterred, Hikida and colleagues follow their intuition and show that for this peculiar $k$ , the WZW theory reproduces features of Einstein gravity, such as the entropy of de Sitter space with a particle in it.

These results are surprising. To explore the robustness of their approach, Hikida and colleagues offer several variations of their equations, which reproduce features of more exotic theories of gravity. These analyses provide evidence that 3D de Sitter gravity may be equivalent to an unusual 2D WZW theory, which would instantiate a form of the dS/CFT correspondence. Interestingly, this WZW theory does not satisfy the usual rules of quantum mechanics since it is a nonunitary theory. This feature aligns with the dS/CFT vision that de Sitter quantum gravity is equivalent to a non-quantum-mechanical theory.

At present, most of the rules for de Sitter quantum gravity are waiting to be discovered. This search is an exciting challenge, and Hikida and colleagues have made a creative and interesting contribution. While it remains to be seen whether their particular rules will be incorporated into an ultimate theory of nature, it is clear that we must expand our theoretical horizons to understand quantum gravity in our expanding Universe.

References

P. J. E. Peebles, and B. Ratra, “The cosmological constant and dark energy,” Rev. Mod. Phys. 75, 559 (2003).
Y. Hikida et al., “Holography in de Sitter space via Chern-Simons gauge theory,” Phys. Rev. Lett. 129, 041601 (2022).
S. Kachru et al., “de Sitter vacua in string theory,” Phys. Rev. D 68, 046005 (2003).
J. Maldacena, Int. J. Theor. Phys. 38, 1113 (1999).
A. Strominger, “The dS/CFT correspondence,” J. High Energy Phys. 2001, 034 (2001).
E. Witten, “Quantum Gravity In De Sitter Space,” arXiv:hep-th/0106109.
M. Alishahiha, “The dS/dS Correspondence,” AIP Conf. Proc. 743, 393 (2004).
D. Anninos et al., “Higher spin realization of the DS/CFT correspondence,” Class. Quantum Gravity 34, 015009 (2016).
J. Cotler and K. Jensen, “Emergent unitarity in de Sitter from matrix integrals,” J. High Energy Phys. 2021, 89 (2021).
E. Witten, “2 + 1 dimensional gravity as an exactly soluble system,” Nuclear Phys. B 311, 46 (1988).

About the Author

Jordan Cotler has a B.Sc. in physics and mathematics from the Massachusetts Institute of Technology and a Ph.D. in physics from Stanford University. He is currently a Junior Fellow at the Harvard Society of Fellows and a Fellow at the Harvard Black Hole Initiative. He is interested in nonperturbative aspects of gauge theory and gravity, in particular those pertaining to early universe cosmology and black holes. He also studies quantum information theory and computational complexity theory as applied to developing algorithms for quantum computers as well as exploring underlying information processing in quantum many-body systems.