Symmetrical Binding for Topological States
In a material with a so-called topologically protected state, particles—electrons, photons, or phonons, for example—can move around boundaries in the material without losing energy or being scattered. One way to make a material with such a state is to add into its lattice one or more defects that violate the lattice’s rotational symmetry—for example, disclinations (line defects). However, such defects can break the lattice’s chiral symmetry, which helps protect the topological state. Now, Yun Jing of Pennsylvania State University and colleagues propose and demonstrate a way to ensure chiral symmetry is preserved in the presence of such a defect [1].
When a system has chiral symmetry, its energy spectrum is symmetrical around a “privileged zero frequency.” However, disclinations can move the system away from this zero frequency. Jing and his team found, theoretically, that they could prevent that from happening by making the spectrums of the “cores” of the disclinations symmetric. If they do that, they predict that the system’s chiral symmetry and its topological state will be protected.
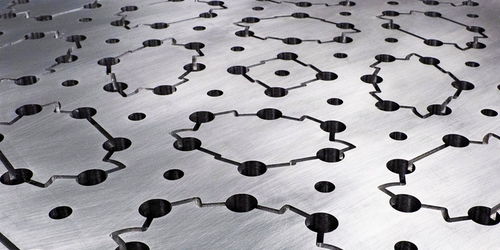
To validate their theory, the team made an acoustic honeycomb lattice using aluminum plates and introduced into it a disclination by removing a 2𝜋∕3 section of a central honeycomb. They then acoustically excited the lattice using speakers and measured its acoustic response using a small microphone. The team found that the peak of the energy spectrum of the disclination core was symmetric about the system’s zero frequency and that the honeycomb lattice maintained its chiral symmetry in the presence of the disclination, confirming their predictions.
–Sarah Wells
Sarah Wells is an independent science journalist based outside of Washington, DC.
References
- Y. Deng et al., “Observation of degenerate zero-energy topological states at disclinations in an acoustic lattice,” Phys. Rev. Lett. 128, 174301 (2022).