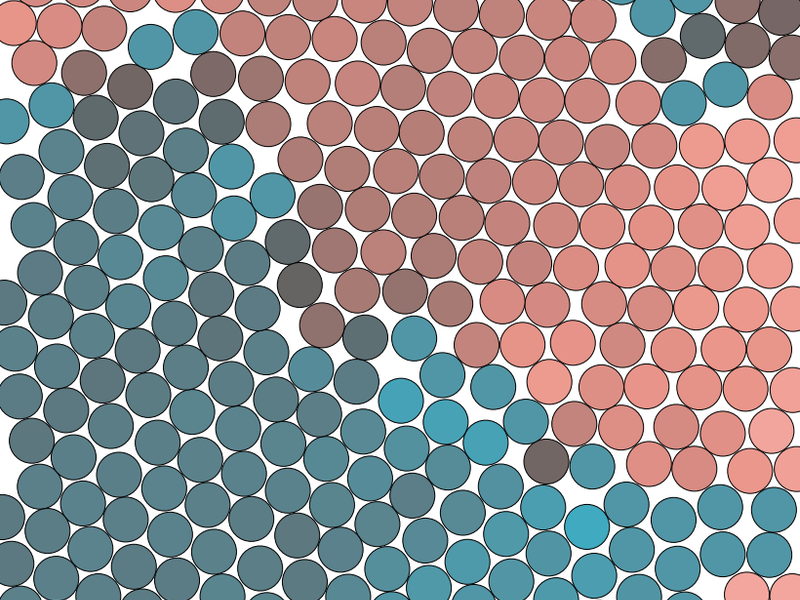
Two-Dimensional Crystal Found in a Nonequilibrium System
Ludovic Berthier of the University of Montpellier, France, was not looking to upend understanding of two-dimensional (2D) crystal formation when he tasked a student with simulating the properties of circular particles in an infinitely large box. But that is exactly what happened when that student—Leonardo Galliano—found a regime where the system formed a solid with perfect, long-range crystalline order, a behavior previously thought impossible for 2D particle systems [1]. Berthier says that while the result is largely of theoretical interest, he hopes that experimentalists will be able to find hints of this 2D ordering, which could have an influence on the behavior of systems ranging from shaken sand grains to moving bacteria. “I would lie if I said that next week someone will produce this behavior in the lab,” Berthier says. “But these predictions raise a challenge that I hope will be taken up.”
In equilibrium, temperature-induced fluctuations squelch any crystal that tries to form in a 2D particle system, with the fluctuations causing particles to jiggle out of their lattice positions just enough that the periodicity of any repeated pattern gets erased. “The particles might look ordered, but if you delve into the [properties] of the system, the long-range order isn’t there,” Berthier says. A mathematical proof forbidding the formation of such crystals backs up this observation. Nonequilibrium systems, however, are a whole different ball game.
In principle, there is no reason that a nonequilibrium 2D particle system can’t crystalize. But just like in their equilibrium counterparts, fluctuations hinder order. In more than three decades of experiments and simulations, no nonequilibrium 2D particle system has been shown to crystalize.
In the new study, Berthier and his colleagues simulate the behavior of circular particles whose motion is determined solely by collisions with their neighbors. The model they use is one commonly employed to simulate nonequilibrium dynamics in granular systems and other macroscopic particle systems. When two particles collide, they each recoil a random distance that relates to the jump sizes of particles undergoing a random walk. This type of motion is analogous to that seen for systems that undergo so-called Brownian motion, which is thermally driven. However, the team’s model does not include temperature-induced fluctuations or any other equilibrium interactions.
The team’s simulations show that when the density of the particles is high enough, the particles crystalize. These crystals have periodic order that remains intact over long times. The team finds that the reason for this order is the absence of any temperature-like fluctuations in the dynamics of the system. “The model kills the long-wavelength fluctuations that melt equilibrium crystals, and so they aren’t there to destroy the long-range order,” Berthier says. “We find this emerging behavior that is otherwise forbidden in an equilibrium system.”
Berthier is cautious about the possibility of the crystal being experimentally realized. He notes, however, that particles with diameters greater than a few micrometers are insensitive to thermal fluctuations. “Colloids, droplets, granular particles could all potentially display this behavior.”
Correction (27 July 2023): The text was updated to clarify the crystal regime found by Berthier and his colleagues. Researchers have previously observed 2D particle systems with quasi long-range order, in which correlation functions have power-law decays—meaning that they become zero at large distances. Here the team finds a nonequilibrium regime that maintains its crystalline order at these large distances. For 2D systems at equilibrium, such behavior is prohibited by the Mermin-Wagner theorem.
–Katherine Wright
Katherine Wright is the Deputy Editor of Physics Magazine.
References
- L. Galliano et al., “Two-dimensional crystals far from equilibrium,” Phys. Rev. Lett. 131, 047101 (2023).