Perfect Cones Are as Weak as They Seem
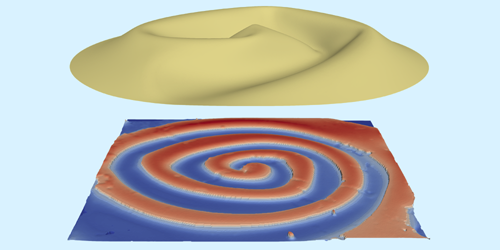
Physicists simplify problems to make calculations easier, but doing so risks neglecting important physical properties, as illustrated by the fabled spherical cow in a vacuum, for example. For decades, oversimplification was thought to explain why thin-walled cones crumple under smaller loads than predicted by theory. Researchers suspected that the descrepancy might result from imperfections in real cones that are absent from theoretically ideal cones. Now Daniel Duffy of the University of Cambridge and his colleagues show that the biggest problem is not that models lack those microscopic details, but that the models assume the wrong boundary conditions [1]. Their result could have implications for the emerging field of soft robotics.
In experiments and simulations, the team investigated the failure dynamics of an upward-pointing cone compressed vertically between two plates. Such a system has been studied before, but whereas most previous models assumed that the cone’s base was fixed throughout the process, Duffy and his colleagues allowed it to move with respect to the bottom plate. Accordingly, they found that the base was the first part to deform, acquiring a trumpet-like flare. This flared region buckled under a lower stress than that required to initiate buckling in fixed-base models.
The new failure mode clearly represents a novel mechanism, Duffy says, because it follows a different scaling law: in earlier models, the critical load increases with the square of the cone’s thickness; in the new one it follows a 5/2 power law.
The finding could prove disappointing to some, as flat discs made of specially configured liquid-crystal elastomers form cones when heated, and these cones have been proposed as powerful actuators. But, as Duffy points out, understanding this weakness is the first step to resolving it.
–Marric Stephens
Marric Stephens is a Corresponding Editor for Physics Magazine based in Bristol, UK.
References
- D. Duffy et al., “Lifting, loading, and buckling in conical shells,” Phys. Rev. Lett. 131, 148202 (2023).