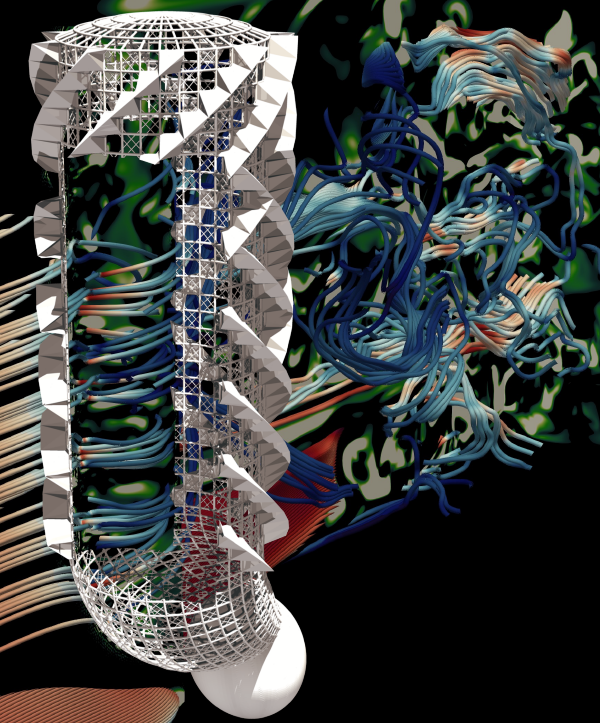
How Water Flows inside a Sea Sponge
Glass sponges make up a class of sea sponges that inhabit many ocean regions at depths of 450 to 900 meters. Anchored to the seafloor, these sponges draw sustenance from plankton and other organic debris. Because the food particles are sparsely distributed, the sponges need a way of filtering them from seawater. And that method should be efficient lest it consume too much precious energy. Now Giacomo Falcucci of the University of Rome and his collaborators have conducted fluid dynamics simulations of one species of glass sponge, the Venus flower basket (Euplectella aspergillum) [1]. They found that the sponge’s intricately shaped skeleton causes seawater to passively flow upward through the sponge’s filtration system, reducing the metabolic cost of extracting food.
Glass sponges owe their name to the star-shaped silica nanostructures, called spicules, that fuse together to form the skeletal structure. The body of a typical glass sponge resembles a flower vase with a central cavity, an opening at the top called the osculum, and lace-like walls that let seawater flow in and out.
Besides openings, the walls of the E. aspergillum sponge’s skeleton also feature spiral ridges. The geometry’s complexity dictated the simulation method. Rather than try to numerically solve the Navier-Stokes equation with a complex boundary, Falcucci turned to the lattice Boltzmann method. The method treats a fluid as a continuum of discrete particles and computes the probabilities of their successive locations on a lattice. The lattice can be complex, like the sponge’s skeleton, or simple, like undisturbed water, as needed. The simulation, which ran on a supercomputer, encompassed 1011 lattice points and 106 time steps.
The researchers explored different turbulence conditions, which can be characterized by the Reynolds number. Inside the sponge, the Reynolds number is given by uD/𝜈, where u is the undisturbed flow speed, D is the diameter of the osculum, and 𝜈 is the viscosity. In all, Falcucci and his collaborators considered 10 different values of the Reynolds number from 5 (not turbulent at all) up to 5000 (highly turbulent).
The simulations revealed that for all Reynolds numbers, a significant fraction of the water that flowed horizontally into the central cavity exited vertically via the osculum. The fraction was largest (37%) for a Reynolds number of 100, which corresponds to a relatively slow-moving horizontal flow of 1 liter per hour into the sponge cavity. Falcucci says that the high fractions at low Reynolds numbers hint at a functional adaption by the sponges to extreme living conditions where nutrients must be scavenged in nearly still water. What’s more, the flow that emerged from the osculum originally entered the cavity along its entire length, indicating the efficient use of ambient flow.
The notion that sea sponges rely on the passive conversion of horizonal flow to vertical flow was first proposed in 1972 [2]. That scenario, which also aimed to account for how prairie dogs ventilate their burrows, assumed that sponges use a velocity gradient that occurs in the boundary layer where seawater flows over the seafloor. The faster flow at the top of a sponge would draw up fluid from the slower flow at the sponge’s bottom, thanks to Bernoulli’s principle.
But it turns out that most of the body of E. aspergillum lies above the velocity gradient of the seafloor’s boundary layer. The simulations by Falcucci and colleagues show that an upward flow can still happen, as the sponge’s spiral ridges offer a kind of stairway that directs water upward. The operating principle resembles that of the helicopter that Leonardo da Vinci designed at the end of the 15th century. The helicopter would be kept aloft, the painter proposed, by a spiral-shaped aerial screw.
The sponges do not solely rely on passive flows to get their meals. The water moving up through the sponge’s body is filtered by a system that consists of nanoscale slits that extract food particles and of active pumps that help push fluid through. The importance of those pumps was studied in previous experiments on live sponges, both in the lab and in their natural habitat [3]. The researchers monitored the respiration of the sponges and found that the pumping comprised at least 28% of the organisms’ energy consumption. They also found that the vertical flow through the slits ceased when the pumps were disabled with electrical impulses. Those observations suggest that active pumping is vital for keeping sponges alive, but passive flow—as characterized by Falcucci and colleagues—helps sponges lower their energy bills.
–Charles Day
Charles Day is a Senior Editor for Physics Magazine.
References
- G. Falcucci et al., “Adapting to the abyss: Passive ventilation in the deep-sea glass sponge Euplectella aspergillum,” Phys. Rev. Lett. 132, 208402 (2024).
- S. Vogel and W. L. Bretz, “Interfacial organisms: Passive ventilation in the velocity gradients near surfaces,” Science 175 (1972).
- S. P. Leys et al., “The sponge pump: The role of current induced flow in the design of the sponge body plan,” PLoS ONE 6 (2011).