A new connection between electricity and magnetism

Robert  McMichael; Mark Stiles

Viewpoint

A new connection between electricity and magnetism

Center for Nanoscale Science and Technology, National Institute of Standards and Technology, Gaithersburg, MD 20899-6202

February 9, 2009• Physics 2, 11

A magnetic domain wall moving along a ferromagnetic wire can generate a voltage across the wire. This electromotive force, which is not the same as Faraday’s law of induction, is part of a growing family of interactions that are being discovered in the field of spintronics.

Figure caption — **Figure 1:** Micromagnetic model of a vortex-type domain wall being driven down a magnetic ( ${Ni}_{80} {Fe}_{20}$ ) wire by an applied magnetic field. The wire is $20 nm$ wide and $20 nm$ thick. (a) The color indicates the in-plane angle of the magnetization, and the arrows indicate the approximate magnetization direction. (b) The motion of the domain wall and the reversal of the core polarity at the wire edge in a constant applied field of $μ_{0} H_{appl} = 3 mT$ . Colors indicate the in-plane magnetization angle, and the altitude corresponds to the out-of-plane component of the magnetization. From left to right, the vortex is near the center of the stripe moving downward [ $0.0 ns$ , same as (a)], approaches the bottom edge $(2.5 ns)$ , annihilates $(3.5 ns)$ and reforms with negative polarity while moving away from the edge $(5.0 ns)$ . (c) Shows the trajectory of the vortex core as it traces out a zigzag path down the wire, changing polarity and direction at the edge. The red (blue) parts of the trajectory indicate that the core is pointing into (out of) the plane. The arrows indicate the direction of motion with time. The heavy black dots indicate the positions of the core in the images in (b). Both the vortex core polarity and the transverse vortex velocity, **$v_{y}$** , change sign at the wire edge. Therefore, the predicted domain-wall motion voltage does not change sign because it is proportional to the product of the two.

Electricity arises from the flow of electron charge, ferromagnetism from the ordering of electron spins. The interactions between electricity and ferromagnetism have been crucial to our technological development over the last centuries: the magnetic fields generated by electrical currents and the voltages generated by moving magnets are two cornerstones of our modern lifestyle. Important applications range from the largest power plants, to electric motors, down to the nanoscale write heads in our laptop hard drives. In contrast, the physics of ordered electron spin transport (a spin-polarized current) has become important only relatively recently. The new field of spintronics [1] is based on the ongoing prediction, discovery, and interpretation of additional interactions between electricity and magnetism that follow from the flow of spin-polarized electrons. Writing in Physical Review Letters, Shengyuan Yang and colleagues from the University of Texas, Austin, present a theoretical description and measurements of a new spintronic interaction: the voltage induced by a magnetic domain wall moving along a ferromagnetic wire [2].

So far, spintronic interactions have mainly been studied in devices made from layered ferromagnetic metal films, where the layers are thin enough that flowing electron spins carry magnetic information from layer to layer without drastic realignment. The most dramatic example is the finding that the electrical resistance between two magnetic layers depends on their relative magnetic orientation. The discovery of this effect, called giant magnetoresistance [3,4], led rapidly to the development of novel sensor and data storage technologies and was recognized with the 2007 Nobel Prize in Physics, awarded to Albert Fert and Peter Grünberg.

A related effect, dubbed “spin transfer torque,” occurs when spins flowing from one layer to another can reorient the magnetization in the layers [5]. The effects of spin transfer torque, such as precession and switching of magnetism in small junction devices, have been measured under a wide variety of conditions. The complementary effect, spin pumping [6], occurs when the dynamics of the magnetization in the layers drives a spin current in the device. The consequences of spin pumping are more difficult to observe than those of spin transfer torques but are still well established experimentally [7,8].

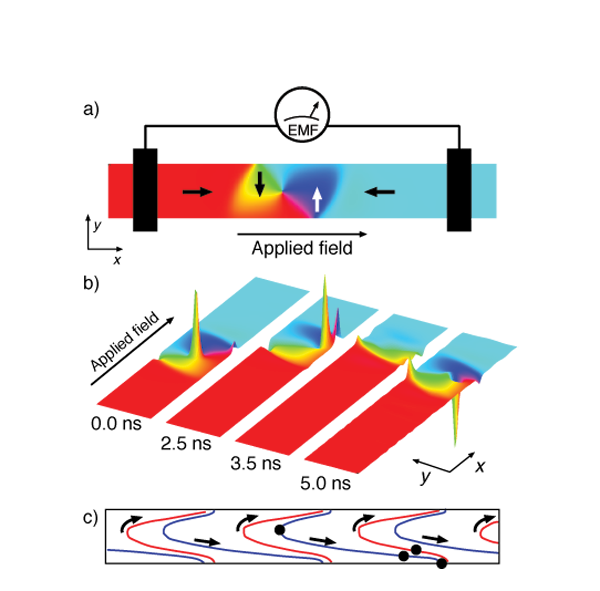
Spintronic effects are not only found in multilayer structures. For example, in single-layer magnetic nanowires, the analog to giant magnetoresistance is domain-wall resistance: the additional resistance due to a domain wall [Fig. 1(a)] between two regions of opposite magnetization. A current in a nanowire can also move a domain wall via the same spin transfer torques that causes precession and switching in multilayers [9–11]. As electrons flow through a ferromagnet, their spins tend to align with the magnetization. When they pass into a region of nonuniform magnetization, such as a domain wall, the electron spins rotate to stay aligned with the local magnetization direction. A reaction torque on the changing magnetization in the domain wall can cause the pattern of magnetization at the domain wall to move in the direction of the electron flow.

Several theoretical groups [12–15] have predicted the existence of a new effect that is both complementary to current-induced domain-wall motion and also analogous to spin pumping in layered structures: a voltage or current induced by domain-wall motion. The measurements of Yang et al. are the first observations of these effects [2].

Conceptually, it is straightforward to measure this domain-wall motion-induced voltage: simply propagate a domain wall down a ferromagnetic wire while measuring the voltage difference between the ends of the wire, as illustrated in Fig. 1(a). In practice, however, the voltage induced by the moving domain wall is only one of several voltages arising from different effects, and the challenge to the experimenter is to sort out this one small signal from other signals that compete with it. In particular, voltages induced by changing magnetic fluxes in the detection circuit, as described by Faraday’s Law, must be eliminated.

In the experiments devised by Yang et al., large alternating currents in a copper strip generate an alternating magnetic field that introduces domain walls and drives them down the wire. Each half-cycle of the driving field introduces one domain wall and drives it the length of the sample. In addition to creating and moving the domain walls responsible for generating the voltage of interest, the alternating magnetic field also introduces a competing induced voltage in the detection circuit, as well as other voltages induced by flux from the changing magnetization in nearby ferromagnetic structures. The authors separate the domain-wall signal from the competing signals by a clever modulation scheme. On top of the driving field, they superimpose a dc field and a modulation field at a much lower frequency than the driving field. They tune the amplitudes of the fields carefully so that over part of the period of the modulation field, the total field does not cross the threshold necessary for introducing domain walls. In this way, they achieve a low-frequency modulation of the signal due to domain-wall motion. On the other hand, the modulation field has a negligible effect on competing induced voltages, which allows them to distinguish the true signal. As additional checks on their results, the authors measure the dependence of the domain-wall motion-induced voltage on the domain-wall launching frequency and on the applied field. Both are consistent with the theory they present in the paper, offering additional evidence that their measured result is the new effect they describe.

The early predictions [12–15] for a voltage induced by domain-wall motion considered idealized domain walls, which were less complicated in structure than the domain walls in the wires of Yang et al. In the wires they study, domain walls take the form of a vortex, where the direction of the magnetization circulates around a vortex core [Fig. 1(a)]. At the vortex core, the magnetization tilts out of the plane and whether it tilts in a positive or negative direction determines the polarity of the domain wall. To understand Yang et al.’s measurements, it helps to look at a micromagnetic model of a constant and uniform magnetic field driving a vortex-type domain wall down a magnetic wire. In Fig. 1(a), the magnetization lies along the long direction of the wire except at the domain wall, where it changes direction. When a magnetic field is applied, the domain wall starts to move and the core approaches one of the edges. When the vortex core reaches the edge, its polarity reverses and it moves to the other edge. Figures 1(b) and 1(c) show the time evolution of a vortex core moving from left to right and changing polarity when it hits an edge. Since the early models are not directly applicable to vortex walls showing this complex motion, Yang et al. developed a new approach for computing the domain-wall motion-induced voltage that could account for the complexities of a realistic domain wall. Interestingly, they find the same magnitude of the voltage as predicted earlier for the motion of idealized domain walls subject to the same applied magnetic field.

As the first measurement of the voltage induced by domain-wall motion, the experiment described by Yang et al. is an important entry in the matrix of spintronic relationships between magnetic and electrical properties in magnetic metals.

References

I. Zutic, J. Fabian, and S. Das Sarma, Rev. Mod. Phys. 76, 323 (2004)
S. A. Yang, G. S. D. Beach, C. Knutson, D. Xiao, Q. Niu, M. Tsoi, and J. L. Erskine, Phys. Rev. Lett. 102, 067201 (2009)
M. N. Baibich, J. M. Broto, A. Fert, F. Nguyen Van Dau, F. Petroff, P. Etienne, G. Creuzet, A. Friederich, and J. Chazelas Phys. Rev. Lett. 61,2472 (1988)
G. Binasch, P. Grünberg, F. Saurenbach, and W. Zinn, Phys. Rev. B 39, 4828 (1989)
D. C. Ralph and M. D. Stiles, J. Magn. Magn. Mater. 320, 1190 (2008)
Y. Tserkovnyak, A. Brataas, G. E. W. Bauer, and B. I. Halperin, Rev. Mod. Phys. 77, 1375 (2005)
S. Mizukami, Y. Ando, and T. Miyazaki, Phys. Rev. B 66, 104413 (2002)
B. Heinrich, Y. Tserkovnyak, G. Woltersdorf, A. Brataas, R. Urban, and G. E. W. Bauer, Phys. Rev. Lett. 90, 187601 (2003)
L. Berger, J. Appl. Phys. 3, 2156 (1978); 3, 2137 (1979)
G. S. D. Beach, M. Tsoi, and J. L. Erskine, J. Magn. Magn. Mater. 320, 1272 (2008)
Y. Tserkovnyak, A. Brataas, and G. E. W. Bauer, J. Magn. Magn. Mater. 320, 1282 (2008)
L. Berger, Phys. Rev. B 33, 1572 (1986)
S. E. Barnes, J. Ieda, and S. Maekawa Appl. Phys. Lett. 89, 122507 (2006) ; S. E. Barnes and S. Maekawa, Phys. Rev. Lett. 98, 246601 (2007)
W. M. Saslow, Phys. Rev. B 76, 184434 (2007)
R. A. Duine, Phys. Rev. B 77, 014409 (2008)

About the Authors

Robert (Bob) McMichael is a physicist in the Electron Physics Group in the Center for Nanoscale Science and Technology (CNST) at the National Institute of Standards and Technology (NIST). Bob’s research at NIST has touched on a broad spectrum of phenomena in magnetic thin films and nanomaterials, but remains centered on micromagnetics and magnetization dynamics in magnetic thin films. He received a B.S. in Engineering-Physics from Pacific Lutheran University, and M.S. and Ph.D. degrees in Physics from The Ohio State University. He came to NIST in 1990 on an NRC Postdoctoral Research Fellowship, served on the research staff of the Metallurgy Division in the Materials Science and Engineering Lab, and then joined the CNST in 2007.

Mark Stiles is a physicist in the Electron Physics Group in the Center for Nanoscale Science and Technology at the National Institute of Standards and Technology (NIST). Mark’s research at NIST has focused on the development of ab initio theoretical methods for predicting the properties of magnetic nanostructures. He is a Fellow of the American Physical Society and he has served as a Divisional Associate Editor for Physical Review Letters. He received a M.S./B.S. in Physics from Yale University, and M.S. and Ph.D. degrees in Physics from Cornell University. Following postdoctoral research at AT&T Bell Laboratories, he joined the research staff at NIST. He is also an Adjunct Professor in the Department of Physics and Astronomy at the Johns Hopkins University.