Large rare patches of order in disordered boson systems

Peter B. Weichman

Viewpoint

Large rare patches of order in disordered boson systems

BAE Systems, Advanced Information Technologies, 6 New England Executive Park, Burlington, MA 01803, USA

September 28, 2009• Physics 2, 81

The existence, through statistical fluctuation, of arbitrarily large regions with a certain order in an otherwise disordered system, allow one to set bounds on various important thermodynamic properties.

Figure caption — **Figure 1:** (Top) Schematic illustration of lattice bosons near unit filling. At $n = 1$ in the Mott insulating phase (MI) when $(J / U) < (J / U)_{c}$ the effective wave function of each particle spreads only a finite distance $ξ (J)$ . For $n > 1$ ( $n < 1$ ), the extra particles (holes) travel freely within the essentially inert background, and the state is a superfluid (SF) for arbitrarily small $| n - 1 |$ . (Bottom) Phase diagram, showing Mott insulating (including those with higher integer filling) and SF phases. The transitions through the tips of the Mott lobes at $(J / U)_{c}$ (and following the dashed lines at constant filling beyond them) have a special particle-hole symmetry.

The effects of disorder on the phases and phase transitions in the zero-temperature ground state of many-body quantum systems have been of intense interest for many decades. Perhaps most familiar is the transition from a metal to an insulator in noninteracting electronic systems, where increasing disorder in the atomic lattice interferes with an electron’s ability to hop coherently from site to site, eventually confining each to a finite volume, a phenomenon known as Anderson localization.

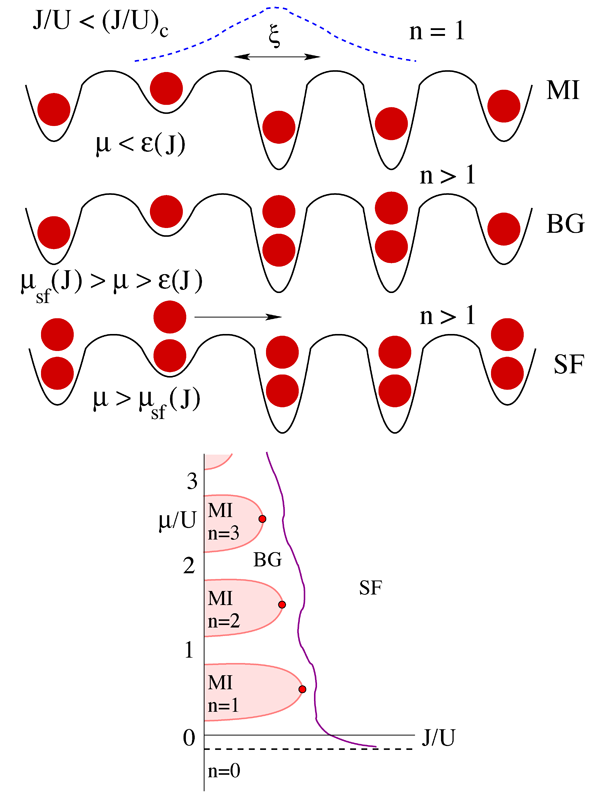
Analogous phenomena occur in Bose systems, such as $^{4} He$ absorbed in porous media, or magnetically trapped atomic vapors in periodic or disordered optical potentials, but there is now a fascinating interplay between disorder, interactions (required in the absence of Pauli exclusion to avoid system collapse), and superfluidity [1]. The clean system is visualized in the top panel of Fig. 1, where one imagines populating a periodic potential with one boson per site (filling factor $n = 1$ ). If the tunneling amplitude $J$ between sites is weak then, even in the absence of disorder, the onsite mutual hard-core repulsion $U$ localizes the effective single-particle wave functions to a finite size $ξ (J)$ . The resulting phase is known as a Mott insulator, and is identified by the finite energy gap $ε (J)$ required to overcome the repulsion and add a particle (or hole) to the system. This phase is therefore also incompressible.

This system can now be driven into a superfluid phase in two distinct ways (bottom panel of Fig. 1). First, one may increase (or decrease) the chemical potential $μ$ , overcome the energy gap, and add a small density of particles (or holes). These particles are free to propagate coherently throughout the system, and effectively form a dilute Bose superfluid on top of a uniform insulating background. Alternatively, one may increase the hopping strength $J$ (or lower the repulsion $U$ ) so that the ratio $J / U$ exceeds a critical value $(J / U)_{c}$ . The length $ξ (J)$ diverges, and particles and holes simultaneously gain sufficient mobility, tunneling coherently through each other to form a dense, strongly interacting superfluid. This phase transition is characterized by the existence of an extra particle-hole symmetry, and belongs to a different universality class [1] compared to the first scenario.

Consider now the addition of disorder, visualized in Fig. 2. If it is sufficiently bounded, a shrunken incompressible Mott phase with fixed filling $n = 1$ still survives. A sufficiently large chemical potential can again be applied to overcome the energy gap and add extra particles (or holes) to the system. A key question now is “do these particles still form a superfluid?” At least for small hopping $J$ , the answer must be no; the extra particles still see a residual random potential due to the distortion of the background Mott phase by the disorder. The usual Anderson localization arguments then imply that the effective single-particle energy states experienced by these particles must be localized, and the system remains insulating. However, the density can be varied continuously, so this phase, known as a Bose glass, is compressible.

As one continues to add particles or holes to the system, the background becomes gradually more smooth, the localization length increases, and the system eventually undergoes a superfluid transition (bottom panel of Fig. 2). A picture to keep in mind is that of isolated superfluid droplets that grow, join, and percolate to eventually span the system.

The question remaining, which has generated much controversy in recent years, is whether the Bose glass phase must completely surround the Mott lobe, or whether, in fact, a direct Mott-superfluid transition might take place at larger $J$ , closer to the tip, or perhaps only through the tip. Strong arguments, based on this superfluid droplet picture, have been presented [1,2] that forbid such a direct transition, but a rigorous proof remained elusive. In a recent paper appearing in Physical Review Letters [3], Lode Pollet, Nikolai Prokof’ev, and Boris Svistunov at the University of Massachusetts, US, and Matthias Troyer from ETH Zurich, Switzerland, have presented precisely such a proof. The previous arguments were based on proposals for the Mott phase boundary in terms of that of the periodic system, and the phase just beyond it. Unfortunately, this boundary is highly model dependent, and depends on reasonable, but unproven, assumptions about the influence of nonperturbative, finite amplitude disorder. The key insight in the rigorous proof is to focus instead on the superfluid phase boundary, and the nature of the phase just beyond it [3] (see, however, Ref. [4] where the implications of the proof for the Mott-Bose glass transition are addressed as well).

Pollet and his colleagues use so-called “large rare region” arguments that are based on the simple but powerful idea that a finite set of random variables will, with finite probability, take values within any specified range, no matter how restricted. Moreover, in an infinite, translation invariant system, any such finite probability event will take place infinitely often somewhere in the system. For example, independently assigned random site potentials will, through statistical fluctuations, give rise to arbitrarily large, arbitrarily near-uniform regions that mimic the properties of the bulk periodic system. Within the Bose glass phase, for example, there must exist arbitrarily large superfluid droplets. Though isolated, the known properties of these droplets allow one, for example, to prove that the superfluid excitation spectrum is gapless.

Taking this a step further, one may focus on large regions over which one probability distribution mimics another. Thus, for example, an infinite sequence of flips of a fair coin, will have arbitrarily long subsequences that appear to be those of an unfair coin, with, say, 2:1 (or any other) ratio of heads to tails. Applying this idea to the insulating phase near the superfluid transition line, it follows that there always exist large rare regions in which the disorder distribution mimics that of a system that lies on the superfluid side of its transition line. Thus the insulating phase must contain arbitrarily large superfluid droplets. Since the superfluid phase is compressible, this can be used to show that this phase not only has no energy gap, but is in fact compressible, i.e., it must be a Bose glass not a Mott phase [3,4].

Such large rare regions are the bane of Monte Carlo simulations, which necessarily are limited to (relatively small) finite domains. This easily explains how very rare compressible regions could be missed in what otherwise numerically looks like a Mott phase. The weak disorder limit in some studies also leads to situations where the superfluid compressibility is irresolvably small for entirely different reasons, again mimicking a Mott phase.

Field-theoretic approaches contain all “rare region effects” because disorder is fully averaged from the beginning, but choosing the form of the action that describes the correct universal “fixed point” structure of the model is a subtle issue [2]. One may consider disorder that preserves particle-hole symmetry on average, and leads to no change in average filling; or disorder that breaks particle-hole symmetry outright and leads to a net change in filling. The latter generates terms in the action that appear to provide a much stronger perturbation to the periodic model than those generated by the former. However, the rare regions arguments of Pollet et al. prove this to be illusory. The operative notion is “spontaneous restoration of symmetry”, and occurs in many systems [5,6]—the most familiar being the restoration of up-down Ising symmetry at liquid-vapor critical points. Thus it turns out that the former choice describes the correct fixed point model, and the terms breaking global particle-hole symmetry actually disappear at the superfluid transition. A fundamental error often made, then, is to not bother including the weaker terms under the assumption that they are not important, thereby obtaining an incorrect description of both the insulating phase and the superfluid phase transition. The key observation is that apparently weak terms that break a fundamental symmetry of the problem can have an outsized effect, and this is indeed the case here.

References

M. P. A. Fisher, P. B. Weichman, G. Grinstein, and D. S. Fisher, Phys. Rev. B 40, 546 (1989)
P. B. Weichman and R. Mukhopadhyay, Phys. Rev. B 77, 214516 (2008)
L. Pollet, N. V. Prokof’ev, B. V. Svistunov, and M. Troyer, Phys. Rev. Lett. 103, 140402 (2009)
V. Gurarie, L. Pollet, N. V. Prokof’ev, B. V. Svistunov, and M. Troyer arXiv:0909.4593v1
M. P. A. Fisher, Physica (Amsterdam) 177A, 553 (1991)
A. T. Dorsey and M. P. A. Fisher, Phys. Rev. Lett. 68, 694 (1992)

About the Author

Peter B. Weichman received his Ph.D. from Cornell University in 1986. He is currently a senior research scientist at BAE Systems in Burlington, Massachusetts. His interests span a wide range of topics in theoretical physics, including quantum and classical phase transitions, fluid turbulence, geophysical fluid flow, oceanography, and electromagnetic and acoustic remote sensing. He has been especially interested in all forms of boson mistreatment for more than 25 years.