Led by the nodes
In any new class of superconducting materials, the momentum space structure of the wave function describing bound “Cooper pairs” of electrons of momentum k and -k is considered one of the central clues to the origins of the pairing interaction that binds the electrons together. One key feature of such a wave function, as for atomic wave functions, is the number of times it changes sign; knowledge of the number and position of these nodes can give information on the underlying symmetries as well as the momentum dependence of the pairing interaction. Within the BCS theory of superconductivity, the energy gap Δ(k) (or superconducting order parameter) is directly related to this wave function, and more easily measured. For the new iron-based superconductors, three years of intense research have not yet produced a consensus on the symmetry or nodal structure of this energy gap in k space [1]. Nevertheless, as sample quality has improved considerably, to the point where similar probes are finding similar results on similar samples, there is a growing belief that the superconducting gap function in these systems may be of one symmetry class (called A1g), but intrinsically diverse as regards the nodal or near-nodal structure of the gap. Calculations suggest that this may be due to the sensitivity of the gap nodes in this class to small perturbations of the electronic structure [2,3].
In a Physical Review B article [4], Jean-Philippe Reid and collaborators from the University of Sherbrooke, Canada, Ames Laboratory and Iowa State University, both in the US, and the Canadian Institute for Advanced Research, use low-temperature heat transport measurements to probe the nodal and near-nodal structure of the superconducting gap in Ba(Fe1-xCox)2As2. An observation of a finite residual linear term in the thermal conductivity κ(T) as T→0 gives direct evidence of gap nodes [5]. If the nodes are only slightly lifted, leaving a small gap, the application of a moderate magnetic field leads to field-induced thermal transport. In this way, by measuring κ(T), with and without a magnetic field, for different directions of the heat current along the principal axes of the crystal, one can search for the nodal or near-nodal regions of the Fermi surface. In Reid et al.’s article, results of heat transport measurements in Ba(Fe1-xCox)2As2 for a range of dopings are reported. In the absence of a magnetic field, a linear term in the low-temperature c-axis thermal conductivity is observed, implying that there are nodes in the gap in regions of the Fermi surface that dominate the c-axis thermal conductivity. In zero field, the linear term is absent or unobservably small for the a-axis thermal conductivity [6]. However, in the presence of a moderate magnetic field, excited quasiparticle heat conduction along the in-plane a-axis is seen. This implies that there are near-nodal gap regions associated with deep minima of the gap in regions of the Fermi surface that dominate the in-plane thermal transport.
Almost all of the analyses of symmetry classes and nodal structures for the iron-based systems have been performed assuming a two-dimensional (2D) Fermi surface for these materials, which consists of small, nearly circular pockets of electrons and holes. This simplification was justified in terms of electronic structure calculations and transport measurements on the original family of iron-based superconductors, the so-called 1111 family. However, measurements on the second such family discovered, the so-called 122 materials, suggest that they are considerably more three-dimensional (3D) [7,8]. This feature of the 122 systems is potentially important for applications of high-temperature superconductivity, since two-dimensionality promotes fluctuations and anisotropic critical fields that can degrade the currents superconducting wires can carry. Reid et al. reported a linear T term in the low-temperature c-axis heat conductivity of Ba(Fe1-xCox)2As2 crystals, reflecting the existence of order parameter nodes, compared to a small or zero linear term in the ab plane. Furthermore, the anisotropy ratio (κc/κc,N)/(κa/κa,N) of the electronic thermal conductivities normalized to the normal state value was found to increase as the sample was doped away from optimal Tc. Reid et al. argue that such an anisotropy can arise if the gap nodes are located near flared regions of the quasicylindrical Fermi surfaces, such that the corresponding low- T κa is negligible. Nodes contributing specifically to c-axis transport had been suggested in Ref. [8].
Another striking feature of these experiments is that the T→0 asymptotic values of κa/T are much smaller than one might expect from the simple BCS theory of quasiparticle transport in a 2D nodal superconductor. Starting from known results for a d-wave superconductor, using appropriate parameters for the iron-based systems, and scaling to 3D, one obtains a much too large κa/T compared to experiment. The low- T linear term in the thermal conductivity is predicted by theory to be universal (disorder-independent for small disorder) in the p-wave or d-wave case [9,10]. Mishra et al. [11] showed recently that this expression continues to hold in the A1g type states thought to be characteristic of iron-pnictide systems, but that universality breaks down quickly due to the strong influence of disorder. This effect usually makes the residual thermal conductivity bigger, not smaller, however.
The leading class of theories for the pairing in these materials relies on an effective interaction between electrons of momentum/spin k↑, -k↓, which arises from the exchange of spin fluctuations. Early 2D calculations of this type [2] for the pairing state of the iron-based superconductors found that nodes could occur on the electron sheets, but the gap on the hole sheets was always fairly large and isotropic. Vertical nodes running the length of the electron Fermi cylinders, as found in these approaches, would produce a large linear term in the thermal conductivity, in contradiction to experiment. Therefore, a true gap must exist on the electron sheets. On the other hand, the minimum gap on this surface can be quite small; indeed it must be to account for the observed magnetic field dependence [11]. But at the lowest temperatures (the thermal conductivity measurements reflect the asymptotic behavior of κ/T in the 50mK range), one may assume that one is well below this gap energy. Therefore, within this 2D framework it is difficult to understand how the linear T term in κ observed by Reid et al. can be generated.
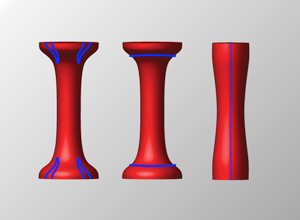
Recently, however, Ref. [12] presented a calculation of 3D spin-fluctuation pairing in the 122 systems. The ground state was found to be generically of A1g symmetry, as in the 2D case; on the other hand, the gap on the hole sheets was now found to have short nodal segments near the top of the Brillouin zone at momenta where the Fermi surface sheets display some outward flaring, like the bell of a French horn (Fig. 1, left and center). The nodal lines on the hole sheets in such states can be nearly vertical, as shown in Fig. 1, left, or nearly horizontal, as sketched schematically in Fig. 1, center. For such parameters, the electron sheets were found not to possess nodes, but rather deep minima of the superconducting gap corresponding to the vertical lines spanning the whole Brillouin zone in the z direction, as shown in Fig. 1, right. While electronic structure calculations [13] disagree on whether such highly flared hole sheets are present, they have been observed, for some dopings, in angle-resolved photoemission experiments on 122 materials [14].
These microscopic predictions accord rather well with the qualitative explanation of Reid et al. for their results. They point out that the heat current is weighted by the Fermi velocity squared, and that if flared portions of the Fermi surface coincide with the positions of the nodes, a significant κc/T might be paired with a tiny and perhaps unobservable κa/T. In the two schematic examples in Fig. 1, left and center, nodes indeed occur in these locations. In addition, it is qualitatively clear from the figure why the size of the observed linear term in the plane might be small compared to the value estimated from the d-wave case. The full result can be obtained only in an effective 2D situation in which the nodes run the entire length of the Fermi cylinder; to the extent the nodal surfaces consist of short segments as in these states, the phase space will be smaller and one will obtain a correspondingly small value of κ/T. It remains to be seen whether a fully consistent picture of the zero-field T dependence can be made to accord with the much more isotropic field dependence observed.
An additional bulk probe of the superconducting gap Δ(k) is provided by specific heat measured as a function of magnetic field direction [15–18]. Such a measurement of C(T;H) as a function of the field angle α which the field H makes with the a axis in the ab plane, as has already been performed in the FeTe system [19], would clearly reveal the difference between the cases shown in Fig. 1, left and center; if one could also rotate the field H by the polar angle θ relative to the crystalline c axis, one could, in principle, map out the kz positions of the nodes as well.
The determination of the nodal and near-nodal structure of the superconducting gap Δ(k) over the multiple Fermi surfaces of the iron-based superconductors can provide a fingerprint of the momentum dependence of the pairing interaction. Just as the frequency dependence of the gap for the traditional low- Tc superconductors (such as Pb and Hg) was used to identify the pairing interaction in these materials as arising from a “retarded” phonon-mediated and an “instantaneous” screened Coulomb interaction, the momentum dependence of the gap in the iron-based superconductors can test the various pairing mechanisms that have been proposed for these materials. The thermal conductivity measurements of Reid et al. have provided an important step towards this goal.
References
- K. Ishida, Y. Nakai, and H. Hosono, J. Phys. Soc. Jpn. 78, 062001 (2009); J. Paglione and R. L. Greene, arXiv:1006.4618; D .C. Johnston, arXiv:1005.4392
- K. Kuroki et al., Phys. Rev. Lett. 101, 087004 (2008); F. Wang, H. Zhai, Y. Ran, A. Vishwanath, and D.-H. Lee, 102, 047005 (2009); S. Graser, T. A. Maier, P. J. Hirschfeld, and D. J. Scalapino, New J. Phys. 11, 025016 (2009)
- A. Kemper et al., New J. Phys. 12, 073030 (2010)
- J-P. Reid, M. A. Tanatar, X. G. Luo, H. Shakeripour, N. Doiron-Leyraud, N. Ni, S. L. Bud’ko , P. C. Canfield, R. Prozorov, and L. Taillefer, Phys. Rev. B 82, 064501 (2010)
- H. Shakeripour, C. Petrovic, and Louis Taillefer, New J. Phys. 11, 055065 (2009)
- X. G. Luo et al., Phys. Rev. B 80, 140503 (2009); L. Ding et al., New J. Phys. 11, 093018 (2009); M. A. Tanatar et al., Phys. Rev. Lett. 104, 067002 (2010)
- M. A. Tanatar et al., Phys. Rev. B 79, 094507 (2009)
- C. Martin et al., Phys. Rev. B 81, 060505 (2010)
- M. Graf, S.-K.Yip, J. A. Sauls, and D. Rainer, Phys. Rev. B 53, 15147 (1996)
- M. R. Norman and P. J. Hirschfeld, Phys. Rev. B 53, 5706 (1996)
- V. Mishra et al., Phys. Rev. B 79, 094512 (2009)
- S. Graser et al., Phys. Rev. B 81, 214503 (2010)
- D. J. Singh, Phys. Rev. B 78, 094511 (2008); A. S. Sefat et al., Phys. Rev. Lett. 101, 117004 (2008); G. Wang, Y. Qian, G. Xu, X. Dai, and Z. Fang, 104, 047002 (2010)
- S. Thirupathaiah et al., Phys. Rev. B 81, 104512 (2010); V. Brouet et al., 80, 165115 (2009)
- For a review, see Y. Matsuda, K. Izawa, and I. Vekhter, J. Phys. Condens. Matter 18, R705 (2006)
- S. Graser et al., Phys. Rev. B 77, 180514 (2008)
- A. B. Vorontsov and I. Vekhter, arXiv:1006.0738
- A. V. Chubukov and I. Eremin, arXiv:1006.3091
- B. Zeng et al.,arXiv:1007.3597