Tiny resonators generate a large optical spectrum
If the invention of the laser appeared to be a solution in search of a problem, the optical frequency comb is a set of solutions for an entirely new realm of problems. In its simplest implementation, an optical frequency comb can be visualized in the frequency domain as an array of equally spaced and coherently related well-defined frequencies (or modes) spanning a broad spectral range. As a versatile ruler to calibrate the frequency of light with tens of thousands of equally spaced tick marks, an optical frequency comb enables exquisitely precise measurements of the electromagnetic spectrum. This Nobel-prize-winning technology [1] has driven to the heart of fundamental physics through experiments probing the temporal stability of fundamental constants [2], yet adeptly addressing practical concerns such as molecular fingerprinting and ultrasensitive trace gas detection [3]. Further applications include boosting the sensitivity, range, resolution, and allowing non-colocated LIDAR (light detection and ranging) [4], vastly increasing the number of signals traveling through optical fiber, and entangling atomic quantum bits [5]. For a comb with sufficiently broad bandwidth, it is now straightforward to stabilize and to determine the absolute frequencies of all comb lines [6], revolutionizing optical-frequency metrology, wave-form synthesis, and optical atomic clocks. Although much of the research has been driven by “conventional” optical frequency combs derived from femtosecond modelocked lasers, the push for low power, compact devices has sparked interest in many novel techniques, each successively smaller, pushing into the realm of microtechnology. These include miniature mode-locked lasers [7], gas-filled fibers [8], compact fiber cavities [9], and microresonators [10].
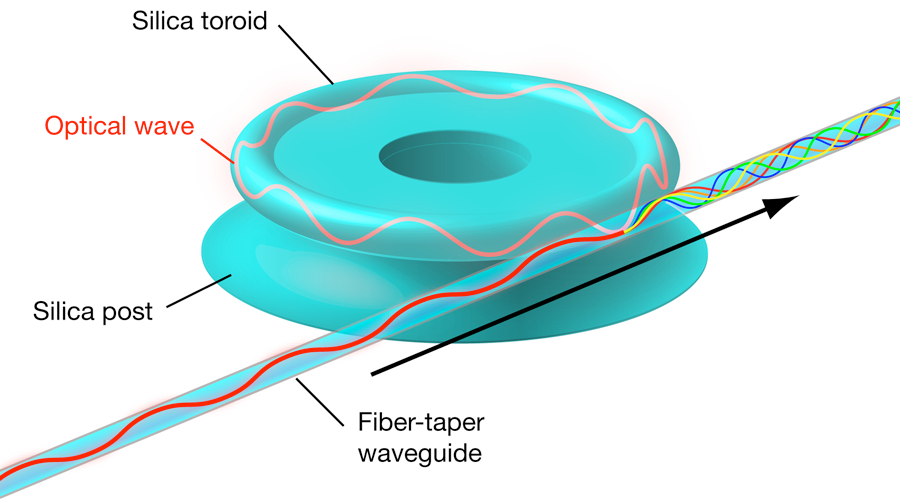
Writing in Physical Review A, Yanne Chembo and Nan Yu of the NASA Jet Propulsion Lab in Pasadena, US, now provide the first complete analysis of one implementation of these compact frequency combs belonging to a class of devices called whispering-gallery-mode (WGM) resonators [11]. Whispering galleries such as those at St. Paul’s Cathedral in London, Statuary Hall in Washington DC, and St. John Lateran’s Basilica in Rome are well known to reflect sound produced at one focus of an elliptical chamber, along the wall to the diametrically opposite focus. Sound bounces from the wall of the gallery with very little loss of energy and thus can be heard at a great distance. The same phenomenon occurs naturally for radio waves propagating in the atmosphere. This acoustical whispering-gallery mode, first described by Rayleigh [12], has an optical analog in microspheres and microtoroids that has attracted the attention of many researchers as a result of its the tight spatial confinement and low dissipation. In a miniature, optical version of a life-sized whispering gallery, laser light is coupled into a circular waveguide, such as a microtoroid, which can have a diameter close to that of a human hair (Fig. 1). When the light propagates along the boundary of the ring at a grazing angle, its mode is guided along the circumference. The wave packet can make numerous trips around the ring before it is absorbed, but only frequencies of light that fit perfectly within the circumference of the ring can do so. If the light path is an integral number of wavelengths, the light waves constructively superimpose each trip around. This match between the propagating frequency and the circumference is called a resonance, or a whispering-gallery mode.
In a linear world, the WGM resonator would merely store the single input laser frequency until the energy has dissipated. The combination of the high quality factor and tight mode confinement, however, enable efficient nonlinear interactions. Typically avoided in fiber optics, here the nonlinear interactions are fostered. The single input pump frequency drives the generation of an entire assemblage of well-defined frequencies through cascaded nonlinear interactions, creating a comb of frequencies.
First, through degenerate four-wave mixing, the pump laser obtains two frequency sidebands, often termed signal and idler frequencies. Due to the cavitylike nature of the WGM, where resonance occurs at an integral number of wavelengths, the signal and idler are born at an integral number of modes of the cavity. Through a complex set of interactions, the signal and idler frequencies then successively mix with the pump laser to create a series of cascaded nonlinearities or “hyperparametric” interactions. Since the microcavity exhibits dispersion due to the frequency-dependent index of refraction of the glass, the mode spacing of the WGM is nonuniform. This phenomenon, combined with the nonlinear mixing, which forces only pairwise equidistant modes, suggests generation of a useless and irregular comblike structure of frequencies—a ruler with unknown and varying spacing. Nonetheless, high-precision measurements against a conventional frequency comb reveal that the sidebands’ deviation from the equidistant position is smaller than a few milihertz across an octave of spectrum [13], revealing a gap between theoretical understanding and experimental results.
Until now, despite significant experimental effort in frequency comb generation with WGM resonators, the nature of the nonlinear interaction only has been qualitatively postulated. Chembo and Yu show the first quantitative analysis of the massively cascaded hyperparametric interactions. The authors establish a framework for the study of combs generated in monolithic resonators, addressing a series of unanswered questions about the fundamental properties of combs generated within whispering-gallery-mode resonators. Their general set of equations provides insight into vital concerns, such as regions of comb stability, pump thresholds, and the role of material dispersion. They show evidence of why the modes are indeed equally spaced, a critical feature in the versatility and usefulness of the comb, and derive counterintuitive results that limit the breadth or bandwidth into which the comb can be generated. For ease of frequency stabilization, an octave of spectral bandwidth (where the high-frequency components are twice that of the low frequency) is desired. Although intuition would suggest the widest comb generation at the zero dispersion condition or where geometrical and material dispersions cancel, the article shows an instability region where comb generation is prohibited. Additionally, crucial questions, such as the frequency position of the primary signal and idler, are reproduced by their theory, and strong coupling of adjacent modes as compared to that of the extrema is shown. Their analysis can be extended with a full noise analysis investigating the optical phase diffusion of each mode and phase noise of microwaves extracted from this type of comb.
Although nonuniform dispersion of the output coupling of WGM resonators still presents challenges for short pulse applications of these lasers, the tunable broad frequency comb with known spectrum enables numerous areas of physics, from entangled qubits to bridging the optical-microwave gap, which could unify metrology with micro- and nano-photonics. The intriguing ability of microresonator combs to derive an optical frequency comb directly from a continuous-wave-laser source shows great experimental promise. A deeper understanding of the theoretical nature of these low-power, whispering-gallery-mode resonators focuses experiments on methods to further advance the achievable bandwidth, stability, noise properties, and applications of these infinitesimal combs.
Acknowledgments
The work at Lincoln Laboratory is supported by the Department of the Air Force, under Contact Number FA8721-05-C-0002. Opinions, interpretations, conclusions, and recommendations are those of the author and are not necessarily endorsed by the United States Government.
References
- See the Nobel lectures by John L. Hall, Rev. Mod. Phys. 78, 1279 (2006); Theodor W. Hänsch, Rev. Mod. Phys. 78, 1297 (2006)
- T. Rosenband et al., Science 319, 1808 (2008)
- S. A. Diddams, L. Hollberg, and V. Mbele, Nature 445, 627 (2007); B. Bernhardt et al., Nature Photon. 4, 55 (2010)
- I. Coddington, W. C. Swann, L. Nenadovic, and N. R. Newbury, Nature Photon. 3, 351 (2009)
- D. Hayes et al., Phys. Rev. Lett. 104, 140501 (2010)
- H. R. Telle, G. Steinmeyer, A. E. Dunlop, J. Stenger, D. H. Sutter, and U. Keller, Appl. Phys. B 69, 327 (1999)
- A. Bartels, D. Heinecke, and S. A. Diddams, Science 326, 681 (2009)
- F. Couny and F. Benabid, J. Opt. A 11, 103002 (2009)
- D. Braje, L. Hollberg, and S. A. Diddams, Phys. Rev. Lett. 102, 193902 (2009)
- P. Del’Haye et al., Nature 450, 1214 (2007); A. A. Savchenkov et al., Phys. Rev. Lett. 101 093902 (2008); I. S. Grudinin, N. Yu, and L. Maleki, Optics Lett. 34, 878 (2009); J. S. Levy et al., Nature Photon. 4, 37 (2009); L. Razzari et al., 4, 41 (2009)
- Y. K. Chembo and N. Yu, Phys. Rev. A 82, 033801 (2010)
- L. Rayleigh, Philos. Mag. 20, 1001 (1910)
- P. Del’ Haye, O. Arcizet, A. Schliesser, R. Holzwarth, and T. J. Kippenberg, Phys. Rev. Lett. 101, 053903 (2008)