How Solid is Supersolid?
Among known states of natural matter, supersolids are often regarded as counterintuitive in the extreme. For more than 50 years, a number of authors with different perspectives such as Gross [1], Andreev and Lifshitz [2], Thouless [3], and Chester [4], have contemplated this exotic state. Thought to be the coexistence of superfluid and crystalline orders in the same single-component material, supersolids still attract broad interest. The original predictions were made for perfect quantum crystals in free space, with the most promising candidate being solid helium- 4 ( 4He), which is known to have the largest amplitude of zero-point motion of atoms in the ground state. Such motion was presumed to create a stable (repulsive) gas of zero-point vacancies undergoing Bose-Einstein condensation at low temperature. Thus the perfect crystal would simultaneously be a solid and a superfluid. This simple and attractive idea sparked a wide experimental search for supersolidity in solid 4He, which, however, yielded no positive results. Recent interest in the supersolid state of matter was reignited after observations of a signal consistent with supersolidity in the torsional oscillator experiment performed by Kim and Chan at Penn State [5] in 2004. In what follows, we will survey both the recent experimental findings and the theoretical landscape in supersolid research.
In terms of theory and calculations, the problem of supersolidity in free space for solids with one or two atoms in the unit cell is not amenable to any existing analytical scheme. This is the result of strong competition between the two orders—superfluid and crystalline—at the interatomic distance. In fact, the crystalline lattice is free to adjust its period continuously in order to eliminate vacancies and/or interstitials introduced out of equilibrium. As of today, there is no clear understanding of what is required to obtain a stable supersolid state in free space with few atoms in the unit cell, and this is one interesting research direction to explore.
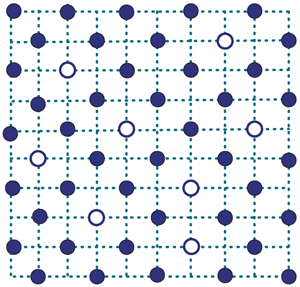
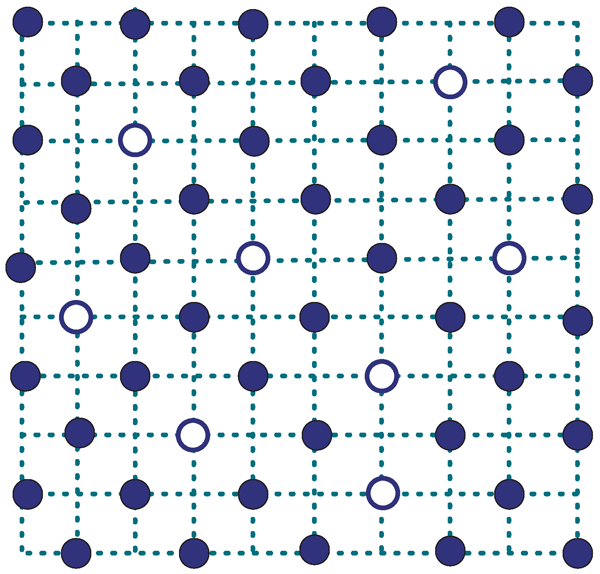
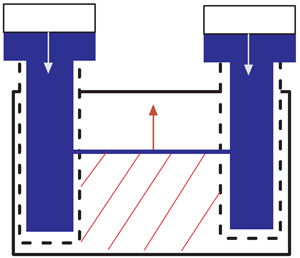
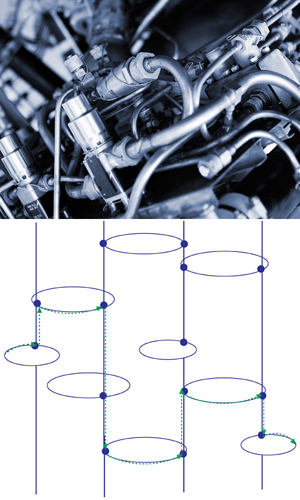
Widespread efforts are also aimed at finding simplified models of supersolids. Lattice supersolids are quite well studied nowadays [6]; in such systems the lattice is imposed externally, and accordingly, the solid period is forced to take a discrete set of values, i.e., it cannot change continuously. Crystalline order in such lattice models breaks the discrete translation invariance of the external lattice potential and is typically “pinned” to the lattice, as shown in Fig. 1. As a result, excitations of such order cost finite energy—as opposed to a solid in free space. The superfluid component can be introduced by doping the solid, i.e., by adding (removing) atoms to produce particle (hole) excitations that, if stable against phase separation—which is always an option—Bose condense. In many respects, this picture is similar to that of the so-called doped Mott insulator. In such an insulator, the number of particles is exactly equal to the number of the lattice sites. Should the onsite repulsion between particles significantly exceed the kinetic energy loss due to localization, particles become localized—one at each site. If, however, the balance between particles and sites is violated, the insulator becomes a superfluid. Most importantly, pinned solid structures lack the ability to adjust their period continuously to make it commensurate with the particle number and eliminate the superfluid component (shown by open circles in Fig. 1). It seems that most known lattice supersolids are of this kind, with a notable exception presented recently in Ref. [7].
At the other end of the problem we find Bose-Einstein condensates with the spatial modulation of density induced by interparticle interactions, as first envisioned by Gross [1] (see also Ref. [8]). These supersolids are best described within the classical field approach, relying on the mean-field description of bosons in terms of the so-called Gross-Pitaevskii equation, where the quantum bosonic operator is replaced by a classical field. They emerge in the special limit of weak interatomic potentials and a large (infinite in the classical-field limit) number of particles in the unit cell. A nontrivial situation occurs when the spatial period becomes comparable with the interparticle distance. In this regime, the discreteness of particle number becomes important and the Gross-Pitaevskii approach fails when this number approaches unity.
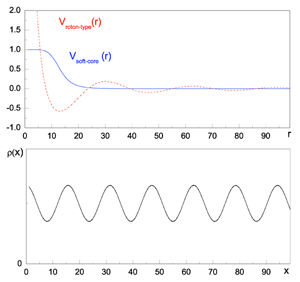
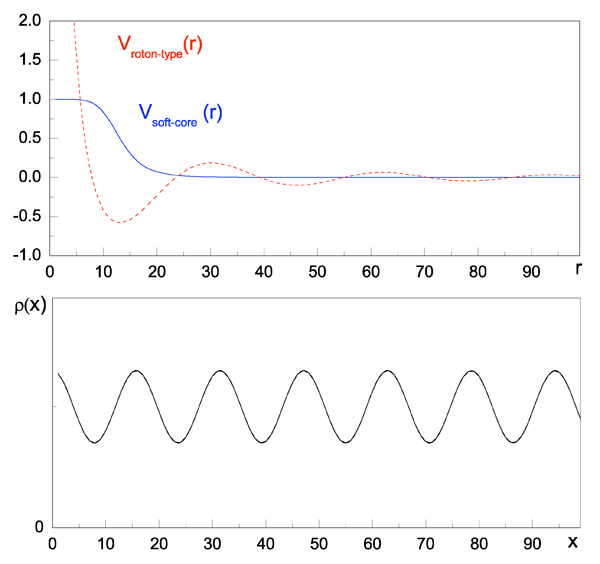
One may also think of tailoring a two-body potential to extend the region of stability for the supersolid phase in free space, starting from the classical field perspective. This idea was realized by Cinti et al. and Saccani et al. [9], when a microscopic model featuring the supersolid phase (with the number of particles per self-organized unit cell being around 10) was constructed. The potential consists of a generic repulsive part, saturating to a constant below some radius R0. The microscopic structure of the supersolid state is that of liquid droplets (each of a radius ∼R0) arranged in a regular solidlike structure. Since particles in each droplet are in a liquid state, the overall phase coherence is achieved by means of Josephson coupling between the droplets. Since the interatomic distance is much smaller than a typical wavelength of the density modulations, the discreetness of the particle number in the unit cell becomes irrelevant and the resulting supersolid can be described within the classical field approach, that is, following from the solutions of the mean-field equations. These key features are depicted in Fig. 2, which shows the shapes of the interaction potentials used in Refs. [8,9], as well as the resulting density modulation—the crystalline-type order. It would be interesting to establish for some reference cases where the boundary between the classical and quantum worlds stands in terms of the number of particles per unit cell. At the moment it seems very unlikely, though not improbable, that a supersolid with one atom per unit cell exists for a two-body interaction potential of a standard shape [10]. Similar classical field considerations apply to lattice models in the limit of large occupation numbers and weak interactions (on-site and nearest-neighbor), ensuring large supersolid regions in the parameter space [11]. Remarkably, the supersolid behavior in such lattice models survives even at filling factors close to 1/2 [7].
Although not yet observed experimentally or numerically, the supersolid state is guaranteed to exist in two-dimensional dipolar systems. This is an immediate consequence of the theorem that first-order transitions that involve a density change, such as liquid-solid ones, are forbidden due to log-divergent negative terms in the surface tension (see, e.g., Ref. [12]). It is thus guaranteed that on approach to the solid phase from the superfluid side of the phase diagram (by changing any control parameter) one can construct a state containing large solid domains that is lower in energy than the homogeneous liquid. At T=0, such domains will form a secondary lattice superstructure leading to the global supersolid phase (in fact, a whole set of supersolid phases [12]). The microscopic picture of this phenomenon, however, was never quantified by measuring the surface tension parameters.
The experimental situation in solid 4He
Apart from general fundamental questions, the main puzzle is to understand what happens in 4He, where both orders are in strong competition with each other. Initial attempts to detect supersolid properties [13] have yielded essentially nothing, including the new results in solids subject to pressure gradients [14]. However, following Leggett’s torsion oscillator (TO) proposal [15] and hints of anomalous behavior found by Goodkind in ultrasound measurements of hexagonal-close-packed (hcp) 4He at temperatures T≈0.2K [16], in 2004 Kim and Chan discovered a change in the TO period. They interpreted it as the signature of the nonclassical rotational inertia (NCRI) of 4He atoms in the solid state [5]. Since then, small TO period reduction by a few nanoseconds (for a typical resonant period of ∼1 millisecond) below T≈0.2K has been reproduced by many groups all around the world. Specific heat measurements also show an anomaly (a wide and weak peak) in the same temperature range [17]. It is also worth mentioning quite unique setups detecting the TO effect under overall dc rotation [18]. Some observed features, however, (such as low- T dissipation) are still controversial and require further experimental investigation.
There are numerous difficulties with the standard supersolid interpretation of the period decrease. The most striking ones are as follows:
(i) The NCRI fraction (NCRIF) is very sensitive to the TO cell design: the NCRIF of ∼1% [5] originally detected in a composite cell was found either to drop by ∼100 times in a much more rigid cell [19] or increase by a factor of 20 in a narrow annulus space [20]. Polycrystalline samples grown by the blocked capillary technique may have both larger and smaller NCRIF than samples grown at constant temperature from the superfluid, not to mention large annealing effects [20].
(ii) The potential flow tests (verifying that NCRIF is sensitive to changes in the topology of the flow pattern) remain controversial [21].
(iii) There is no clear evidence of a phase transition yet. Typically, it is a crossover taking place within the temperature interval 0.1K<T<0.25K, which is barely changing—despite enormous variations in the NCRIF by several orders of magnitude (!)—from one TO setup to the next. This is not expected even in strongly disordered 3D superfluids.
(iv) An enigmatic feature, which is impossible to reconcile with the uniform condensation of vacancies in a 3D sample, is a dissipation peak centered at the temperature of the strongest variation of NCRIF [5]. This peak shows up as a dip in the amplitude of the resonant oscillations vs temperature.
(v) The NCRI suppression, occurring when the rim velocity v oscillates at an amplitude as small as ν∼10– 100μ/s, cannot be accounted for by any known mechanism of lowering the superfluid critical velocity from a typical value of a few m/s. More importantly, the effect of velocity does not seem to be of a sharp threshold character, with a pronounced critical value νc. Rather, it is found to be equivalent, in a certain sense, to temperature [22]: the NCRIF depends on a single variable ξ∼(T/T*)ξ+(ν/ν*)λ, with T*, ν*, ξ>0, λ>0, λ/ξ≈0.43 being some parameters. Such collapse of the data into a single master curve, in particular, implies that vc must be an increasing function of temperature: νc∼Tξ/λ , with ξ/λ≈2.3. However, this feature has been recently questioned by Kojima whose data suggests that νc first significantly decreases with T and then saturates to a constant value [23].
(vi) At low temperature Th<50– 70mK, the NCRIF data demonstrate hysteretic behavior as a function of velocity [24]. To some extent, a summary of this feature and of the previous one can be presented in the form of a “phase diagram” in the ( ν , T ) plane [25].
(vii) Extremely small concentrations of 3He impurities (as small as 10ppb– 1ppm) strongly affect the onset temperature and the NCRIF amplitude [26].
The phenomena described above (and also the results of first-principles simulations discussed below) unambiguously indicate that NCRI is induced by structural disorder. Nevertheless, a consistent microscopic picture behind the experimental findings is still missing. Being the hallmark signature of the superfluid behavior, sensitivity to the sample topology has to be settled beyond reasonable doubt in future experiments. The current research trend is to design new experiments to attack the problem from different angles; on the theoretical side, the challenge is to understand the mechanisms leading to the observed striking phenomena. Below we review some of the new and existing directions.
A strong indication of structural disorder comes from NMR experiments [27] where a large fraction of 3He impurities (about 100ppm) is trapped by some lattice defects. If these defects are dislocations, then their density is at least 4 orders of magnitude larger than the traditionally accepted values in the bulk. Most recent NMR measurements [28] at low 3He concentrations have found a peak in T1 relaxation time (a measure of how fast the magnetization along the applied magnetic field relaxes to its equilibrium value) at temperatures where the TO anomaly, specific heat anomaly [17], and the maximum of dissipation are observed [5]. This peak [28] might be an indication of some phase transformation occurring within the defect structure.
An unexpected discovery by Day and Beamish [29] was that the shear modulus μ(T) of hcp 4He hardens below T0∼0.15K at frequencies 2– 2000Hz—exactly in the range of the TO anomaly. Such a hardening in solid 4He was observed much earlier by Tsymbalenko [30] at significantly larger temperatures and frequencies. At the moment it is not clear if these two anomalies are due to one and the same mechanism. Originally, the low-temperature hardening effect was observed by Friedel [31] in pure aluminum more than 50 years ago. Amazingly, many properties of μ(T) and NCRIF regarding temperature and rim velocity dependencies, the responses to 3He impurities, or the hysteretic behavior [24,29], are similar, if not identical, to each other. The hardening of 4He can be related to pinning of dislocations by 3He impurities [29] as well as to self-trapping [32] in the periodic potential provided by the surrounding crystalline lattice—the so-called Peierls potential. Within the linear response regime, the shear modulus increase leads to the TO period decrease at frequencies well below acoustic resonances; i.e., there is a possibility for a purely “classical” interpretation of the TO anomaly. This explanation, however, does not pass the quantitative test: numerical evaluation of the TO period shift due to a 20% change in μ shows that it is barely a few percent of the observed effect [33]. The other difficulty is that similar period shifts, including hysteresis, small critical velocities, and the effect of small concentrations of 3He are observed in porous materials (Vycor [5], porous gold [34] and Gelsil [35]) where dislocations, whatever they are in such highly disordered medium, should be strongly pinned by pore walls. Finally, critical stresses for suppression of the shear modulus effect are almost 2 orders of magnitude larger than stresses generated by ac velocities ∼10μm/s influencing the NCRI effect [36].
The “ordinary glass” approach [37] involves proposing a phenomenological model for the generalized response function to describe period shifts using several fitting parameters and functions. The goal of this phenomenology is to demonstrate that the experimentally observed response is consistent with zero superfluid fraction and thus can be of nonsuperfluid origin. This approach, however, does not explain the microscopic origins of the “ordinary glassiness,” which is known not to exist (at least, on the classical level) in solids made of simple atomic elements, nor does it explain the physics behind model parameters and their temperature dependencies. In this sense the ordinary glass framework is as enigmatic as the supersolid interpretation itself.
Recent work by Reppy [38] posed serious experimental objections to the idea of a superfluid origin of the period shift. In this experiment, the change of the period of oscillation—as a function of deliberately introduced disorder—was observed at high ( ∼1K), not low, temperatures, while in the T→0 limit the oscillation periods of samples with different amounts of disorder were approaching one and the same value. Similar behavior was observed upon annealing—period changes occurred at high T. Clearly, experiments of the kind carried out by Reppy (or at least the annealing part) should be repeated by other groups to settle the issue. The most recent results on the triple-frequency TO [39] have clearly demonstrated that the NCRIF can be extremely sensitive to the mechanical properties depending on the TO design. The possibility of the nonsuperfluid origin of the NCRI was also reported by Eyal et al. [40], where a strong NCRI effect has been seen at temperatures as high as 1.3-1.9K—well above the 0.2K anomaly [5].
Given the rather unknown character of structural disorder in solid 4He, it is desirable to study behavior of almost ideal crystals. Working in this direction, the group at École Normale Supérieure in Paris is now growing essentially monocrystalline 4He samples, free from 3He impurities, and is performing ultrasound [41] and TO experiments on them [42]. One of the striking effects found recently is the strong low- T shear modulus softening effect [41]—just the opposite to the observation of Day and Beamish. Such softening is metastable—it disappears upon the thermal cycling. The nature of this effect requires further study.
Observation of direct superflow through 4He
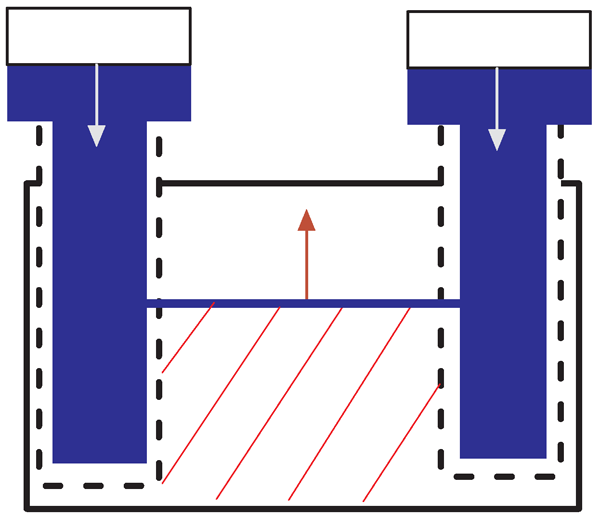
A unique experimental setup for studying the dc flow response of solid helium was created by Ray and Hallock [43]. In these experiments, atoms of 4He can be injected into solid 4He from the superfluid reservoirs through two Vycor rods. A schematic of the setup is shown in Fig. 3, the so-called syringe regime, when both electrodes are utilized for injecting liquid helium into the solid. In flow experiments one looks at the system response when pressure/temperature is changed in one reservoir by monitoring pressure/temperature in the second reservoir. The syringe regime is used for studying the so-called isochoric compressibility, that is, the variation of the density of atoms—injected one-by-one into a constant-volume solid phase—in response to varying the outside chemical potential. Such compressibility has been found to be comparable to that of liquid 4He, despite the fact that in an ideal solid it is essentially zero. As suggested by Söyler et al. [44], crystal growth is possible by the mechanism of dislocation superclimb—edge dislocations add atoms to atomic layers provided there is superfluid atomic transport along their cores (see Fig. 3). It is important that the giant isochoric compressibility regime has always been observed together with the superflow through the solid. Furthermore, while the solid may contain frozen-in pressure gradients of about 0.1 bar, the accumulation of matter under the injection occurs in a uniform manner—the pressure rises in unison at the opposite ends of the sample. This feature is clearly inconsistent with any type of classical plastic behavior, which results in time-dependent pressure gradients. At the same time, the picture of a percolating network of dislocations with superfluid cores delivering matter independently of the pressure gradients is consistent with such an observation.
Another important piece of evidence for superfluidity in the experiment [43] is the observed regime of supercritical flow—linear dependence on time of the pressure difference between the two superfluid reservoirs. The value of the onset temperature T≈0.5K is about 4 times smaller than the λ temperature of liquid 4He. This excludes the interpretation that the flow proceeds along stable mesoscopic liquid channels, which are known to exist in polycrystalline samples at the melting curve [45]. The strongest evidence against the liquid-channel picture is a striking and dramatic drop of the critical dc flux taking place at temperatures around 70mK, with a surprising subsequent revival at temperatures around 60mK [40]. This phenomenon appears to be the key to understanding properties of superfluid structural defects in solid He, and definitely deserves more attention.
Given the extremely controversial character of the TO data in terms of their superfluid interpretation, the natural question to ask is whether phenomena revealed by Ray and Hallock have anything to do with the TO behavior. Formally, the results by Ray and Hallock do imply that there should be some mass decoupling corresponding to the superfluid fraction. However, the estimates of the superfluid fraction they observed [43] show that it is too small to be resolved in a TO experiment. An intriguing connection might be through yet-to-be-understood quantum physics of structural defects.
Theoretical understanding of solid 4He
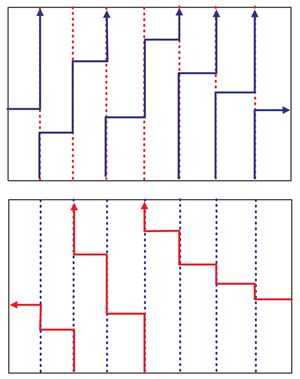
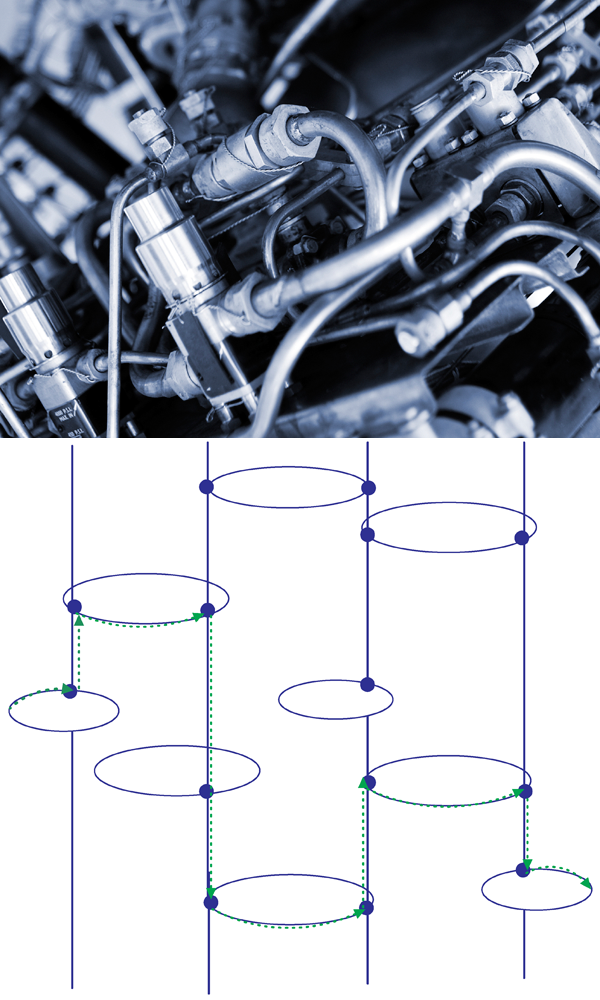
Theorists have also been hard at work shedding light on what is and what is not possible in bosonic continuous-space solids with the typical interparticle interaction U(r), and in particular, in the hcp crystal of 4He. Let us consider an interaction potential that does not favor vacancies or interstitials in the classical, not quantum, crystal at zero T (in fact, we are not aware of any counterexample) and ask whether quantum mechanics can change this situation and induce zero-point vacancies or interstitials (to become superfluid according to the Andreev-Lifshitz scenario [2]). Remember that Feynman’s path integral representation of the equilibrium statistics of bosons in d dimensions establishes an exact mapping onto statistics of classical (d+1)-dimensional “polymers” (particle world lines). With the celebrated Pollock and Ceperley formula this yields an explicit relation between the variation of polymer windings and superfluid response. Thus supersolidity can be pictured (see Fig. 4) as a disordered state of world lines of vacancies (interstitials) among relatively straight and ordered world lines of the rest of the bosons.
Within the path integral representation one can perform first-principles simulations of systems consisting of thousands of 4He atoms. This representation is also a powerful tool for general qualitative analysis. Using the language of path integrals, Prokof’ev and Svistunov have proven a theorem (see Ref. [26] in Ref. [46]) that no supersolid is possible without such zero-point defects. The crucial control parameter ξins=mUR20/h2, where m denotes the particle mass, and U and R0 are the characteristic values of the interaction potential and interparticle distance, respectively. This parameter controls the ratio between the potential and zero-point kinetic energy terms for vacancy/interstitial world lines. Large ξins ensures that no zero-point vacancies (or interstitials) can be induced. In other words, for large ξins the crystal adjusts its volume so that no zero-point defects—either vacancies or interstitials—are present in the ground state. Furthermore, energy gaps for their creation in pairs are large (cf. the discussion presented by Andreev and Lifschitz in Ref. [2]), and thus the ground state is insulating according to the above mentioned theorem.
Reliable first principles Monte Carlo simulations allow us to explore basic properties of ideal hcp crystals, as well as point and extended defects embedded in it. These simulations have demonstrated that a perfect hcp crystal of 4He is an insulator with the gap of about 13K for vacancies and 23K for interstitials (see Refs. [32,33] in Ref. [46]). Furthermore, it has been numerically observed that injection of vacancies into a perfect sample leads to their clustering (see Ref. [38] in Ref. [46]) into Frank’s dislocation loops (see Ref. [31]) in the basal plane (or phase separation at the melting curve). These results invalidate the Andreev-Lifshitz-Thouless-Chester (ALTC) proposal.
Having argued against the ALTC supersolid, we stress that the hcp solid 4He is likely to support supersolidity induced by a network of superfluid structural topological defects. Ab initio Monte Carlo simulations have revealed that some grain boundaries [47] and dislocations [48,44] support superflow. More than two decades ago, Shevchenko predicted very unusual dynamical properties of a network of superfluid dislocations [49]—large temperature range where at extremely low frequencies the behavior is normal, while at frequencies relevant to the experiment the response is superfluid. In the macroscopic limit, this microscopic model realizes a state filled with long-lived circulating persistent currents (cf. the “vortex fluid” model of Anderson [50] and the interpretation of the TO data in terms of such a fluid by Penzev et al. [51]). The normal response of the Shevchenko state is controlled by the rate of phase-slip events, which are processes by which the superflow dissipates its energy. As a result, the flow can be characterized by frequency-dependent viscosity (significantly suppressed at times shorter than a typical phase-slip time). The thermodynamic superfluid transition sets in at a temperature Tc≈T*/L, where L stands for a typical length of free dislocation segments in units of its width (of the order of the lattice spacing) and T*∼1K is the temperature of the superfluid transition in liquid 4He. Thus, if the specific heat anomaly around 50– 100mK [17] is due to a thermodynamic transition into the superfluid state of the dislocation network, a typical length of the dislocation segments must be only 50– 100 angstroms, which corresponds to an extremely large density of dislocations—in the range 1011– 1013cm-2—that may require special growth conditions, with additional deformations produced akin to the setup [52]. By the same token, for typical densities of dislocations 106– 109cm-2, the true thermodynamic transition may never be observed unless temperatures are lowered below 0.1mK. It is important to note that, while Tc is strongly dependent on dislocation density, the onset temperature of superfluid-type behavior, T0, is determined by local phase-slip rates and, therefore, is independent of L.
There are strong reasons to believe that the effect of anomalous isochoric compressibility observed by Ray and Hallock points to the dislocation network scenario of supersolidity. Söyler et al. [44] argued that the natural framework for the giant isochoric compressibility is the climb of (rough) edge dislocations supported by the superfluidity of their cores as it requires mass transport in the crystal. Most recently, it was noted [53] that the dip in the flow rate [54] may be due to the dislocation roughening induced by the frozen internal stresses. Superclimb is one of the qualitatively new effects in the emerging subfield of metallurgical behavior induced by quantum phenomena—“quantum metallurgy,” as called by A. Dorsey. It provides strong evidence that supersolidity of structural defects and metallurgical behavior of solid 4He are closely interconnected and the setup of Ray and Hallock [43] is highly promising for studying this new physics.
Solid 4He may also exist in the metastable superglass state observed in Monte Carlo simulations of quenched liquid 4He (see Ref. [32] in Ref. [46]). This state is characterized by the absence of any long-range structural order and superfluid fractions as large as ten percent. It is, however, unlikely that this phase can form under the experimental conditions of relatively slow cooling in TO. A recent experiment [52] challenges this view and suggests that bosonic 4He, as opposed to fermionic 3He, can form stable glass or dense dislocation network at low T. It has been observed that inducing structural disorder by external stress in solid 4He leads to the ∼T2 contribution to pressure. No such effect has been seen in samples of solid 3He. This contribution is consistent with linear specific heat due to two-level systems in glasses and/or dislocations.
New challenges
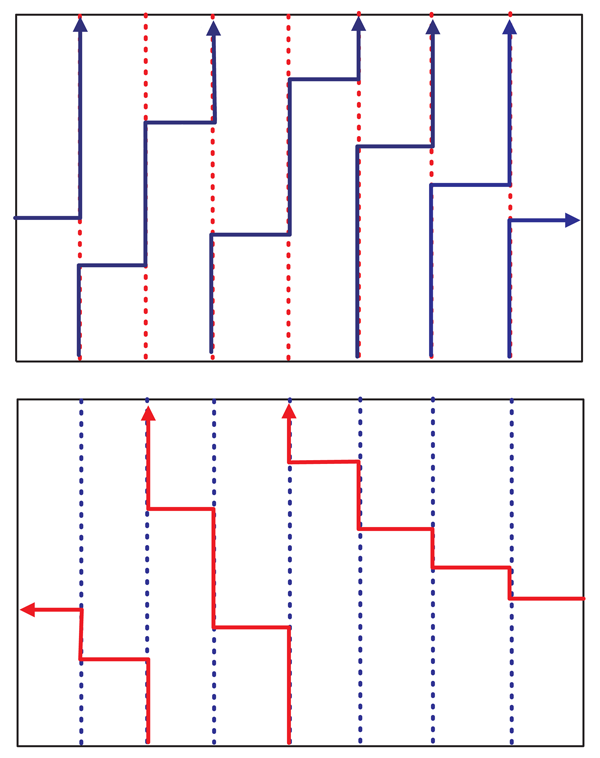
Clearly, more measurements are needed. In our opinion, in order of priority, it is crucial to measure the isochoric compressibility χ(T,ω) and the critical superflow rate R(T,ω) as functions of temperature T, frequency ω, and 3He concentration. The superclimb effect is expected to be suppressed below a temperature Tr, determining dislocation roughening [44]. Such suppression must lead to reduced χ(T,ω) and enhanced R(T,ω). We note that R(T,ω) and χ(T,ω) are sensitive to the nature of the Shevchenko state, that is, to the phase-slip rate. Such dependencies will provide valuable information for constructing theoretical models of the network where purely superfluid dynamics is coupled to climbing and gliding motion of structural defects. One possible structure of such dynamical network is shown in Fig. 5. Another important aspect is understanding the nature of the dip in the flow rate at low temperature [54] and, in particular, its shape and position as a function of 3He concentration (see the proposal in Ref. [53]). The “smoking gun” evidence for supersolid behavior would be the observation of the persistent currents.
The Ray and Hallock setup can shed light on the fundamental question: Why was there no flow observed upon applying mechanical pressure to the solid in a number of experiments [13,14]? In this regard we note that the syringe effect [43] immediately implies the possibility of reversing it, i.e., creating a flow of matter from the solid to a reservoir (via a super link) upon applying pressure to the solid. To what extent the very large aspect ratio (length/width ∼100– 400) of the capillaries and, possibly, the crystal growth conditions in the setups [13,14] are relevant to the answer remains to be seen. Interestingly, Ray and Hallock have observed that the syringe effect does not take place if the crystal is grown by injecting atoms into the superfluid at sufficiently low temperature [43].
The “conductance through solid” measurements can be helpful in resolving yet another puzzle, namely, the close similarity between all TO features observed in crystalline 4He and solid 4He confined in Vycor [5] and other nanopore materials [34]. The key question is whether or not there are differences in R(T,ω) and χ(T,ω) in both media. At this point it is instructive to recall the history of discovering linear topological defects in solids—the dislocations and disclinations. This discovery was made by Vito Volterra long before the crystalline structure of solids had been seen [55]. In fact, the only requirement for having a dislocation characterized by typical long-range stresses around the core is that a medium can sustain shear stresses up to some threshold. Thus, while crystallinity introduces exact quantization of the Burgers vector and related topological protection of the defect, such exactness is not required for characterizing dislocations as linear concentrators of stresses. Dislocations introduced at this level of description could explain the similarity between TO responses of crystalline 4He and strongly disordered solid 4He in Vycor.
References
- E. P. Gross, Phys. Rev. 106, 161 (1957); Ann. Phys. 4, 57 (1958)
- A. F. Andreev and I. M. Lifshitz, Sov. Phys. JETP 29, 1107 (1969)
- D. J. Thouless, Ann. Phys. 52, 403 (1969)
- G. V. Chester, Phys. Rev. A 2, 256 (1970)
- E. Kim and M. H. W. Chan, Nature 427, 225 (2004); Science 305, 1941 (2004)
- G. G. Batrouni and R. T. Scalettar, Phys. Rev. Lett. 84, 1599 (2000); F. Hébert, G. G. Batrouni, R. T. Scalettar, G. Schmid, M. Troyer, and A. Dorneich, Phys. Rev. B 65, 014513 (2001); S. Wessel and M. Troyer, Phys. Rev. Lett. 95, 127205 (2005); R. G. Melko, A. Paramekanti, A. A. Burkov, A. Vishwanath, D. N. Sheng, and L. Balents, 95, 127207 (2005); L. Pollet, J. D. Picon, H. P. Büchler, and M. Troyer, 104, 125302 (2010)
- T. Ohgoe, T. Suzuki, and N. Kawashima, arXiv:1110.5261
- Y. Pomeau and S. Rica, Phys. Rev. Lett. 72, 2426 (1994); L. Santos, G. V. Shlyapnikov, and M. Lewenstein, 90, 250403 (2003); M. Vengalattore, J. Guzman, S. R. Leslie, F. Serwane, and D. M. Stamper-Kurn, Phys. Rev. A 81, 053612 (2010); N. Henkel, R. Nath, and T. Pohl, Phys. Rev. Lett. 104, 195302 (2010); X. Li, W. V. Liu, and C. Lin, arXiv:1005.4027; C. Josserand, Y. Pomeau, and S. Rica, Phys. Rev. Lett. 98, 195301 (2007)
- F. Cinti, P. Jain, M. Boninsegni, A. Micheli, P. Zoller, and G. Pupillo, Phys. Rev. Lett. 105, 135301 (2010); S. Saccani, S. Moroni, and M. Boninsegni, Phys. Rev. B 83, 092506 (2011)
- L. Pollet (private communication)
- I. Danshita, and C. A. R. Sá de Melo, Phys. Rev. Lett. 103, 225301 (2009)
- B. Spivak and S. A. Kivelson, Phys. Rev. B 70, 155114 (2004); B. Spivak (private communication)
- D. J. Bishop, M. A. Paalanen, and J. D. Reppy, Phys. Rev. B 24, 2844 (1981); D. S. Greywall, 16, 1291 (1977)
- J. Day, T. Herman, and J. Beamish, Phys. Rev. Lett. 95, 035301 (2005); J. Day and J. Beamish, 96, 105304 (2006); A. S. C. Rittner, W. Choi, E. J. Mueller, and J. D. Reppy, Phys. Rev. B 80, 224516 (2009)
- A. J. Leggett, Phys. Rev. Lett. 25, 1543 (1970)
- J. Goodkind, Phys. Rev. Lett. 89, 095301 (2002)
- X. Lin, A. C. Clark, Z. G. Cheng, and M. H. W. Chan, Phys. Rev. Lett. 102, 125302 (2009); 103, 259903 (2009)
- M. Yagi, A. Kitamura, N. Shimizu, Y. Yasuta, and M. Kubota, J. Low Temp. Phys. 162, 754 (2011); 162, 492 (2011); H. Choi, D. Takahashi, K. Kono, and E. Kim, Science 330, 1512 (2010)
- J. West and M. Chan, talk at Supersolids 2009, Banff, http://www.phys.ualberta.ca/supersolids/talks/WestChan-talk.pdf; D. Kim, talk at LT26, Beijing, August 12, 2011, http://lt26.iphy.ac.cn/abstract/pdf/A0458.pdf
- A. S. Rittner and J. D. Reppy, Phys. Rev. Lett. 97, 165301 (2006); 98, 175302 (2007); 101, 155301 (2008)
- J. Reppy, A. S. C.Rittner, W. Choi, and E. Kim, talk at Supersolids 2009, Banff, http://www.phys.ualberta.ca/supersolids/talks/REPPY-talk.pdf.
- E. Pratt, B. Hunt, V. Gadagkar, M. Yamashita, A. Balatsky, and J. C. S. Davis, Science 332, 821 (2011)
- H. Kojima (private communication)
- Y. Aoki, J. C. Graves, and H. Kojima, Phys. Rev. Lett. 99, 015301 (2007)
- H. Choi, S. Kwon, D.Y. Kim, and E. Kim, Nature Physics 6, 424 (2010)
- E. Kim, J. S. Xia, J. T. West, X. Lin, A. C. Clark, and M. H. W. Chan, Phys. Rev. Lett. 100, 065301 (2008)
- R. Toda, P. Gumann, K. Kosaka, M. Kanemoto, W. Onoe, and Y. Sasaki, Phys. Rev. B 81, 214515 (2010)
- S. S. Kim, C. Huan, L. Yin, J. Xia, D. Candela, and N. S. Sullivan, Phys. Rev. Lett. 106, 185303 (2011)
- J. Day and J. Beamish, Nature 450, 853 (2007); J. Day, O. Syshchenko, and J. Beamish, Phys. Rev. B 79, 214524 (2009)
- V. L. Tsymbalenko, Sov. Phys. JETP 60, 537 (1984)
- J. Friedel, Dislocations (Pergamon Press, New York, 1964)
- D. Aleinikava, E. Dedits, A. B. Kuklov, and D. Schmeltzer, Europhys. Lett. 89, 46002 (2010)
- A. C. Clark, J. D. Maynard, and M. H. W. Chan, Phys. Rev. B 77, 184513 (2008)
- D. Y. Kim, S. Kwon, H. Choi, H. C. Kim, and E. Kim, New J. Phys. 12, 033004 (2010)
- K. Shirahama (private communication)
- J. Day, O. Syshchenko, and J. Beamish, Phys. Rev. Lett. 104, 075302 (2010)
- Z. Nussinov, A. V. Balatsky, M. J. Graf, and S. A. Trugman, Phys. Rev. B 76, 014530 (2007); A. F. Andreev, JETP 108, 1157 (2009); arXiv:0809.1990; S. E. Korshunov, JETP Lett. 90, 156 (2009); arXiv:0906.4627
- J. Reppy, Phys. Rev. Lett. 104, 255301 (2010)
- J. Reppy, LT26, 2011, Beijing, http://lt26.iphy.ac.cn/abstract/pdf/A1389.pdf.; X. Mi, E. Mueller, and J. D. Reppy, arXiv:1109.6818
- A. Eyal, O. Pelleg, L. Embon, and E. Polturak, Phys. Rev. Lett. 105, 025301 (2010)
- X. Rojas, A. Haziot, V. Bapst, and S. Balibar, Phys. Rev. Lett. 105, 145302 (2010)
- S. Balibar (private communication)
- M. W. Ray and R. B. Hallock, Phys. Rev. Lett. 100, 235301 (2008); Phys. Rev. B 79, 224302 (2009)
- Ş. G. Söyler, A. B. Kuklov, L. Pollet, N. V. Prokof’ev, and B. V. Svistunov, Phys. Rev. Lett. 103, 175301 (2009)
- S. Sasaki, R. Ishiguro, F. Caupin, H. J. Maris, and S. Balibar, Science 313, 1098 (2006); S. Balibar and F. Caupin, J. Phys. Condens. Matter 20, 173201 (2008)
- N. V. Prokof’ev, Adv. Phys. 56, 381 (2007)
- L. Pollet, M. Boninsegni, A. B. Kuklov, N. V. Prokof ’ev, B. V. Svistunov, and M. Troyer, Phys. Rev. Lett. 98, 135301 (2007)
- M. Boninsegni, A. B. Kuklov, L. Pollet, N. V. Prokof ’ev, B. V. Svistunov, and M. Troyer, Phys. Rev. Lett. 99, 035301 (2007)
- S. I. Shevchenko, Sov. J. Low Temp. Phys. 13, 61 (1987); D. V. Fil and S. I. Shevchenko, Phys. Rev. B 80, 100501 (2009)
- P. W. Anderson, Nature Phys. 3, 160 (2007)
- A. Penzev, Y. Yasuta, and M. Kubota, Phys. Rev. Lett. 101, 065301 (2008)
- A. A. Lisunov, V. A. Maidanov, V. Yu. Rubanskiy, S. P. Rubets, E. Ya. Rudavskii, A. S. Rybalko, and V. A. Tikhii, Phys. Rev. B 83, 132201 (2011)
- D. Aleinikava and A. B. Kuklov, Phys. Rev. Lett. 106, 235302 (2011); arXiv:1108.1182
- M. W. Ray and R. B. Hallock, Phys. Rev. Lett. 105, 145301 (2010)
- V. Volterra, Ann. Sci. Ec. Norm. Super. Paris 24, 401 (1907)